| Citation: |
Jiazhe Lin, Rui Xu, Xiaohong Tian. PATTERN FORMATION IN REACTION-DIFFUSION NEURAL NETWORKS WITH LEAKAGE DELAY[J]. Journal of Applied Analysis & Computation, 2019, 9(6): 2224-2244. doi: 10.11948/20190001
|
PATTERN FORMATION IN REACTION-DIFFUSION NEURAL NETWORKS WITH LEAKAGE DELAY
-
Abstract
Due to the heterogeneity of the electromagnetic field in neural networks, the diffusion phenomenon of electrons exists inevitably. In this paper, we investigate pattern formation in a reaction-diffusion neural network with leakage delay. The existence of Hopf bifurcation, as well as the necessary and sufficient conditions for Turing instability, are studied by analyzing the corresponding characteristic equation. Based on the multiple-scale analysis, amplitude equations of the model are derived, which determine the selection and competition of Turing patterns. Numerical simulations are carried out to show the possible patterns and how these patterns evolve. In some cases, the stability performance of Turing patterns is weakened by leakage delay and synaptic transmission delay.-
Keywords:
- Pattern formation /
- reaction-diffusion /
- leakage delay /
- neural network /
- amplitude equation
-
-
References
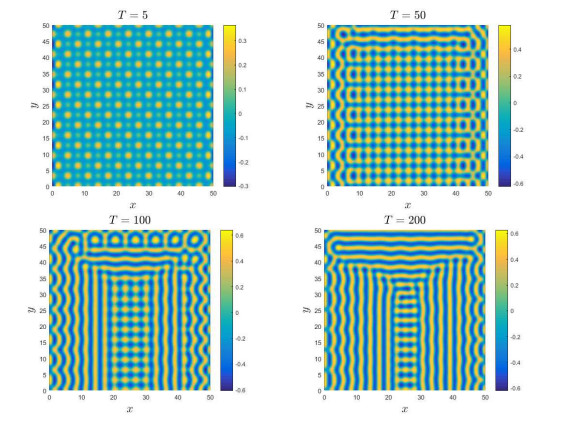
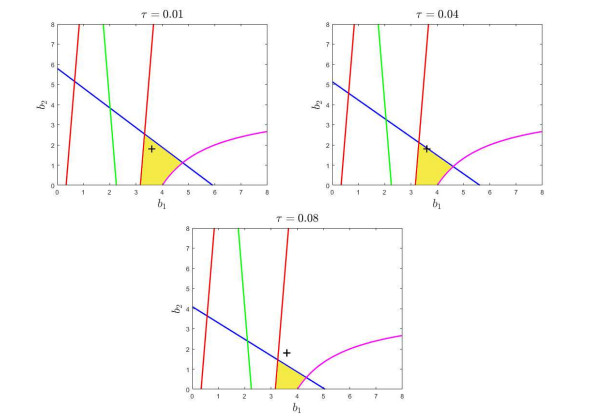
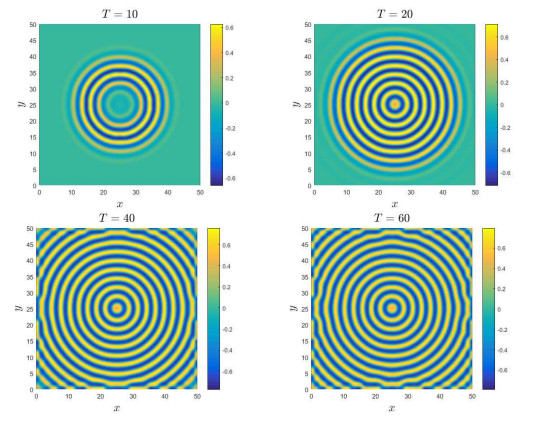
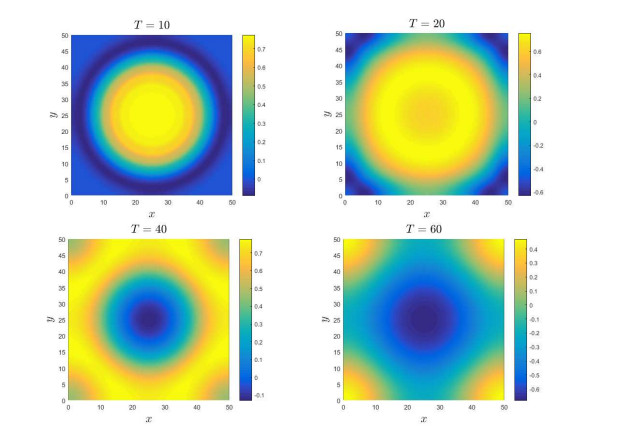
[1] G. Bao and Z. Zeng, Analysis and design of associative memories based on recurrent neural network with discontinuous activation functions, Neurocomputing, 2012, 77(1), 101-107. doi: 10.1016/j.neucom.2011.08.026 [2] L. O. Chua and L. Goraş, Turing patterns in cellular neural networks, International Journal of Electronics, 1995, 79(6), 719-736. doi: 10.1080/00207219508926307 [3] T. Deb, A. K. Ghosh and A. Mukherjee, Singular value decomposition applied to associative memory of Hopfield neural network, Materials Today: Proceedings, 2018, 5(1), 2222-2228. doi: 10.1016/j.matpr.2017.09.222 [4] K. Gopalsamy, Leakage delays in BAM, Journal of Mathathematical Analysis and Applications, 2007, 325(2), 1117-1132. doi: 10.1016/j.jmaa.2006.02.039 [5] K. Gopalsamy, Stability and oscillations in delay differential equations of population dynamics, Kluwer Academic, Dordrecht, 1992. [6] M. Garvie, Finite difference schemes for reaction-diffusion equations modeling predator-prey interactions in MATLAB, Bulletin of Mathematical Biology, 2007, 69(3), 931-956. doi: 10.1007/s11538-006-9062-3 [7] B. Han and Z. Wang, Turing patterns of a Lotka-Volterra competitive system with nonlocal delay, International Journal of Bifurcation and Chaos, 2018, 28(7), 1830021. doi: 10.1142/S0218127418300215 [8] C. Huang, L. Huang, J. Feng, M. Nai and Y. He, Hopf bifurcation analysis for a two-neuron network with four delays, Chaos, Solitons and Fractals, 2007, 34, 795-812. doi: 10.1016/j.chaos.2006.03.089 [9] C. Huang, Y. He, L. Huang and Z. Yuan, Hopf bifurcation analysis of two neurons with three delays, Nonlinear Analysis: Real World Applications, 2007, 8(3), 903-921. doi: 10.1016/j.nonrwa.2006.03.014 [10] C. Huang, Y. Meng, J. Cao, A. Alsaedi and F. Alsaadi, New bifurcation results for fractional BAM neural network with leakage delay, Chaos, Solitons and Fractals, 2017, 100, 31-44. doi: 10.1016/j.chaos.2017.04.037 [11] C. Huang and J. Cao, Impact of leakage delay on bifurcation in high-order fractional BAM neural networks, Neural Networks, 2018, 98, 223-235. doi: 10.1016/j.neunet.2017.11.020 [12] B. Kosko, Neural Networks and Fuzzy Systems, Prentice Hall, New Delhi, 1992. [13] Z. Lin, D. Ma, J. Meng and L. Chen, Relative ordering learning in spiking neural network for pattern recognition, Neurocomputing, 2018, 275, 94-106. doi: 10.1016/j.neucom.2017.05.009 [14] P. Melin and D. Sánchez, Multi-objective optimization for modular granular neural networks applied to pattern recognition, Information Sciences, 2017, 460-461, 594-610. [15] T. Mikołajczyk, K. Nowicki, A. Bustillo and D.Y. Pimenov, Predicting tool life in turning operations using neural networks and image processing, Mechanical Systems and Signal Processing, 2018, 104, 503-513. doi: 10.1016/j.ymssp.2017.11.022 [16] L. Olien and J. Bélair, Bifurcations, stability, and monotonicity properties of a delayed neural network model, Physica D, 1997, 102(3-4), 349-363. doi: 10.1016/S0167-2789(96)00215-1 [17] Q. Ouyang, Patterns Formation in Reaction-diffusion Systems, Shanghai Science and Technology Education Press, Shanghai, 2000. [18] R. Sakthivel, P. Vadivel, K. Mathiyalagan, A. Arunkumar and M. Sivachitra, Design of state estimator for bidirectional associative memory neural networks with leakage delays, Information Sciences, 2015, 296, 263-274. doi: 10.1016/j.ins.2014.10.063 [19] Y. Seo and K. Shin, Hierarchical convolutional neural networks for fashion image classification, Expert Systems with Applications, 2019, 116, 328-339. doi: 10.1016/j.eswa.2018.09.022 [20] X. Tian and R. Xu, Hopf bifurcation analysis of a reaction-diffusion neural network with time delay in leakage terms and distributed delays, Neural Processing Letters, 2016, 43(1), 173-193. doi: 10.1007/s11063-015-9410-0 [21] X. Tian, R. Xu and Q. Gan, Hopf bifurcation analysis of a BAM neural network with multiple time delays and diffusion, Applied Mathematics and Computation, 2015, 266, 909-926. doi: 10.1016/j.amc.2015.06.009 [22] A.M. Turing, The chemical basis of morphogenesis, Philosophical Transactions of the Royal Society B, 1952, 237(1-2), 37-72. [23] S. Tyagi, S. Jain, S. Abbas, S. Meherrem and R. Ray, Time-delay-induced instabilities and Hopf bifurcation analysis in 2-neuron network model with reaction-diffusion term, Neurocomputing, 2018, 313, 306-315. doi: 10.1016/j.neucom.2018.06.008 [24] Y. Wang, J. Cao, G. Sun and J. Li, Effect of time delay on pattern dynamics in a spatial epidemic model, Physica A, 2014, 412, 137-148. doi: 10.1016/j.physa.2014.06.038 [25] Z. Wu, J. Li and J. Li, Pattern formations of an epidemic model with Allee effect and time delay, Chaos, Solitons and Fractals, 2017, 104, 599-606. doi: 10.1016/j.chaos.2017.09.028 [26] J. Yang, L. Wang, Y. Wang and T. Guo, A novel memristive Hopfield neural network with application in associative memory, Neurocomputing, 2017, 227, 142-148. doi: 10.1016/j.neucom.2016.07.065 [27] H. Yin and X. Wen, Pattern formation through temporal fractional derivatives, Scientific Reports, 2018, 8(1), 5070. doi: 10.1038/s41598-018-23470-8 [28] H. Zhao, X. Huang and X. Zhang, Turing instability and pattern formation of neural networks with reaction-diffusion terms, Nonlinear Dynamics, 2014, 76, 115-124. doi: 10.1007/s11071-013-1114-2 [29] X. Zhang, G. Sun and Z. Jin, Spatial dynamics in a predator-prey model with Beddington-DeAngelis functional response, Physical Review E, 2012, 85(2), 021924. doi: 10.1103/PhysRevE.85.021924 [30] Q. Zheng and J. Shen, Dynamics and pattern formation in a cancer network with diffusion, Communications in Nonlinear Science and Numerical Simulation, 2015, 27(1-3), 93-109. doi: 10.1016/j.cnsns.2015.02.023 [31] Q. Zheng and J. Shen, Pattern formation in the FitzHugh-Nagumo model, Computers and Mathematics with Applications, 2015, 70(5), 1082-1097. doi: 10.1016/j.camwa.2015.06.031 -
-
Figures(7)
Export File
Citation
Jiazhe Lin, Rui Xu, Xiaohong Tian. PATTERN FORMATION IN REACTION-DIFFUSION NEURAL NETWORKS WITH LEAKAGE DELAY[J]. Journal of Applied Analysis & Computation, 2019, 9(6): 2224-2244. doi: 10.11948/20190001
Format
Content
DownLoad:
-
Figure 1. Phase diagrams of system (1.2) with
$ \tau = 0.02 < \tau_0 = 0.0759 $ (see left-hand figure) and$ \tau = 0.08 > \tau_0 = 0.0681 $ (see right-hand figure), where$ c_1 = 2 $ ,$ c_2 = 4 $ ,$ a_1 = -4 $ ,$ a_2 = 2 $ ,$ b_1 = 3.6 $ ,$ b_2 = 1.2 $ and$ f_i(\cdot), g_i(\cdot) $ $ (i = 1, 2) $ are chosen as$ \tanh(\cdot) $ . -
Figure 2. The bifurcation diagram of system (1.2) with respect to the delay
$ \tau\in[0, 0.15] $ . -
Figure 3. The trajectories of
$ h_1(\tau) $ ,$ h_2(\tau) $ ,$ h_3(\tau) $ and$ h_4(\tau) $ with respect to the delay$ \tau $ , where$ d_1 = 0.1 $ ,$ d_2 = 1.6 $ ,$ c_1 = 2 $ ,$ c_2 = 4 $ ,$ \phi_1 = -4 $ ,$ \phi_2 = 2 $ ,$ \varphi_1 = 3.6 $ ,$ \varphi_2 = 1.2 $ (Fig. 3(a)) and$ d_1 = 0.1 $ ,$ d_2 = 1.6 $ ,$ c1 = 2 $ ,$ c_2 = 4 $ ,$ \phi_1 = -4 $ ,$ \phi_2 = 2 $ ,$ \varphi_1 = 3.1 $ ,$ \varphi_2 = 1.2 $ (Fig. 3(b)).$ f_i(\cdot), g_i(\cdot) $ $ (i = 1, 2) $ are chosen as$ \tanh(\cdot) $ . -
Figure 4. Snapshots of contour pictures of the time evolution of
$ u(x, y, t) $ at time$ T = 5, 50, 100, 200 $ , respectively, with parameter values$ \tau = 0.01 $ ,$ d_1 = 0.1 $ ,$ d_2 = 1.6 $ ,$ c_1 = 2 $ ,$ c_2 = 4 $ ,$ a_1 = -4 $ ,$ a_2 = 2 $ $ b_1 = 3.4 $ ,$ b_2 = 1.2 $ and initial condition$ u_0 = v_0 = \sin(x)\cos(y)+\xi(x, y) $ in which$ \xi(x, y) $ is a stochastic process with respect to the spatial variable$ (x, y) $ . -
Figure 5. The bifurcation diagram of the parameters
$ b_1 $ and$ b_2 $ with$ \tau = 0.01 $ ,$ 0.04 $ ,$ 0.08 $ , respectively, where$ d_1 = 0.1 $ ,$ d_2 = 1.6 $ ,$ c_1 = 2 $ ,$ c_2 = 4 $ ,$ a_1 = -4 $ ,$ a_2 = 2 $ . The position of the `+' is$ (3.6, 1.8) $ . -
Figure 6. Snapshots of contour pictures of the time evolution of
$ u(x, y, t) $ at time$ T = 10, 20, 40, 60 $ , respectively, with parameter values$ \tau = 0.01 $ ,$ d_1 = 0.1 $ ,$ d_2 = 1.6 $ ,$ c_1 = 2 $ ,$ c_2 = 4 $ ,$ a_1 = -4 $ ,$ a_2 = 2 $ ,$ b_1 = 3.6 $ ,$ b_2 = 1.8 $ and initial condition$ u_0 = v_0 = (x-25)^2+(y-25)^2 < 100 $ . -
Figure 7. Snapshots of contour pictures of the time evolution of
$ u(x, y, t) $ at time$ T = 10, 20, 40, 60 $ , respectively, where$ \tau = 0.08 $ and other parameter values are similar to those in Fig. 6.