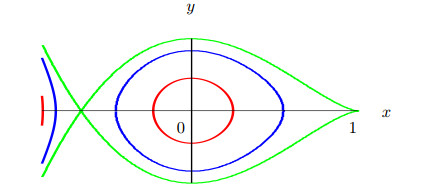
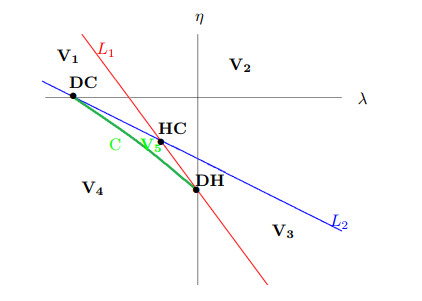
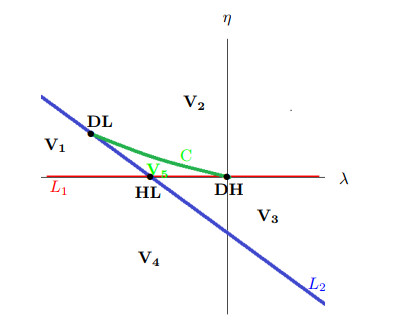
|
[1]
|
V. I. Arnold, Ten problems, Adv. Soviet Math., 1990, 1, 1-8.
Google Scholar
|
|
[2]
|
R. Asheghi and H. R. Zangeneh, Bifurcations of limit cycles from quintic Hamiltonian systems with an eye-figure loop, Nonlinear Anal. (TMA), 2008, 68, 2957-2976. doi: 10.1016/j.na.2007.02.039
CrossRef Google Scholar
|
|
[3]
|
R. Asheghi and H. R. Zangeneh, Bifurcations of limit cycles from quintic Hamiltonian systems with an eye-figure loop (Ⅱ), Nonlinear Anal. (TMA), 2008, 69, 4143-4162. doi: 10.1016/j.na.2007.10.054
CrossRef Google Scholar
|
|
[4]
|
R. Asheghi and H. R. Zangeneh, Bifurcations of limit cycles for a quintic Hamiltonian system with a double cuspidal loop, Comput. Math. Appl., 59(2010), 1409-1418. doi: 10.1016/j.camwa.2009.12.024
CrossRef Google Scholar
|
|
[5]
|
M. Cndido, J. Llibre and C. Valls, Non-existence, existence, and uniqueness of limit cycles for a generalization of the Van der PolDuffing and the Rayleigh-Duffing oscillators, Physica D: Nonlinear Phenomena, 2020, 132458.
Google Scholar
|
|
[6]
|
F. Dumortier and C. Li, Perturbations from an elliptic Hamiltonian of degree four: (Ⅰ) Saddle loop and two saddle cycle, J. Differ. Equat., 2001, 176, 114-157. doi: 10.1006/jdeq.2000.3977
CrossRef Google Scholar
|
|
[7]
|
F. Dumortier, R. Roussarie and J. Sotomayor, Generic 3-parameter families of vector fields on the plane, unfolding a singularity with nilpotent linear part. The cusp case of codimension 3, Ergodic theory and dynamical systems, 1987, 7, 375-413. doi: 10.1017/S0143385700004119
CrossRef Google Scholar
|
|
[8]
|
V. Gathen and J. Gerhard, Modern Computer Algebra, Cambridge University Press, 2013.
Google Scholar
|
|
[9]
|
M. Grau, F. Mañosas and J. Villadelprat, A Chebyshev criterion for Abelian integrals, Trans. Amer. Math. Soc., 2011, 363, 109-129. doi: 10.1090/S0002-9947-2010-05007-X
CrossRef Google Scholar
|
|
[10]
|
M. Gouveia, J. Llibre and L. A. Roberto, Phase portraits of the quadratic polynomial Liénarddifferential systems, Proc. Royal Soc. Edinb. A., 2020, 1-15.
Google Scholar
|
|
[11]
|
M. Han, Asymptotic expansions of Abelian integrals and limit cycle bifurcations, Int. J. Bifur. Chaos, 2012, 22, 1250296 (30 pages). doi: 10.1142/S0218127412502963
CrossRef Google Scholar
|
|
[12]
|
M. Han, J. Yang and D. Xiao, Limit cycle bifurcations near a double homoclinic loop with a nilpotent saddle, Int. J. Bifur. Chaos, 2012, 22, 1250189 (33 pages). doi: 10.1142/S0218127412501891
CrossRef Google Scholar
|
|
[13]
|
M. Han, J. Yang and P. Yu, Hopf bifurcations for near-Hamiltonian systems, Int. J. Bifur. Chaos, 2009, 19, 4117-4130. doi: 10.1142/S0218127409025250
CrossRef Google Scholar
|
|
[14]
|
M. Han and P. Yu, Normal Forms, Melnikov Functions and Bifurcations of Limit Cycles, Springer, 2012.
Google Scholar
|
|
[15]
|
M. Han, H. Zang and J. Yang, Limit cycle bifurcations by perturbing a cuspidal loop in a Hamiltonian system, J. Differ. Equat., 2009, 246, 129-163. doi: 10.1016/j.jde.2008.06.039
CrossRef Google Scholar
|
|
[16]
|
M. Han, J. Yang, A. A. Tarta and Y. Gao, Limit cycles near homoclinic and heteroclinic loops, J. Dynam. Differ. Equat., 2008, 20, 923-960. doi: 10.1007/s10884-008-9108-3
CrossRef Google Scholar
|
|
[17]
|
L. Hong, J. Lu and X. Hong, On the number of zeros of Abelian integrals for a class of quadratic reversible centers of genus one J. Nonlinear Modeling and Analysis, 2020, 2(2), 161-171.
Google Scholar
|
|
[18]
|
Y. A. Kuznetsov, Elements of Applied Bifurcation Theory (2nd Ed), Springer, New York, 1998.
Google Scholar
|
|
[19]
|
C. Li and C. Rousseau, A system with three limit cycles appearing in a Hopf bifurcation and dying in a homoclinic bifurcation: The cusp of order 4, J. Differ. Equat., 1989, 79, 132-167. doi: 10.1016/0022-0396(89)90117-4
CrossRef Google Scholar
|
|
[20]
|
C. Li and Z. Zhang, A criterion for determining the monotonicity of the ratio of two Abelian integrals, J. Differ. Equat., 1996, 124, 407-424. doi: 10.1006/jdeq.1996.0017
CrossRef Google Scholar
|
|
[21]
|
J. Llibre, A. C. Murza and C. Valls, On a conjecture on the integrability of Liénard systems, Rendiconti del Circolo Matematico di Palermo Series 2, 2020, 69, 209-216. doi: 10.1007/s12215-018-00398-6
CrossRef Google Scholar
|
|
[22]
|
F. Mañosas and J. Villadelprat, Bounding the number of zeros of certain Abelian integrals, J. Differ. Equat., 2011, 251, 1656-1669. doi: 10.1016/j.jde.2011.05.026
CrossRef Google Scholar
|
|
[23]
|
P. Mardesić, D. Novikov, L. Ortiz-Bobadilla and J. Pontigo-Herrera, Infinite orbit depth and length of Melnikov functions, Annales de l'Institut Henri Poincaré C, Analyse non linéaire, 2019, 36, 1941-1957. doi: 10.1016/j.anihpc.2019.07.003
CrossRef Google Scholar
|
|
[24]
|
D. Novikov and S. Malev, Linear Estimate for the Number of Zeros of Abelian Integrals, Qual. The. Dynam. Syst., 2017, 16, 689-696. doi: 10.1007/s12346-016-0213-0
CrossRef Google Scholar
|
|
[25]
|
L. Sheng, M. Han and Y. Tian, On the Number of Limit Cycles Bifurcating from a Compound Polycycle, Inter. J. Bifur. Chaos, 2020, 30, 2050099. doi: 10.1142/S0218127420500996
CrossRef Google Scholar
|
|
[26]
|
X. Sun, M. Han and J. Yang, Bifurcation of limit cycles from a heteroclinic loop with a cusp, Nonlinear Anal. (TMA), 2011, 74, 2948-2965. doi: 10.1016/j.na.2011.01.013
CrossRef Google Scholar
|
|
[27]
|
X. Sun and J. Yang, Sharp bounds of the number of zeros of Abelian integrals with parameters, E. J. Differ. Equat., 2014, 40, 1-12.
Google Scholar
|
|
[28]
|
D. Xiao, Bifurcations on a five-parameter family of planar vector field, J. Dynam. Differ. Equat., 2008, 20, 961-980. doi: 10.1007/s10884-008-9109-2
CrossRef Google Scholar
|
|
[29]
|
Y. Xiong and M. Han, A Note on the Expansion of the First Order Melnikov Function Near a Class of 3-polycycle, J. Nonl. Model. Anal., 2020, 2, 125-130.
Google Scholar
|
|
[30]
|
J. Yang and X. Sun, Bifurcation of limit cycles for some Lienard systems with a nilpotent singular point, Inter. J. Bifur. Chaos, 2015, 25, 1550066(14pages), doi: 10.1142/S0218127415500662
CrossRef Google Scholar
|
|
[31]
|
J. Yang and F. Liang, Limit cycle bifurcations of a kind of Lienard system with a hyperbolic saddle and a nilpotent cusp, J. Appl. Anal. Compu., 2015, 5:3, 515-526.
Google Scholar
|
|
[32]
|
L. Zhao and D. Li, Bifurcations of limit cycles from a quintic Hamiltonian system with a heteroclinic cycle, Acta Math. Sinica, 2014, 30, 411-422. doi: 10.1007/s10114-014-2615-8
CrossRef Google Scholar
|




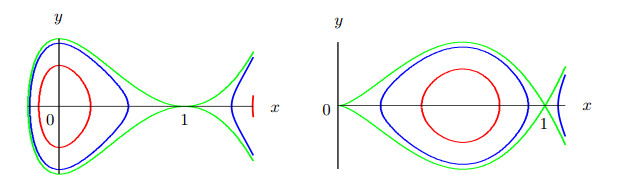

 DownLoad:
DownLoad: