FINITE-TIME SYNCHRONIZATION FOR COUPLED SYSTEMS WITH TIME DELAY AND STOCHASTIC DISTURBANCE UNDER FEEDBACK CONTROL

Yao Xu; Rong Shen; Wenxue Li

doi:10.11948/20180051

2020 Volume 10 Issue 1

Article Contents

Article navigation > Journal of Applied Analysis & Computation > 2020 > 10(1): 1-24

Next Article Previous Article

Yao Xu, Rong Shen, Wenxue Li. FINITE-TIME SYNCHRONIZATION FOR COUPLED SYSTEMS WITH TIME DELAY AND STOCHASTIC DISTURBANCE UNDER FEEDBACK CONTROL[J]. Journal of Applied Analysis & Computation, 2020, 10(1): 1-24. doi: 10.11948/20180051

Citation:

Yao Xu, Rong Shen, Wenxue Li. FINITE-TIME SYNCHRONIZATION FOR COUPLED SYSTEMS WITH TIME DELAY AND STOCHASTIC DISTURBANCE UNDER FEEDBACK CONTROL[J]. Journal of Applied Analysis & Computation, 2020, 10(1): 1-24. doi: 10.11948/20180051

FINITE-TIME SYNCHRONIZATION FOR COUPLED SYSTEMS WITH TIME DELAY AND STOCHASTIC DISTURBANCE UNDER FEEDBACK CONTROL

1.
Department of Mathematics, Harbin Institute of Technology (Weihai), Weihai, 264209, China

Corresponding author: Email address: wenxue810823@163.com, wenxuetg@hitwh.edu.cn (W. Li)

Abstract

Abstract

This paper proposes a framework for finite-time synchronization of coupled systems with time delay and stochastic disturbance under feedback control. Combining Kirchhoff's Matrix Tree Theorem with Lyapunov method as well as stochastic analysis techniques, several sufficient conditions are derived. Differing from previous references, the finite time provided by us is related to topological structure of networks. In addition, two concrete applications about stochastic coupled oscillators with time delay and stochastic Lorenz chaotic coupled systems with time delay are presented, respectively. Besides, two synchronization criteria are provided. Ultimately, two numerical examples are given to illustrate the effectiveness and feasibility of the obtained results.
- Finite-time synchronization /
- stochastic coupled systems /
- feedback control /
- time delay
MSC: 34D06, 93B52, 93E15

FullText(HTML)

References

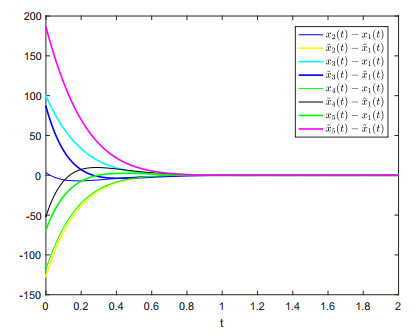
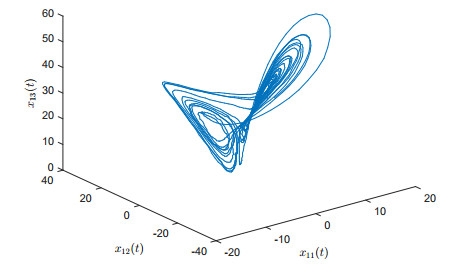
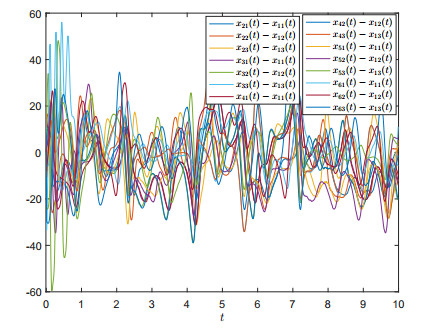
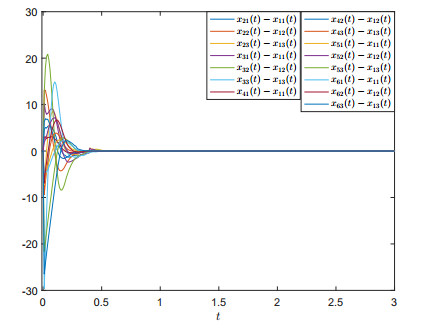
[1]	M. P. Aghababa, S. Khanmohammadi and G. Alizadeh, Finite-time synchronization of two different chaotic systems with unknown parameters via sliding mode technique, Appl. Math. Model., 2011, 35(6), 3080-3091. doi: 10.1016/j.apm.2010.12.020 CrossRef Google Scholar
[2]	I. Ahmad, A. Saaban, A. Ibrahim and M. Shahzad, Robust finite-time anti-synchronization of chaotic systems with different dimensions, Mathematics, 2015, 3(4), 1222-1240. doi: 10.3390/math3041222 CrossRef Google Scholar
[3]	J. Almeida, C. Silvestre and A. M. Pascoal, Event-triggered output synchronization of heterogeneous multi-agent systems, Int. J. Robust Nonlinear Control, 2017, 27(8), 1302-1338. doi: 10.1002/rnc.3629 CrossRef Google Scholar
[4]	A. Bondavalli, F. Brancati, A. Flammini and S. Rinaldi, Master failure detection protocol in internal synchronization environment, IEEE Trans. Instrum. Meas., 2012, 62(1), 4-12. Google Scholar
[5]	Y. Cai, Y. Kang and W. Wang, A stochastic sirs epidemic model with nonlinear incidence rate, Appl. Math. Comput., 2017, 305, 221-240. Google Scholar
[6]	J. Cao, Z. Wang and Y. Sun, Synchronization in an array of linearly stochastically coupled networks with time delays, Physica A, 2007, 385(2), 718-728. doi: 10.1016/j.physa.2007.06.043 CrossRef Google Scholar
[7]	T. Chen, Z. Sun and B. Wu, Stability of multi-group models with cross-dispersal based on graph theory, Appl. Math. Model., 2017, 47, 745-754. doi: 10.1016/j.apm.2017.03.055 CrossRef Google Scholar
[8]	H. Du, Y. He and Y. Cheng, Finite-time synchronization of a class of second-order nonlinear multi-agent systems using output feedback control, IEEE Trans. Circuits Syst. I-Regul. Pap., 2014, 61(6), 1778-1788. doi: 10.1109/TCSI.2013.2295012 CrossRef Google Scholar
[9]	J. Geng, M. Liu and Y. Zhang, Stability of a stochastic one-predator-two-prey population model with time delays, Commun. Nonlinear Sci. Numer. Simul., 2017, 53, 65-82. doi: 10.1016/j.cnsns.2017.04.022 CrossRef Google Scholar
[10]	B. Guo, Y. Xiao and C. Zhang, Graph-theoretic approach to exponential synchronization of coupled systems on networks with mixed time-varying delays, J. Franklin Inst., 2017, 354(12), 5067-5090. doi: 10.1016/j.jfranklin.2017.05.029 CrossRef Google Scholar
[11]	Y. Guo, X. Ding and Y. Li, Stochastic stability for pantograph multi-group models with dispersal and stochastic perturbation, J. Franklin Inst., 2016, 353(13), 2980-2998. doi: 10.1016/j.jfranklin.2016.06.001 CrossRef Google Scholar
[12]	K. Hong and P. Weng, Stability and traveling waves of diffusive predator-prey model with age-structure and nonlocal effect, J. Appl. Anal. Comput., 2012, 2(2), 173-192. Google Scholar
[13]	D. Huang, M. Jiang and J. Jian, Finite-time synchronization of inertial memristive neural networks with time-varying delays via sampled-date control, Neurocomputing, 2017, 266, 527-539. doi: 10.1016/j.neucom.2017.05.075 CrossRef Google Scholar
[14]	M. Karimi-Ghartemani and M. R. Iravani, A method for synchronization of power electronic converters in polluted and variable-frequency environments, IEEE Trans. Power Syst., 2004, 19(3), 1263-1270. Google Scholar
[15]	M. Y. Li and Z. Shuai, Global-stability problem for coupled systems of differential equations on networks, J. Differ. Equ., 2010, 248(1), 1-20. Google Scholar
[16]	S. Li, H. Su and X. Ding, Synchronized stationary distribution of hybrid stochastic coupled systems with applications to coupled oscillators and a chua's circuits network, J. Franklin Inst., 2018, 355(17), 8743-8765. doi: 10.1016/j.jfranklin.2018.09.015 CrossRef Google Scholar
[17]	W. Li, S. Wang, H. Su and K. Wang, Global exponential stability for stochastic networks of coupled oscillators with variable delay, Commun. Nonlinear Sci. Numer. Simul., 2015, 22(1–3), 877-888. Google Scholar
[18]	M. Liu, Dynamics of a stochastic regime-switching predator-prey model with modified leslie-gower holling-type ii schemes and prey harvesting, Nonlinear Dyn., 2019, 96(1), 417-442. Google Scholar
[19]	Y. Liu, W. Li and J. Feng, The stability of stochastic coupled systems with time-varying coupling and general topology structure, IEEE Trans. Neural Netw. Learn. Syst., 2018, 29(9), 4189-4200. doi: 10.1109/TNNLS.2017.2757767 CrossRef Google Scholar
[20]	X. Mao, Stochastic Differential Equations and Their Applications Horwood, 1997. Google Scholar
[21]	X. Peng, H. Wu, K. Song and J. Shi, Global synchronization in finite time for fractional-order neural networks with discontinuous activations and time delays., Neural Netw., 2017, 94, 46-54. doi: 10.1016/j.neunet.2017.06.011 CrossRef Google Scholar
[22]	R. Trudeau, Introduction to graph theory, Courier Dover Publications, 2013. Google Scholar
[23]	D. Wang, S. Liu, K. Liu and Y. Zhao, Control and synchronization of julia sets generated by a class of complex time-delay rational, J. Appl. Anal. Comput., 2016, 6(4), 1049-1063. Google Scholar
[24]	H. Wang, J.-P. Wu, X.-S. Sheng et al., A new stability result for nonlinear cascade time-delay system and its application in chaos control, Nonlinear Dyn., 2015, 80(1-2), 221-226. doi: 10.1007/s11071-014-1862-7 CrossRef Google Scholar
[25]	J. Wang, H. Zhang, Z. Wang and D. W. Gao, Finite-time synchronization of coupled hierarchical hybrid neural networks with time-varying delays, IEEE Trans. Cybern., 2017, 47(10), 2995-3004. doi: 10.1109/TCYB.2017.2688395 CrossRef Google Scholar
[26]	M. Wang and W. Li, Stability of random impulsive coupled systems on networks with markovian switching, Stoch. Anal. Appl., 2019, DOI: 10.1080/07362994.2019.1643247, 1-26. CrossRef Google Scholar
[27]	P. Wang, Y. Hong and H. Su, Asymptotic stability in probability for discrete-time stochastic coupled systems on networks with multiple dispersal, Int. J. Robust Nonlinear Control, 2017, 28(4), 1119-1217. Google Scholar
[28]	P. Wang, B. Zhang and H. Su, Stabilization of stochastic uncertain complex-valued delayed networks via aperiodically intermittent nonlinear control, IEEE Trans. Syst., Man, Cybern., Syst., 2018, 49(3), 649-662. Google Scholar
[29]	X. Wang, J. A. Fang, H. Mao and A. Dai, Finite-time global synchronization for a class of markovian jump complex networks with partially unknown transition rates under feedback control, Nonlinear Dyn., 2015, 79(1), 47-61. Google Scholar
[30]	Z. Wang, Y. Wang and Y. Liu, Global synchronization for discrete-time stochastic complex networks with randomly occurred nonlinearities and mixed time delays, IEEE Trans. Neural Netw., 2009, 21(1), 11-25. Google Scholar
[31]	D. J. Watts and S. H. Strogatz, Collective dynamics of 'small-world' networks., Nature, 1998, 393(6684), 440. doi: 10.1038/30918 CrossRef Google Scholar
[32]	D. West, Introduction to Graph Theory. Prentice Hall, 21, 1996. Google Scholar
[33]	K. Wu, T. Tian, L. Wang and W. Wang, Asymptotical synchronization for a class of coupled time-delay partial differential systems via boundary control, Neurocomputing, 2016, 197, 113-118. doi: 10.1016/j.neucom.2016.02.050 CrossRef Google Scholar
[34]	X. Wu, C. Xu and J. Feng, Complex projective synchronization in drive-response stochastic coupled networks with complex-variable systems and coupling time delays, Commun. Nonlinear Sci. Numer. Simul., 2015, 20(3), 1004-1014. doi: 10.1016/j.cnsns.2014.07.003 CrossRef Google Scholar
[35]	Y. Wu, B. Chen and W. Li, Synchronization of stochastic coupled systems via feedback control based on discrete-time state observations, Nonlinear Anal. Hybrid Syst, 2017, 26, 68-85. doi: 10.1016/j.nahs.2017.04.006 CrossRef Google Scholar
[36]	Y. Wu, J. Zhu and W. Li, Intermittent discrete observation control for synchronization of stochastic neural networks, IEEE Trans. Cybern., 2019, DOI: 10.1109/TCYB.2019.2930579. CrossRef Google Scholar
[37]	Q. Xie, G. Si, Y. Zhang et al., Finite-time synchronization and identification of complex delayed networks with markovian jumping parameters and stochastic perturbations, Chaos Solitons Fractals, 2016, 86, 35-49. doi: 10.1016/j.chaos.2016.02.021 CrossRef Google Scholar
[38]	Y. Xu, Q. Li and W. Li, Periodically intermittent discrete observation control for synchronization of fractional-order coupled systems, Commun. Nonlinear Sci. Numer. Simul., 2019, 74, 219-235. doi: 10.1016/j.cnsns.2019.03.014 CrossRef Google Scholar
[39]	Y. Xu, H. Zhou and W. Li, Stabilisation of stochastic delayed systems with lévy noise on networks via periodically intermittent control, Int. J. Control, 2018, DOI: 10.1080/00207179.2018.1479538, 1-14. CrossRef Google Scholar
[40]	J. Yin, Y. Li and A. Gu, Regularity of pullback attractors for non-autonomous stochastic coupled reaction-diffusion systems, J. Appl. Anal. Comput., 2017, 7(3), 884-898. Google Scholar
[41]	W. Ying, J. Cao and G. Wen, Quantized synchronization of chaotic neural networks with scheduled output feedback control, IEEE Trans. Neural Netw. Learn. Syst., 2017, 28(11), 2638-2647. doi: 10.1109/TNNLS.2016.2598730 CrossRef Google Scholar
[42]	W. Yu, G. Chen and J. Cao, Adaptive synchronization of uncertain coupled stochastic complex networks, Asian J. Control, 2011, 13(3), 418-429. Google Scholar
[43]	S. Zhai, Y. Zhou and Q. Li, Synchronization for coupled nonlinear systems with disturbances in input and measured output, Appl. Math. Comput., 2017, 294, 227-237. Google Scholar
[44]	C. Zhang, W. Li and K. Wang, A graph-theoretic approach to stability of neutral stochastic coupled oscillators network with time-varying delayed coupling, Math. Meth. Appl. Sci., 2014, 37(8), 1179-1190. doi: 10.1002/mma.2879 CrossRef Google Scholar
[45]	C. Zhang and L. Shi, Exponential synchronization of stochastic complex networks with multi-weights: A graph-theoretic approach, Journal of the Franklin Institute, 2019, 356(7), 4106-4123. doi: 10.1016/j.jfranklin.2019.02.027 CrossRef Google Scholar
[46]	H. Zhou and W. Li, Synchronisation of stochastic-coupled intermittent control systems with delays and lévy noise on networks without strong connectedness, IET Control Theory Appl., 2018, 13(1), 36-49. Google Scholar