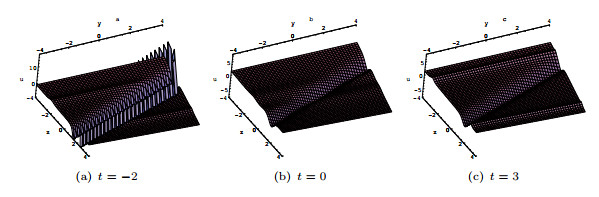
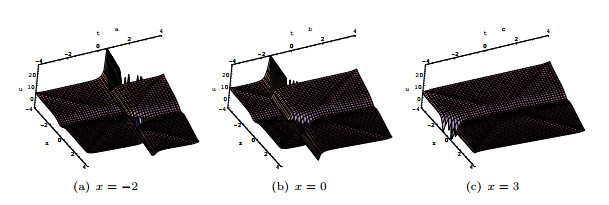
|
[1]
|
A. Biswas, A. Sonmezoglu, M. Ekici et al., Optical soliton perturbation with fractional temporal evolution by extended (G'/G)-expansion method, Optik., 2018, 161, 301-320. doi: 10.1016/j.ijleo.2018.02.051
CrossRef Google Scholar
|
|
[2]
|
S. T. Chen, W. X. Ma, Lump solutions of a generalized Calogero-Bogoyavlenskii-Schiff equation, Comput. Math. Appl., 2018, 76(7), 1680-1685. doi: 10.1016/j.camwa.2018.07.019
CrossRef Google Scholar
|
|
[3]
|
Y. M. Chen, S. H. Ma, Z. Y. Ma, New exact solutions of (3+1)-dimensional Jimbo-Miwa system, Chin. Phys. B., 2013, 22(5), 050510. doi: 10.1088/1674-1056/22/5/050510
CrossRef Google Scholar
|
|
[4]
|
C. Q. Dai, X. F. Zhang, Y. Fan et al., Localized modes of the (n+1)-dimensional schrödinger equation with power-law nonlinearities in PT-symmetric potentials, Commun. Nonlinear. Sci., 2017, 43, 239-250. doi: 10.1016/j.cnsns.2016.07.002
CrossRef Google Scholar
|
|
[5]
|
J. Gao, L. J. Han, Y. H. Huang, Solitary Waves for the Generalized Nonautonomous Dual-power Nonlinear Schrödinger Equations with Variable Coefficients, Journal of Nonlinear Modeling and Analysis, 2019, 1, 251-260.
Google Scholar
|
|
[6]
|
B. Ghanbari, M. S. Osman, D. Baleanu, Generalized exponential rational function method for extended Zakharov-Kuzetsov equation with conformable derivative, Mod. Phys. Lett. A, 2019. DOI: 10.1142/S0217732319501554.
CrossRef Google Scholar
|
|
[7]
|
Y. Kong, L. Xin, Q. Qiu et al., Exact periodic wave solutions for the modified Zakharov equations with a quantum correction, Appl. Math. Lett., 2019, 94, 140-148. doi: 10.1016/j.aml.2019.01.009
CrossRef Google Scholar
|
|
[8]
|
Z. Z. Lan, W. Q. Hu, B. L. Guo, General propagation lattice Boltzmann model for a variable-coefficient compound KdV-Burgers equation, Appl. Math. Model., 2019. DOI: 10.1016/j.apm.2019.04.013.
CrossRef Google Scholar
|
|
[9]
|
F. H. Lin, S. T. Chen, Q. X. Qu et al., Resonant multiple wave solutions to a new (3+1)-dimensional generalized Kadomtsev-Petviashvili equation: Linear superposition principle, Appl. Math. Lett., 2018, 78, 112-117. doi: 10.1016/j.aml.2017.10.013
CrossRef Google Scholar
|
|
[10]
|
Y. Z. Li, J. G. Liu, Multiple periodic-soliton solutions of the (3+1)-dimensional generalised shallow water equation, Pramana, 2018, 90, 71. doi: 10.1007/s12043-018-1568-3
CrossRef Google Scholar
|
|
[11]
|
J. G. Liu, Y. He, New periodic solitary wave solutions for the (3+1)-dimensional generalized shallow water equation, Nonlinear Dyn., 2017, 90, 363-369. doi: 10.1007/s11071-017-3667-y
CrossRef Google Scholar
|
|
[12]
|
W. X. Ma, X. L. Yong, H. Q. Zhang, Diversity of interaction solutions to the (2+1)-dimensional Ito equation, Comput. Math. Appl., 2018, 75(1), 289-295. doi: 10.1016/j.camwa.2017.09.013
CrossRef Google Scholar
|
|
[13]
|
W. X. Ma, Riemann-Hilbert problems of a six-component fourth-order AKNS system and its soliton solutions, Comput. Appl. Math., 2018, 37, 6359-6375. doi: 10.1007/s40314-018-0703-6
CrossRef Google Scholar
|
|
[14]
|
W. X. Ma, Lumps and their interaction solutions of (3+1)-dimensional linear PDEs, J. Geom. Phys., 2018, 133, 10-16. doi: 10.1016/j.geomphys.2018.07.003
CrossRef Google Scholar
|
|
[15]
|
W. X. Ma, J. Li, C. M. Khalique, A Study on Lump Solutions to a Generalized Hirota-Satsuma-Ito Equation in (2+1)-Dimensions, Complexity, 2018, 11, 9059858.
Google Scholar
|
|
[16]
|
X. H. Meng, Rational solutions in Grammian form for the (3+1)-dimensional generalized shallow water wave equation, Comput. Math. Appl., 2018, 75, 4534-4539. doi: 10.1016/j.camwa.2018.03.046
CrossRef Google Scholar
|
|
[17]
|
M. Mirzazadeh, M. Eslami, A. Biswas, Soliton solutions of the generalized Klein-Gordon equation by using G'/G-expansion method, Comput. Appl. Math., 2014, 33(3), 831-839. doi: 10.1007/s40314-013-0098-3
CrossRef Google Scholar
|
|
[18]
|
M. S. Osman, Multiwave solutions of time-fractional (2+1)-dimensional Nizhnik-Novikov-Veselov equations, pramana, 2017, 88, 67. doi: 10.1007/s12043-017-1374-3
CrossRef Google Scholar
|
|
[19]
|
M. S. Osman, On complex wave solutions governed by the 2d ginzburg-landau equation with variable coefficients, Optik., 2018, 156, 169-174. doi: 10.1016/j.ijleo.2017.10.127
CrossRef Google Scholar
|
|
[20]
|
M. S. Osman, D. C. Lu, M. A. Khater, A study of optical wave propagation in the nonautonomous Schrödinger-Hirota equation with power-law nonlinearity, Results Phys., 2019, 13, 102157. doi: 10.1016/j.rinp.2019.102157
CrossRef Google Scholar
|
|
[21]
|
M. S. Osman, J.A.T. Machado, New nonautonomous combined multi-wave solutions for (2+1)-dimensional variable coefficients KdV equation, Nonlinear Dyn., 2018, 93, 733-740. doi: 10.1007/s11071-018-4222-1
CrossRef Google Scholar
|
|
[22]
|
M. S. Osman, One-soliton shaping and inelastic collision between double solitons in the fifth-order variable-coefficient Sawada-Kotera equation, Nonlinear Dyn., 2019, 96, 1491-1496. doi: 10.1007/s11071-019-04866-1
CrossRef Google Scholar
|
|
[23]
|
M. S. Osman, B. Ghanbari, J. A. T. Machado, New complex waves in nonlinear optics based on the complex Ginzburg-Landau equation with Kerr law nonlinearity, Eur. Phys. J. Plus., 2019, 134, 20. doi: 10.1140/epjp/i2019-12442-4
CrossRef Google Scholar
|
|
[24]
|
M. S. Osman, B. Ghanbari, New optical solitary wave solutions of Fokas-Lenells equation in presence of perturbation terms by a novel approach, Optik., 2018, 175, 328-333. doi: 10.1016/j.ijleo.2018.08.007
CrossRef Google Scholar
|
|
[25]
|
M. S. Osman, J. A. T. Machado, The dynamical behavior of mixed-type soliton solutions described by (2+1)-dimensional Bogoyavlensky-Konopelchenko equation with variable coefficients, J. Electromagnet. Wave., 2018, 32, 1457-1464. doi: 10.1080/09205071.2018.1445039
CrossRef Google Scholar
|
|
[26]
|
M. S. Osman, H. I. Abdel-Gawad, M. A. El Mahdy, Two-layer-atmospheric blocking in a medium with high nonlinearity and lateral dispersion, Results Phys., 2018, 8, 1054-1060. doi: 10.1016/j.rinp.2018.01.040
CrossRef Google Scholar
|
|
[27]
|
K. U. Tariq, M. Younis, R. Hadi et al., Optical solutions to the space-time fractional nonlinear Schrödinger equation with quadratic-cubic nonlinearity, Mod. Phys. Lett. B, 2018, 32(26), 1850317. doi: 10.1142/S0217984918503177
CrossRef Google Scholar
|
|
[28]
|
Y. N. Tang, W. X. Ma, W. Xu, Grammian and Pfaffian solutions as well as Pfaffianization for a (3+1)-dimensional generalized shallow water equation, Chin. Phys. B., 2012, 21(7), 070212. doi: 10.1088/1674-1056/21/7/070212
CrossRef Google Scholar
|
|
[29]
|
B. Tian, Y. T. Gao, Beyond travelling waves: a new algorithm for solving nonlinear evolution equations, Comput. Phys. Commun., 2996, 95, 139-142.
Google Scholar
|
|
[30]
|
H. Wang, Lump and interaction solutions to the (2+1)-dimensional Burgers equation, Appl. Math. Lett., 2018, 85, 27-34. doi: 10.1016/j.aml.2018.05.010
CrossRef Google Scholar
|
|
[31]
|
E. M. E. Zayed, New traveling wave solutions for higher dimensional nonlinear evolution equations using a generalized (G'/G)-expansion method, J. Phys. A: Math. Theor., 2019, 42(19), 195202-195214.
Google Scholar
|
|
[32]
|
E. M. E. Zayed, K. A. Gepreel, The (G'/G)-expansion method for finding traveling wave solutions of nonlinear partial differential equations in mathematical physics, J. Math. Phys., 2009, 50, 013502. doi: 10.1063/1.3033750
CrossRef Google Scholar
|
|
[33]
|
E. M. E. Zayed, Traveling wave solutions for higher dimensional nonlinear evolution equations using the (G'/G)-expansion method, J. Appl. Math. Inform., 2010, 28, 383-395.
Google Scholar
|
|
[34]
|
Z. F. Zeng, J. G. Liu, B. Nie, Multiple-soliton solutions, soliton-type solutions and rational solutions for the (3+1)-dimensional generalized shallow water equation in oceans, estuaries and impoundments, Nonlinear Dyn., 2016, 86, 667-675. doi: 10.1007/s11071-016-2914-y
CrossRef Google Scholar
|
|
[35]
|
S. Zhang, J. Tong, W. Wang, A generalized (G'/G)-expansion method for the mKdV equation with variable coefficients, Phys. Lett. A., 2008, 372, 2254-2257. doi: 10.1016/j.physleta.2007.11.026
CrossRef Google Scholar
|
|
[36]
|
Y. Zhou, S. Manukure, W. X. Ma, Lump and lump-soliton solutions to the Hirota-Satsuma-Ito equation, Commun. Nonlinear Sci. Numer. Simulat., 2019, 68, 56-62. doi: 10.1016/j.cnsns.2018.07.038
CrossRef Google Scholar
|
|
[37]
|
D. W. Zuo, Y.T. Gao, G. Q. Meng et al., Multi-soliton solutions for the three-coupled kdv equations engendered by the neumann system, Nonlinear Dyn., 2014, 75(4), 1-8.
Google Scholar
|




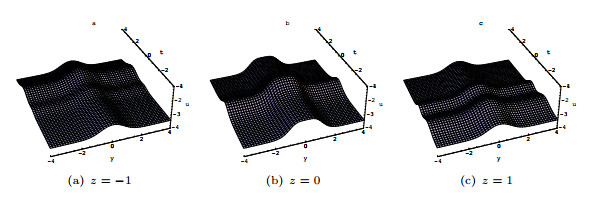

 DownLoad:
DownLoad: