SOLVING AN INVERSE PROBLEM FOR A GENERALIZED TIME-DELAYED BURGERS-FISHER EQUATION BY HAAR WAVELET METHOD

Saedeh Foadian; Reza Pourgholi; S. Hashem Tabasi; Hamed Zeidabadi

doi:10.11948/20170028

2020 Volume 10 Issue 2

Article Contents

Article navigation > Journal of Applied Analysis & Computation > 2020 > 10(2): 391-410

Next Article Previous Article

Saedeh Foadian, Reza Pourgholi, S. Hashem Tabasi, Hamed Zeidabadi. SOLVING AN INVERSE PROBLEM FOR A GENERALIZED TIME-DELAYED BURGERS-FISHER EQUATION BY HAAR WAVELET METHOD[J]. Journal of Applied Analysis & Computation, 2020, 10(2): 391-410. doi: 10.11948/20170028

Citation:

Saedeh Foadian, Reza Pourgholi, S. Hashem Tabasi, Hamed Zeidabadi. SOLVING AN INVERSE PROBLEM FOR A GENERALIZED TIME-DELAYED BURGERS-FISHER EQUATION BY HAAR WAVELET METHOD[J]. Journal of Applied Analysis & Computation, 2020, 10(2): 391-410. doi: 10.11948/20170028

SOLVING AN INVERSE PROBLEM FOR A GENERALIZED TIME-DELAYED BURGERS-FISHER EQUATION BY HAAR WAVELET METHOD

1.
School of Mathematics and Computer Science, Damghan University, P.O.Box 36715-364, Damghan, Iran

Corresponding author: Email address:pourgholi@du.ac.ir (R. Pourgholi) Tel.: +982335220092. Fax: +982335235316

Abstract

Abstract

In this paper, a numerical method consists of combining Haar wavelet method and Tikhonov regularization method to determine unknown boundary condition and unknown nonlinear source term for the generalized time-delayed Burgers-Fisher equation using noisy data is presented. A stable numerical solution is determined for the problem. We also show that the rate of convergence of the method is as exponential $ \Bigl(O\left(\frac{1}{2^{J+1}}\right)\Bigr) $, where $ J $ is maximal level of resolution of wavelet. Some numerical results are reported to show the efficiency and robustness of the proposed approach for solving the inverse problems.
- Ill-posed inverse problems /
- Haar wavelet method /
- Tikhonov regularization method /
- error estimation /
- convergence analysis
MSC: 65N20, 65N21, 65M32, 35K05

FullText(HTML)

References

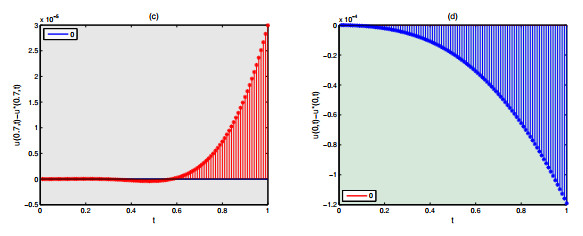
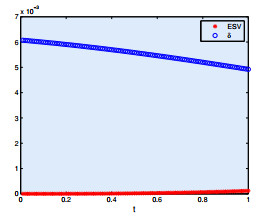
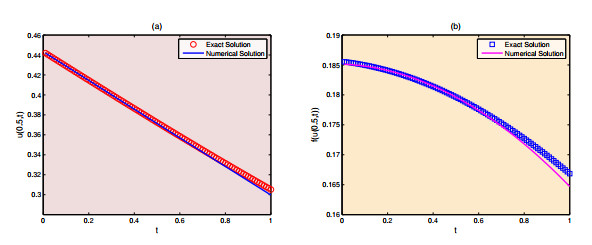
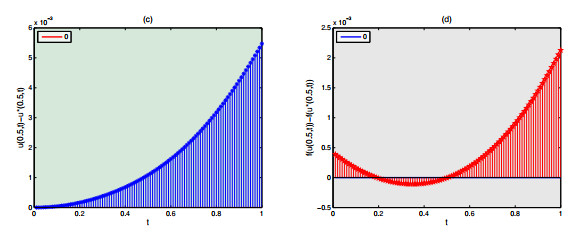
[1]	M. Abtahi, R. Pourgholi and A. Shidfar, Existence and uniqueness of solution for a two dimensional nonlinear inverse diffusion problem, Nonlinear Analysis: Theory, Methods & Applications, 2011, 74(7), 2462-2467. Google Scholar
[2]	O. M. Alifanov, Inverse Heat Transfer Problems, Springer, NewYork, 1994. Google Scholar
[3]	A. J. Ammerman and L. L. Cavalli-Sforza, The Neolithic Transition and the Genetics of Population in Europe, Princeton University Press, 1984. Google Scholar
[4]	J. V. Beck, B. Blackwell and C. R. St. Clair, Inverse Heat Conduction: IllPosed Problems, Wiley-Interscience, NewYork, 1985. Google Scholar
[5]	J. V. Beck and D. C. Murio, Combined function specification-regularization procedure for solution of inverse heat condition problem, AIAA J., 1986, 24(1), 180-185. doi: 10.2514/3.9240 CrossRef Google Scholar
[6]	J. M. G Cabeza, J. A. M Garcia and A. C. Rodriguez, A Sequential Algorithm of Inverse Heat Conduction Problems Using Singular Value Decomposition, International Journal of Thermal Sciences, 2005, 44(3), 235-244. doi: 10.1016/j.ijthermalsci.2004.06.009 CrossRef Google Scholar
[7]	C. F. Chen and C. H. Hsiao, Haar wavelet method for solving lumped and distributed-parameter systems, IEE Proc.: part D, 1997, 144(1), 87-94. Google Scholar
[8]	L. Elden, A Note on the Computation of the Generalized Cross-validation Function for Ill-conditioned Least Squares Problems, BIT, 1984, 24(4), 467-472. doi: 10.1007/BF01934905 CrossRef Google Scholar
[9]	E. S. Fahmy, Travelling wave solution for some time-delayed equations through factorizations, Chaos Solitions Fract., 2008, 38(4), 1209-1216. Google Scholar
[10]	S. Foadian, R. Pourgholi and S. Hashem Tabasi, Cubic B-spline method for the solution of an inverse parabolic system, Applicable Analysis, 2018, 97(3), 438-465. doi: 10.1080/00036811.2016.1272102 CrossRef Google Scholar
[11]	P. K. Galenko and D. A. Danilov, Selection of the dynamically stable regime of rapid solidification front motion in an isothermal binary alloy, J. Cryst. Growth, 2000, 216(1-4), 512-536. doi: 10.1016/S0022-0248(00)00338-9 CrossRef Google Scholar
[12]	G. H. Golub, M. Heath and G. Wahba, Generalized Cross-validation as a Method for Choosing a Good Ridge Parameter, Technometrics, 1979, 21(2), 215-223. doi: 10.1080/00401706.1979.10489751 CrossRef Google Scholar
[13]	A. Haar, Zur theorie der orthogonalen Funktionsysteme, Math. Annal., 1910, 69, 331-371. doi: 10.1007/BF01456326 CrossRef Google Scholar
[14]	P. C. Hansen, Analysis of discrete ill-posed problems by means of the L-curve, SIAM Rev., 1992, 34(4), 561-80. doi: 10.1137/1034115 CrossRef Google Scholar
[15]	G. Hariharan, K. Kannan and K. R. Sharma, Haar wavelet method for solving Fisher's equation, Applied Mathematics and Computation, 2009, 211(2), 284-292. doi: 10.1016/j.amc.2008.12.089 CrossRef Google Scholar
[16]	C. H. Hsiao and W. J. Wang, Haar wavelet approach to nonlinear stiff systems, Math. Comput. Simul., 2001, 57(6), 347-353. doi: 10.1016/S0378-4754(01)00275-0 CrossRef Google Scholar
[17]	C. H. Huang and Y. L. Tsai, A transient 3-D inverse problem in imaging the time-dependentlocal heat transfer coefficients for plate fin, Applied Therma Engineering, 2005, 25(14-15), 2478-2495. doi: 10.1016/j.applthermaleng.2004.12.003 CrossRef Google Scholar
[18]	R. Kalpana and S. R. Balachandar, Haar wavelet method for the analysis of transistor circuits, Int. J. Electron. Commun. (AEU), 2007, 61(9), 589-594. doi: 10.1016/j.aeue.2006.10.003 CrossRef Google Scholar
[19]	C. L. Lawson and R. J. Hanson, Solving Least Squares Problems, Philadelphia, PA: SIAM, 1995. Google Scholar
[20]	L. Martin, L. Elliott, P. J. Heggs, D. B. Ingham, D. Lesnic and X. Wen, Dual Reciprocity Boundary Element Method Solution of the Cauchy Problem for Helmholtz-type Equations with Variable Coefficients, Journal of sound and vibration, 2006, 297(1-2), 89-105. doi: 10.1016/j.jsv.2006.03.045 CrossRef Google Scholar
[21]	H. Molhem and R. Pourgholi, A numerical algorithm for solving a one-dimensional inverse heat conduction problem, Journal of Mathematics and Statistics, 2008, 4(1), 60-63. Google Scholar
[22]	D. C. Murio and J. R. Paloschi, Combined mollification-future temperature procedure for solution of inverse heat conduction problem, J. comput. Appl. Math., 1988, 23(2), 235-244. doi: 10.1016/0377-0427(88)90282-8 CrossRef Google Scholar
[23]	D. A. Murio, The Mollification Method and the Numerical Solution of Ill-Posed Problems, Wiley-Interscience, NewYork, 1993. Google Scholar
[24]	S. Rendine, A. Piazza and L. L. Cavalli-Sforza, Simulation and separation by principle components of multiple demic expansions in Europe, Am. Nat., 1986, 128(5), 681-706. doi: 10.1086/284597 CrossRef Google Scholar
[25]	R. Pourgholi, H. Dana and S. H. Tabasi, Solving an inverse heat conduction problem using genetic algorithm: Sequential and multi-core parallelization approach, Applied Mathematical Modelling, 2014, 38(7-8), 1948-1958. doi: 10.1016/j.apm.2013.10.019 CrossRef Google Scholar
[26]	R. Pourgholi, A. Esfahani, S. Foadian and S. Parehkar, Resolution of an inverse problem by Haar basis and Legendre wavelet methods, IJWMIP., 2013, 11(05), 1350034 (21 pages). Google Scholar
[27]	R. Pourgholi, S. Foadian and A. Esfahani, Haar basis method to solve some inverse problems for two-dimensional parabolic and hyperbolic equations, TWMS J. App. Eng. Math., 2013, 3(1), 10-32. Google Scholar
[28]	R. Pourgholi, M. Rostamian and M. Emamjome, A numerical method for solving a nonlinear inverse parabolic problem, Inverse Problems in Science and Engineering, 2010, 18(8), 1151-1164. doi: 10.1080/17415977.2010.518287 CrossRef Google Scholar
[29]	R. Pourgholi, N. Tavallaie and S. Foadian, Applications of Haar basis method for solving some ill-posed inverse problems, J. Math. Chem., 2012, 50(8), 2317-2337. doi: 10.1007/s10910-012-0036-4 CrossRef Google Scholar
[30]	A. N. Tikhonov and V. Y. Arsenin, On the solution of ill-posed problems, New York, Wiley, 1977. Google Scholar
[31]	A. N. Tikhonov and V. Y. Arsenin, Solution of Ill-Posed Problems, V.H. Winston and Sons, Washington, DC, 1977. Google Scholar
[32]	X. Y. Wang and Y. K. Lu, Exact solutions of the extended Burgers-Fisher equation, Chinese Phys. Lett., 1990, 7(4), 145-147. doi: 10.1088/0256-307X/7/4/001 CrossRef Google Scholar
[33]	J. Zhang, P. Wei and M. Wang, The investigation into the exact solutions of the generalized time-delayed Burgers-Fisher equation with positive fractional power terms, Applied Mathematical Modelling, 2012, 36(5), 2192-2196. doi: 10.1016/j.apm.2011.08.004 CrossRef Google Scholar
[34]	J. Zhou, Y. Zhang, J. K. Chen and Z. C. Feng, Inverse Heat Conduction in a Composite Slab With Pyrolysis Effect and Temperature-Dependent Thermophysical Properties, J. Heat Transfer, 2010, 132(3), 034502 (3 pages). Google Scholar