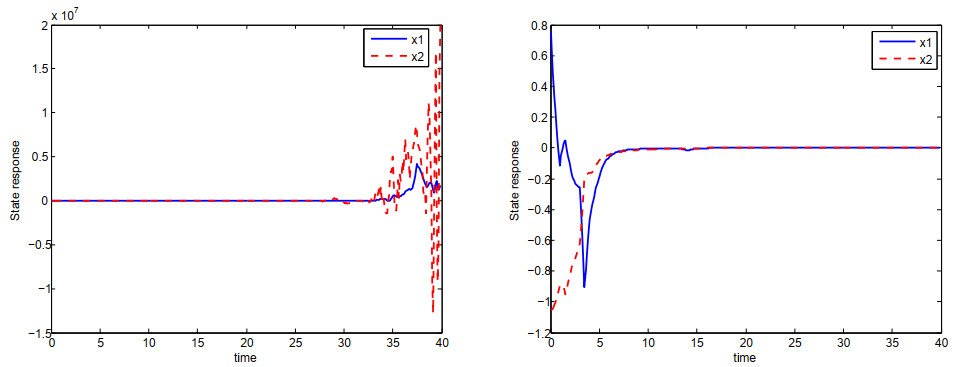
|
[1]
|
E. K. Boukas, Static output feedback control for stochastic hybrid systems: LMI approach, Automatica, 2006, 42(1), 183–188.
Google Scholar
|
|
[2]
|
E. K. Boukas, Communications and control engineering, Control of singular systems with random abrupt changes, Springer, 2008.
Google Scholar
|
|
[3]
|
Y. K. Cui, J. Shen and Y. Chen, Stability analysis for positive singular systems with distributed delays, Automatica, 2018, 94, 170–177.
Google Scholar
|
|
[4]
|
L. Y. Dai, Lectures notes in control and information sciences: Vol. 118. Singular control systems, Springer, New York, 1989.
Google Scholar
|
|
[5]
|
J. C. Geromel and P. Colaneri, Stability and stabilization of continuous-time switched linear systems, Siam J. Control Optim., 2006, 45(5), 1915–1930.
Google Scholar
|
|
[6]
|
M. A. Han and L. J. Sheng, Bifurcation of limit cycles in piecewise smooth systems via Melnikov function, J. Appl. Anal. Comput., 2015, 5(4), 809–815.
Google Scholar
|
|
[7]
|
M. A. Han, On the maximum number of periodic solutions of piecewise smooth periodic equations by average method, J. Appl. Anal. Comput., 2017, 7(2), 788–794.
Google Scholar
|
|
[8]
|
Y. He, M. Wu, J. H. She and G. P. Liu, Delay-dependent robust stability criteria for uncertain neutral systems with mixed delays, Syst. Control Lett., 2004, 51(1), 57–65.
Google Scholar
|
|
[9]
|
M. G. Hua, D. D. Zheng and F. Q. Deng, Partially mode-dependent $l_{2}-l_{\infty}$ filtering for discrete-time nonhomogeneous Markov jump systems with repeated scalar nonlinearities, Inform. Sciences, 2018, 451-452, 223-239.
Google Scholar
|
|
[10]
|
I. Y. Kats and A. A. Martynyuk, Stability and stabilization of nonlinear systems with random structure, Taylor & Francis, 2002.
Google Scholar
|
|
[11]
|
Y. G. Kao, J. Xie and C. H. Wang, An anti-windup design to robust $H_{\infty}$ control for singular Markovian jump systems with actuator saturation and general unknown transition rates, J. Franklin Inst., 2015, 352(12), 5708–5734.
Google Scholar
|
|
[12]
|
N. N. Krasovskii and E. A. Lidskii, Analysis design of controller in systems with random attributes-Part 2, Automat. Rem. Contr., 1961, 22, 1141–1146.
Google Scholar
|
|
[13]
|
C. M. Lee and I. K. Fong, $H_{\infty}$ optimal singular and normal filter design for uncertain singular systems, IET Control Theory A., 2007, 1(1), 119–126.
Google Scholar
|
|
[14]
|
F. B. Li, P. Shi, C. C. Lim and L. G. Wu, Fault detection filtering for nonhomogeneous Markovian jump systems via fuzzy approach, IEEE Trans. Fuzzy Syst., 2018, 26(1), 131–141.
Google Scholar
|
|
[15]
|
M. Li, M. Liu, Y. C. Zhang and Y. H. Geng, Fault tolerant sliding mode control for T-S fuzzy stochastic time-delay system via a novel sliding mode observer approach, Int. J. Syst. Sci., 2018, 49(7), 1353–1367.
Google Scholar
|
|
[16]
|
Y. K. Li, M. Chen, L. Cai, and Q. X. Wu, Resilient control based on disturbance observer for nonlinear singular stochastic hybrid system with partly unknown Markovian jump parameters, J. Franklin Inst., 2018, 355(5), 2243–2265.
Google Scholar
|
|
[17]
|
M. Z. Luo, S. M. Zhong and J. Cheng, Finite-time event-triggered control and fault detection for singular Markovian jump mixed delay systems under asynchronous switching, Adv. Differ. Equ., 2018. DOI: 10.1186/s13662-018-1533-y.
Google Scholar
|
|
[18]
|
Y. C. Ma, N. N. Ma and L. Chen, Synchronization criteria for singular complex networks with Markovian jump and time-varying delays via pinning control, Nonlinear Anal.-Hybri., 2018, 29, 85–99.
Google Scholar
|
|
[19]
|
X. R. Mao, Stability of stochastic differential equations with Markovian switching, Stoch. Proc. Appl., 1999, 79, 45–67.
Google Scholar
|
|
[20]
|
X. R. Mao Exponential stability of stochastic delay interval systems with Markovian switching, IEEE Trans. Automat. Contr., 2002, 47(10), 1604–1612.
Google Scholar
|
|
[21]
|
X. R. Mao and C. G. Yuan, Stochastic differential equations with Markovian switching, Imperial College Press, 2006.
Google Scholar
|
|
[22]
|
X. R. Mao, Stabilization of continuous-time hybrid stochastic differential equations by discrete-time feedback control, Automatics, 2013, 49(12), 3677–3681.
Google Scholar
|
|
[23]
|
X. R. Mao, W. Liu, L. J, Hu, Q. Luo and J. Q. Lu, Stabilization of hybrid stochastic differential equations by feedback control based on discrete-time state observations, Syst. Control Lett., 2014, 73, 88–95.
Google Scholar
|
|
[24]
|
S. Y. Pan, Z. Y. Ye and J. Zhou, $H_{\infty}$ robust control based on event-triggered sampling for hybrid systems with singular Markovian jump, Math. Mett. Appl. Sci., 2019, 42, 790–805.
Google Scholar
|
|
[25]
|
B. Pang and Q. L. Zhang, Sliding mode control for polynomial fuzzy singular systems with time delay, IET Control Theory A., 2018, 12(10), 1483–1490.
Google Scholar
|
|
[26]
|
W. H. Qi, G. D. Zong and H. R. Karim, Observer-based adaptive SMC for nonlinear uncertain singular semi-Markov jump systems with applications to DC motor, IEEE Trans. Circuits Syst. I. Reg. Papers, 2018, 65(9), 2951–2960.
Google Scholar
|
|
[27]
|
R. Shorthen, F.Wirth, O. Mason, K. Wulff and C. King, Stability criteria for switched and hybrid systems, Siam Rev., 2007, 49(4), 545–592.
Google Scholar
|
|
[28]
|
M. H. Sun, J. Lam, S. Y. Xu and Y. Zou, Robust exponential stabilization for Markovian jump systems with mode-dependent input delay, Automatica, 2007, 43, 1799–1807.
Google Scholar
|
|
[29]
|
H. J. Wang, A. K. Xue and R. Q. Lu, New stability criteria for singular systems with time-varying delay and nonlinear perturbations, Int. J. Syst. Sci., 2014, 45(12), 2576–2589.
Google Scholar
|
|
[30]
|
G. L. Wang, Q. L. Zhang and C. Y. Yang, Exponential stability of stochastic singular delay systems with general Markovian switchings, Int. J. Robust Nonlin., 2014, 25(17), 3478–3494.
Google Scholar
|
|
[31]
|
J. M. Wang, S. P. Ma, C. H. Zhang and M. Y. Fu, Finite-time $H_{\infty}$ filtering for nonlinear singular systems with nonhomogeneous Markov jumps, IEEE T. Cybernetics, 2018, 49(6), 2133–2143.
Google Scholar
|
|
[32]
|
Z. G. Wu, H. Y. Su and J. Chu, Robust exponential stability of uncertain singular Markovian jump time-delay systems, Acta Automatica Sinica, 2010, 36(4), 558–563.
Google Scholar
|
|
[33]
|
Z. G. Wu, H. Y. Su and J. Chu, $\text{H}_{\infty}$ filtering for singular Markovian jump systems with time delay, Int. J. Robust Nonlin., 2010, 20(8), 939–957.
Google Scholar
|
|
[34]
|
Y. F. Xie, W. H. Gui, Y. L. Wang and Z. H. Jiang, Memory state feedback controller design for singular systems with multiple internal constant point delays, IET Control Theory A., 2009, 3(6), 631–641.
Google Scholar
|
|
[35]
|
J. Xie, Y. G. Kao, C. H. Zhang and H. R. Karimi, Quantized control for uncertain singular Markovian jump linear systems with general incomplete transition rates, Int. J. Control Autom. Syst., 2017, 15(3), 1107–1116.
Google Scholar
|
|
[36]
|
S. Y. Xing, F. Q. Deng and D. Hou, Delay-dependent $H_{\infty}$ filtering for singular Markov jump systems with wiener process and generally uncertain transition rates, Int. J. Syst. Sci., 2018, 49(8), 1685–1702.
Google Scholar
|
|
[37]
|
S. Y. Xu, T. Chen and J. Lam, Robust H$_{\infty}$ filtering for uncertain Markovian jump systems with mode-dependent time delays, IEEE Trans. Automat. Contr., 2003, 48(5), 900–907.
Google Scholar
|
|
[38]
|
S. Y. Xu and J. Lam, Reduced-order $H_{\infty}$ filter for singular systems, Syst. Control Lett., 2007, 56(1), 48–57.
Google Scholar
|
|
[39]
|
P. Yu, M. A. Han and Y. Z. Bai, Dynamics and bifurcation stidy on an extended lorenz system, J. nonlinear model. anal., 2019, 1(1), 107–128.
Google Scholar
|
|
[40]
|
L. X. Zhang, B. Cai and Y. Shi, Stabilization of hidden semi-Markov jump systems: Emission probability approach, Automatica, 2019, 101, 87–95.
Google Scholar
|
|
[41]
|
J. Zuo, G. B. Liu, Y. L. Wei, Z. D. Wei and J. W. Feng, Mixed filter design for nonlinear singular Markovian jump systems with time-varying delays based on a dissipativity performance index, T. I. Meas. Control, 2018, 40(9), 2779–2788.
Google Scholar
|




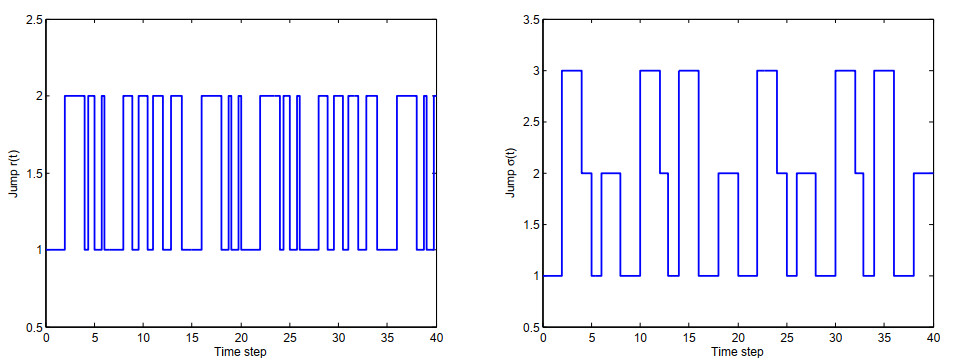

 DownLoad:
DownLoad: