COMPACT FINITE DIFFERENCE SCHEMES OF THE TIME FRACTIONAL BLACK-SCHOLES MODEL

Zhaowei Tian; Shuying Zhai; Zhifeng Weng

doi:10.11948/20190148

2020 Volume 10 Issue 3

Article Contents

Article navigation > Journal of Applied Analysis & Computation > 2020 > 10(3): 904-919

Next Article Previous Article

Zhaowei Tian, Shuying Zhai, Zhifeng Weng. COMPACT FINITE DIFFERENCE SCHEMES OF THE TIME FRACTIONAL BLACK-SCHOLES MODEL[J]. Journal of Applied Analysis & Computation, 2020, 10(3): 904-919. doi: 10.11948/20190148

Citation:

Zhaowei Tian, Shuying Zhai, Zhifeng Weng. COMPACT FINITE DIFFERENCE SCHEMES OF THE TIME FRACTIONAL BLACK-SCHOLES MODEL[J]. Journal of Applied Analysis & Computation, 2020, 10(3): 904-919. doi: 10.11948/20190148

COMPACT FINITE DIFFERENCE SCHEMES OF THE TIME FRACTIONAL BLACK-SCHOLES MODEL

1.
School of Mathematical Sciences, Huaqiao University, Quanzhou 362021, Fujian, China

Corresponding author: Email address:zfwmath@163.com(Z. Weng)
Fund Project: The authors were in part supported by the National Natural Science Foundation of China (Nos. 11701197 and 11701196), the Fundamental Research Funds for the Central Universities (No. ZQN-702), the Promotion Program for Young and Middle-aged Teacher in Science and Technology Research of Huaqiao University(No. ZQN-YX502), and the Project of Education and Scientific Research for Young and Middle-aged Teachers in Fujian Province(No. JAT160024)

Abstract

Abstract

In this paper, three compact difference schemes for the time-fractio-nal Black-Scholes model governing European option pricing are presented. Firstly, in order to obtain the fourth-order accuracy in space by applying the Padé approximation, we eliminate the convection term of the B-S equation by an exponential transformation. Then the time fractional derivative is approximated by $ L1 $ formula, $ L2 - 1_\sigma $ formula and $ L1 - 2 $ formula respectively, and three compact difference schemes with oders $ O(\Delta t^{2-\alpha}+h ^4) $, $ O(\Delta t^{2}+h ^4) $ and $ O(\Delta t^{3-\alpha}+h ^4) $ are constructed. Finally, numerical example is carried out to verify the accuracy and effectiveness of proposed methods, and the comparisons of various schemes are given. The paper also provides numerical studies including the effect of fractional orders and the effect of different parameters on option price in time-fractional B-S model.
- Time-fractional Black-Scholes equation /
- European option /
- exponential transformation /
- compact difference scheme

FullText(HTML)

References

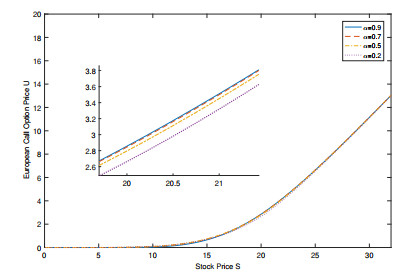
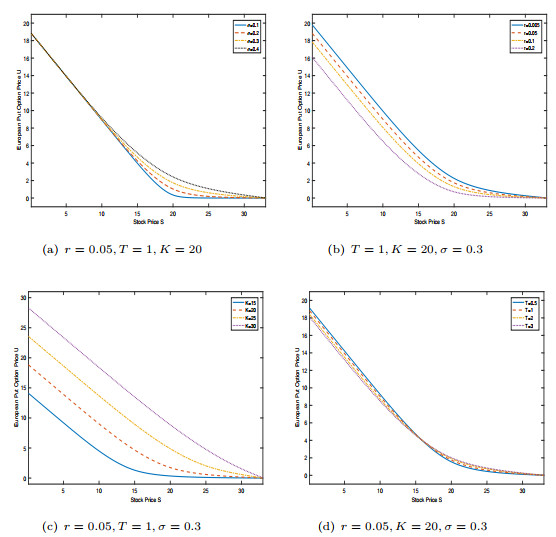
[1]	A. A. Alikhanov, A new difference scheme for the time fractional diffusion equation, J. Comput. Phys., 2015, 280, 424-438. doi: 10.1016/j.jcp.2014.09.031 CrossRef Google Scholar
[2]	F. Black, M. Scholes, The pricing of options and corporate liabilities, J. Polit. Econ., 1973, 81(3), 637-654. doi: 10.1086/260062 CrossRef Google Scholar
[3]	P. Carr, L. Wu, The finite moment log stable process and option pricing, J. Finance, 2003, 58(2), 597-626. Google Scholar
[4]	A. Cartea, D. del-Castillo-Negrete, Fractional diffusion models of option prices in markets with jumps, Phys. A: Stat. Mech. Appl., 2007, 374(2), 749-763. Google Scholar
[5]	W. T. Chen, X. Xu, S. P. Zhu, Analytically pricing European-style options under the modified Black-Scholes equation with a spatial-fractional derivative, Q. Appl. Math., 2014, 72(3), 597-611. doi: 10.1090/S0033-569X-2014-01373-2 CrossRef Google Scholar
[6]	A. Cartea, Derivatives pricing with market point processes using tick-by-tick data, Q. Finance, 2013, 13(1), 111-123. Google Scholar
[7]	W. T. Chen, X. Xu, S. P. Zhu, Analytically pricing double barrier options based on a time-fractional Black-Scholes equation, Comput. Math. Appl., 2015, 69(12), 1407-1419. doi: 10.1016/j.camwa.2015.03.025 CrossRef Google Scholar
[8]	W. T. Chen, B. W. Yan, G. H. Lian et al., Numerically pricing American options under the generalized mixed fractional Brownian motion model, Phys. A: Stat. Mech. Appl., 2016, 451, 180-189. doi: 10.1016/j.physa.2015.12.154 CrossRef Google Scholar
[9]	Z. D. Cen, J. Huang, A. M. Xu et al., Numerical approximation of a time-fractional Black-Scholes equation, Comput. Math. Appl., 2018, 8(75), 2874-2887. Google Scholar
[10]	G. H. Gao, Z. Z. Sun, H. W. Zhang, A new fractional numerical differentiation formula to approximate the Caputo fractional derivative and its applications, J. Comput. Phys., 2014, 259, 33-50. doi: 10.1016/j.jcp.2013.11.017 CrossRef Google Scholar
[11]	G. Jumarie, Derivation and solutions of some fractional Black-Scholes equations in coarse-grained space and time. Application to Merton's optimal portfolio, Comput. Math. Appl., 2010, 59(3), 1142-1164. doi: 10.1016/j.camwa.2009.05.015 CrossRef Google Scholar
[12]	M. N. Koleva, L. G. Vulkov, Numerical solution of time-fractional Black-Scholes equation, J. Comput. Appl. Math., 2017, 36 (4), 1699-1715. Google Scholar
[13]	J. R. Liang, J. Wang, W. J Zhang et al., The solutions to a bi-fractional Black-Scholes-Merton differential equation, Int. J. Pure Appl. Math., 2010, 58(1), 99-112. Google Scholar
[14]	W. Y. Liao, A compact high-order finite difference method for unsteady convection-diffusion equation, Int. J. Comput. Meth. Eng. Sci. Mech., 2012, 13(3), 135-145. Google Scholar
[15]	B. Mandelbrot, The variation of certain speculative prices, J. Bus. Univ. Chicago, 1963, 36, 394-419. Google Scholar
[16]	L. N. Song, W. G. Wang, Solution of the fractional Black-Scholes option pricing model by finite difference method, Abstr. Appl. Anal., 2013, Article ID 194286, 1-10. Google Scholar
[17]	R. H. De Staelen, A. S. Hendy, Numerically pricing double barrier options in a time-fractional Black-Scholes model, Comput. Math. Appl., 2017, 74, 1166-1175. doi: 10.1016/j.camwa.2017.06.005 CrossRef Google Scholar
[18]	Z. Z. Sun, G. H. Gao, Finite Difference Methods for Fractional Differential Equations, Science Press, Beijing, 2015. Google Scholar
[19]	W. Wyss, The fractional Black-Scholes equations, Fract. Calc. Appl. Anal., 2000, 3(1), 51-61. Google Scholar
[20]	X. Z. Yong, X. Zhang, L. F. Wu, A kind of efficient difference method for time-fractional option pricing model, Appl. Math. J. Chin. Univ., 2015, 30(2), 234-244. Google Scholar
[21]	X. Z. Yang, L. F. Wu, S. Z. Sun et al., A universal difference method for time-space fractional Black-Scholes equation, Adv. Differ. Equ., 2016, 71, 1-14. Google Scholar
[22]	H. M. Zhang, F. W. Liu, I. Turner et al., The numerical simulation of the tempered fractional Black-Scholes equation for European double barrier option, Appl. Math. Model., 2016, 40(11-12), 5819-5834. doi: 10.1016/j.apm.2016.01.027 CrossRef Google Scholar
[23]	H. M. Zhang, F. W. Liu, I. Turner et al., Numerical solution of the time fractional Black-Scholes model governing European options, Comput. Math. Appl., 2016, 71(9), 1772-1783. doi: 10.1016/j.camwa.2016.02.007 CrossRef Google Scholar
[24]	Y. Zhang, X. Z. Yang, Pure alternative segment explicit-implicit parallel difference methods for time-fractional Black-Scholes equation, China Science Paper, 2017, 12(17), 1966-1971. Google Scholar
[25]	Z. Q. Zhou, X. M. Gao, Numerical methods for pricing American options with time-fractional PDE models, Math. Prob. Eng., 2016, Article ID 5614950, 1-8. Google Scholar