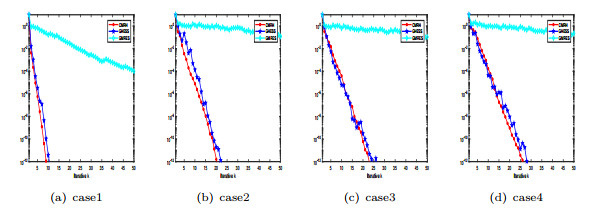
|
[1]
|
L. Amodei and J. M. Buchot, An invariant subspace method for large-scale algebraic Riccati equation, Appl. Numer. Math., 2010, 60, 1067-1082.
Google Scholar
|
|
[2]
|
A. C. Antoulas, Approximation of large-scale dynamical systems, SIAM, Philadelphia, 2005.https://www.researchgate.net/publication/243786519_Approximation_of_Large-Scale_Dynamical_Systems
Google Scholar
|
|
[3]
|
S. Agoujil, A. H. Bentbib, K. Jbilou, and E. M. Sadek, A minimal residual norm method for large-scale Sylvester matrix equations, Elect. Trans. Numer. Anal., 2014, 43, 45-59.
Google Scholar
|
|
[4]
|
L. Bao, Y. Lin and Y. Wei, A modified simple iterative method for nonsymmetric algebraic Riccati equations arising in transport theory, Appl. Math. Comput., 2006, 181(2), 1499-1504.
Google Scholar
|
|
[5]
|
P. Benner, J. Li and T. Penzl, Numerical solution of large-scale Lyapunov equations, Riccati equations, and linear-quadratic optimal control problems, Numer. Linear Algebra. Appl., 2008, 15, 755-777.
Google Scholar
|
|
[6]
|
J. P. Chehab and M. Raydan, Inexact Newton's method with inner implicit preconditioning for algebraic Riccati equations, Comp. Appl. Math., 2017, 36, 955-969. DOI10.1007/s40314-015-0274-8.
Google Scholar
|
|
[7]
|
E. Chu, H. Fan, W. Lin and C. Wang, Structure-preserving doubling algorithms for periodic discrete-time algebraic Riccati equations, Int. J. Control, 2004, 77, 767-788.
Google Scholar
|
|
[8]
|
D. Chu, W. Lin and R. Tan, A Numerical Method for a Generalized Algebraic Riccati Equation, SIAM J. Control Optim., 2006, 45(4), 1222-1250.
Google Scholar
|
|
[9]
|
B. N. Datta, Numeritcal methods for linear control systems design and analysis, Elservier Press, Amsterdam, 2003.
Google Scholar
|
|
[10]
|
R. Freund, G. H. Golub and N. M. Nachtigal, Iterative solution of linear systems, Acta Numer., 1992, 1, 57-100.
Google Scholar
|
|
[11]
|
X. Guo, W. Lin and S. Xu, A structure-preserving doubling algorithm for nonsymmetric algebraic Riccati equation, Numer. Math., 2006, 103, 393-412.
Google Scholar
|
|
[12]
|
M. Heyouni and H. Sadok, A new implementation of the CMRH method for solving dense linear systems, J. Comput. Appl. Math., 2008, 213(2), 387-399.
Google Scholar
|
|
[13]
|
K. Jbilou, Block Krylov subspace methods for large algebraic Riccati equations, Numer. Algorithms, 2003, 34, 339-353.
Google Scholar
|
|
[14]
|
K. Jbilou, Low rank approximate solutions to large Sylvester matrix equations, Appl. Math. Comput., 2006, 177(1), 365-376.
Google Scholar
|
|
[15]
|
K. Jbilou, A. Messaoudi, and H. Sadok, Global FOM and GMRES algorithms for matrix equations, Appl. Numer. Math., 1999, 31(1), 49-63.
Google Scholar
|
|
[16]
|
J. Juang, Existence of algebraic matrix Riccati equations arising in transport theory, Linear Algebra Appl., 1995, 230, 89-100.
Google Scholar
|
|
[17]
|
L. Lu, Solution form and simple iteration of a nonsymmetric algebraic Riccati equation arising in transport theory, SIAM J. Matrix Anal. Appl. 2005, 26, 679-685.https://www.researchgate.net/publication/220656505_Solution_Form_and_Simple_Iteration_of_a_Nonsymmetric_Algebraic_Riccati_Equation_Arising_in_Transport_Theory
Google Scholar
|
|
[18]
|
Matrix Market. http://math.nist.gov/MatrixMarket/.
Google Scholar
|
|
[19]
|
V. Mehrmann, The autonomous linear quadratic control problem, theory and numerical solution. Lecture Notes in Control and Information Sciences, Springer, Berlin, 1991, 163.
Google Scholar
|
|
[20]
|
T. Penzl, LYAPACK: A MATLAB toolbox for large Lyapunov and Riccati equations, model reduction problems, and linear-quadratic optimal control problems, users guide, 2000, 1(0). Available at: www.tu-chemnitz.de/sfb393/lyapack.
Google Scholar
|
|
[21]
|
H. Sadok, CMRH: A new method for solving nonsymmetric linear systems based on the Hessenberg reduction algorithm, Numer. Algorithm, 1999, 20, 303-321.
Google Scholar
|
|
[22]
|
H. Sadok and D. B. Szyld, A new look at CMRH and its relation to GMRES, BIT Numer. Math., 2012, 52(2), 485-501.
Google Scholar
|
|
[23]
|
N. Sandell, On Newton's method for Riccati equation solution, IEEE Trans. Auto. Control, 1974, 19, 254-255.
Google Scholar
|
|
[24]
|
V. Simoncini, On two numerical methods for the solution of large-scale algebraic Riccati equations, IMA J. Numer. Anal., 2013, 1-17.https://www.researchgate.net/publication/265480867_On_two_numerical_methods_for_the_solution_of_large-scale_algebraic_Riccati_equations
Google Scholar
|
|
[25]
|
K. Zhang and C. Gu, Flexible global generalized Hessenberg methods for linear systems with multiple right-hand sides, J. Comput. Appl. Math, 2014, 263, 312-325.
Google Scholar
|




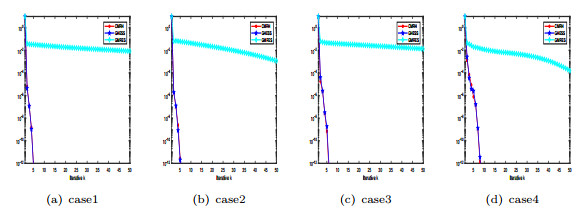

 DownLoad:
DownLoad: