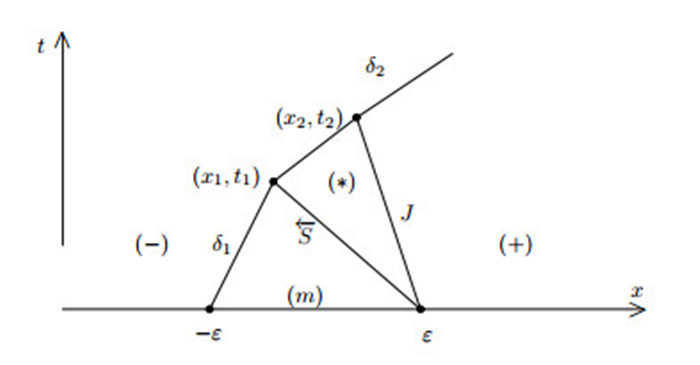
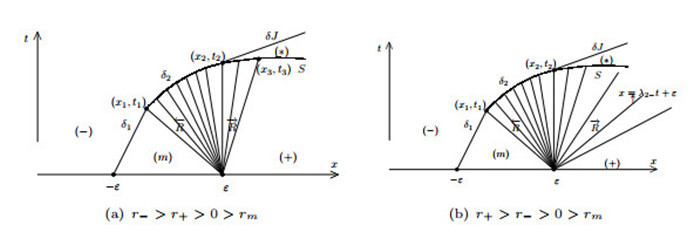
|
[1]
|
Y. Brenier, Solutions with concentration to the Riemann problem for one-dimensional Chaplygin gas dynamics, J. Math. Fluid Mech., 2005, 7, S326–S331. doi: 10.1007/s00021-005-0162-x
CrossRef Google Scholar
|
|
[2]
|
Y. Brenier and E. Grenier, Sticky particles and scalar conservation laws, SIAM J. Numer. Anal., 1998, 35(6), 2317–2328. doi: 10.1137/S0036142997317353
CrossRef Google Scholar
|
|
[3]
|
A. Bressan, Hyperbolic systems of conservation laws: the one-dimensional Cauchy problem, Oxford Lecture Ser. Math. Appl., Vol. 20. Oxford University Press, Oxford, 2000.
Google Scholar
|
|
[4]
|
G. Chen and H. Liu, Formation of delta-shocks and vacuum states in the vanishing pressure limit of solutions to the isentropic Euler equations, SIAM J. Math. Anal., 2003, 34(4), 925–938. doi: 10.1137/S0036141001399350
CrossRef Google Scholar
|
|
[5]
|
H. Cheng and H. Yang, Delta shock waves in chromatography equations, J. Math. Anal. Appl., 2011, 380(2), 475–485. doi: 10.1016/j.jmaa.2011.04.002
CrossRef Google Scholar
|
|
[6]
|
V. G. Danilov and D. Mitrovic, Delta shock wave formation in the case of triangular hyperbolic system of conservation laws, J. Differential Equations, 2008, 245(12), 3704–3734.
Google Scholar
|
|
[7]
|
B. Engquist and O. Runborg, Multiphase computations in geometrical optics, J. Comput. Appl. Math., 1996, 74, 175–192. doi: 10.1016/0377-0427(96)00023-4
CrossRef Google Scholar
|
|
[8]
|
P. Le Floch, An existence and uniqueness result for two nonstrictly hyperbolic systems, IMA Vol. Math. Appl., Vol. 27. Springer-Verlag, Berlin/New York, 1990.
Google Scholar
|
|
[9]
|
L. Guo, L. Pan and G. Yin, The perturbed Riemann problem and delta contact discontinuity in chromatography equations, Nonlinear Analysis, 2014, 106, 110–123. doi: 10.1016/j.na.2014.04.016
CrossRef Google Scholar
|
|
[10]
|
L. Guo, Y. Zhang and G. Yin, Interactions of delta shock waves for the Chaplygin gas equations with split delta functions, J. Math. Anal. Appl., 2014, 410(1), 190–201. doi: 10.1016/j.jmaa.2013.07.082
CrossRef Google Scholar
|
|
[11]
|
F. Huang and Z Wang, Well-posedness for pressureless flow, Commun. Math. Phys., 2001, 222(1), 117–146.
Google Scholar
|
|
[12]
|
B. L. Keyfitz and H. C. Kranzer, A viscosity approximation to a system of conservation laws with no classical Riemann solution, Lecture Notes in Math., Vol. 1402. Springer-Verlag, Berlin-New York, 1989.
Google Scholar
|
|
[13]
|
D. J. Korchinski, Solution of a Riemann problem for a $2\times2 $ system of conservation laws possessing no classical weak solution, thesis, Adelphi University, 1977.
Google Scholar
|
|
[14]
|
H. C. Kranzer and B. L. Keyfitz, A strictly hyperbolic system of conservation laws admitting singular shock, IMA Vol. Math. Appl., Vol. 27. Springer-Verlag, Berlin-New York, 1990.
Google Scholar
|
|
[15]
|
M. Nedeljkov, Delta and singular delta locus for one dimensional systems of conservation laws, Math. Methods Appl. Sci., 2004, 27(8), 931–955. doi: 10.1002/mma.480
CrossRef Google Scholar
|
|
[16]
|
M. Nedeljkov and M. Oberguggenberger, Interactions of delta shock waves in a strictly hyperbolic system of conservation laws, J. Math. Anal. Appl., 2008, 344(2), 1143–1157. doi: 10.1016/j.jmaa.2008.03.040
CrossRef Google Scholar
|
|
[17]
|
B. Nilsson, O. S. Rozanova and V. M. Shelkovich, Mass, momentum and energy conservation laws in zero-pressure gas dynamics and $\delta $-shocks, Ⅱ, Appl. Anal., 2011, 90(5), 831–842. doi: 10.1080/00036811.2010.524156
CrossRef $\delta $-shocks" target="_blank">Google Scholar
|
|
[18]
|
B. Nilsson and V. M. Shelkovich, Mass, momentum and energy conservation laws in zero-pressure gas dynamics and delta-shocks, Appl. Anal., 2011, 90(11), 1677–1689. doi: 10.1080/00036810903569515
CrossRef Google Scholar
|
|
[19]
|
Y. Pang, Delta shock wave in the compressible Euler equations for a Chaplygin gas, J. Math. Anal. Appl., 2017, 448(1), 245–261. doi: 10.1016/j.jmaa.2016.10.078
CrossRef Google Scholar
|
|
[20]
|
E. Y. Panov and V. M. Shelkovich, $\delta'-$shock waves as a new type of solutions to systems of conservation laws, J. Differential Equations, 2006, 73(1), 49-86.
Google Scholar
|
|
[21]
|
A. Sen, T. R. Sekhar and V. D. Sharma, Wave interactions and stability of the Riemann solution for a strictly hyperbolic system of conservation laws, Q. Appl. Math., 2017, 75(3), 539–554. doi: 10.1090/qam/1466
CrossRef Google Scholar
|
|
[22]
|
S. F. Shandarin and Y. B. Zeldovich, The large-scale structure of the universe: turbulence, intermittency, structure in a selfgravitating medium, Rev. Modern Phys., 1989, 61(2), 185–220. doi: 10.1103/RevModPhys.61.185
CrossRef Google Scholar
|
|
[23]
|
C. Shen and M. Sun, Stability of the Riemann solutions for a nonstrictly hyperbolic system of conservation laws, Nonlinear Analysis, 2010, 73(10), 3284–3294. doi: 10.1016/j.na.2010.07.008
CrossRef Google Scholar
|
|
[24]
|
W. Sheng and T. Zhang, The Riemann problem for transportation equation in gas dynamics, Mem. Am. Math. Soc., 1999, 137, 1–77.
Google Scholar
|
|
[25]
|
M. Sun, Delta shock waves for the chromatography equations as self-similar viscosity limits, Q. Appl. Math., 2011, 69(3), 425–443. doi: 10.1090/S0033-569X-2011-01207-3
CrossRef Google Scholar
|
|
[26]
|
M. Sun, Interactions of delta shock waves for the chromatography equations, Appl. Math. Lett., 2013, 26(6), 631–637. doi: 10.1016/j.aml.2013.01.002
CrossRef Google Scholar
|
|
[27]
|
D. Tan and T. Zhang, Two-dimensional Riemann problem for a hyperbolic system of nonlinear conservation laws, I. Four-J cases, Ⅱ. Initial data involving some rarefaction waves, J. Differential Equations, 1994, 111(2), 203–253. doi: 10.1006/jdeq.1994.1081
CrossRef Google Scholar
|
|
[28]
|
D. Tan, T. Zhang and Y. Zheng, Delta shock waves as limits of vanishing viscosity for hyperbolic systems of conversation laws, J. Differential Equations, 1994, 112(1), 1–32.
Google Scholar
|
|
[29]
|
G. Wang, One-dimensional nonlinear chromatography system and delta-shock waves, Z. Angew. Math. Phys., 2013, 64(5), 1451–1469. doi: 10.1007/s00033-013-0300-x
CrossRef Google Scholar
|
|
[30]
|
E. Weinan, Yu. G. Rykov and Ya. G. Sinai, Generalized variational principles, global weak solutions and behavior with random initial data for systems of conservation laws arising in ashesion particle dynamics, Comm. Math. Phys., 1996, 177(2), 349–380. doi: 10.1007/BF02101897
CrossRef Google Scholar
|
|
[31]
|
H. Yang, Riemann problems for a class of coupled hyperbolic systems of conservation laws, J. Differential Equations, 1999, 159, 447–484. doi: 10.1006/jdeq.1999.3629
CrossRef Google Scholar
|
|
[32]
|
H. Yang and Y. Zhang, New developments of delta shock waves and its applications in systems of conservation laws, J. Differential Equations, 2012, 252(11), 5951–5993. doi: 10.1016/j.jde.2012.02.015
CrossRef Google Scholar
|
|
[33]
|
H. Yang and Y. Zhang, Delta shock waves with Dirac delta function in both components for systems of conservation laws, J. Differential Equations, 2014, 257(12), 4369–4402. doi: 10.1016/j.jde.2014.08.009
CrossRef Google Scholar
|
|
[34]
|
Q. Zhang, Interactions of delta shock waves and stability of Riemann solutions for nonlinear chromatography equations, Z. Angew. Math. Phys., 2016, 67(1), 1451–1469.
Google Scholar
|
|
[35]
|
Y. Zhang and Y. Zhang, The Riemann problem and interaction of waves in two-dimensional steady zero-pressure adiabatic flow, Internat. J. Non-Linear Mech., 2018, 104, 100–108. doi: 10.1016/j.ijnonlinmec.2018.05.010
CrossRef Google Scholar
|
|
[36]
|
Y. Zhang and Y. Zhang and J. Wang, Interaction of delta shock waves for the Chaplygin Euler equations of compressible fluid flow with split delta functions, Math. Meth. Appl. Sci., 2018, 41(3), 7678–7697.
Google Scholar
|




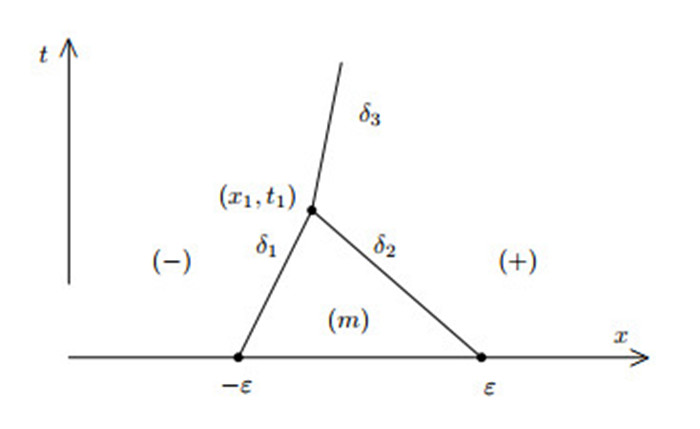

 DownLoad:
DownLoad: