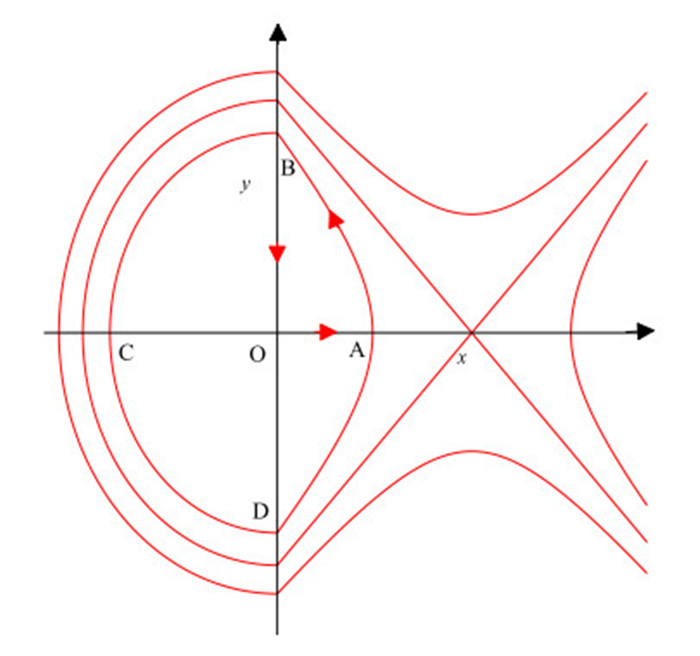
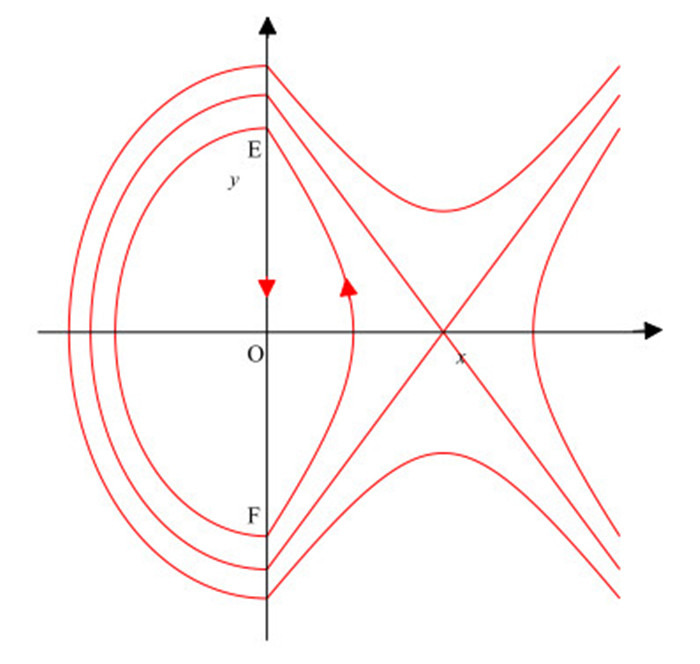
|
[1]
|
X. Cen, C. Liu, L. Yang and M. Zhang, Limit cycles by perturbing quadratic isochronous centers inside piecewise polynomial differential systems, J. Diff. Eqs., 2018, 265, 6083–6126.
Google Scholar
|
|
[2]
|
X. Cen, S. Li and Y. Zhao, On the number of limit cycles for a class of discontinuous quadratic differetnial systems, J. Math. Anal. Appl., 2017, 449, 314–342. doi: 10.1016/j.jmaa.2016.11.033
CrossRef Google Scholar
|
|
[3]
|
X. Chen, J. Llibre and W. Zhang, Averaging approach to cyclicity of Hopf bifurcation in planar lineat-quadratic polynomial discontunuous differential systems, Discrete Continuous Dynamical Systems Series B, 2017, 22, 3953–3965. doi: 10.3934/dcdsb.2017203
CrossRef Google Scholar
|
|
[4]
|
B. Coll, A. Gasull and R. Prohens, Degenerate Hopf bifurcation in discontinuous planar systems, J. Math. Anal. Appl., 2001, 253, 671–690. doi: 10.1006/jmaa.2000.7188
CrossRef Google Scholar
|
|
[5]
|
G. Dong and C. Liu, Note on limit cycles for m-piecewise discontinuous polynomial Liénard differential equations, Z. Angew. Math. Phys., 2017, 68, 97, 8pp. doi: 10.1007/s00033-017-0844-2
CrossRef Google Scholar
|
|
[6]
|
F. Filippov, Differential equations with discontinuous righthand sides, Kluwer Academic, Netherlands, 1988.
Google Scholar
|
|
[7]
|
Y. Gao, L. Peng and C. Liu, Bifurcation of limit cycles from a class of piecewise smooth systems with two vertical straight lines of singularity, Int. J. Bifur. Chaos, 2017, 27, 1750157 (13 pages).
Google Scholar
|
|
[8]
|
M. Han, On the maximum number of periodic solutions of piecewise smooth periodic equations by average method, J. Appl. Anal. Comput., 2017, 7, 788–794.
Google Scholar
|
|
[9]
|
M. Han and L. Sheng, Bifurcation of limit cycles in piecewise smooth systems via Melnikov function, J. Appl. Anal. Comput., 2015, 5, 809–815.
Google Scholar
|
|
[10]
|
M. Han and W. Zhang, On Hopf bifurcation in non-smooth planar systems, J. Diff. Eqs., 2010, 248, 2399–2416. doi: 10.1016/j.jde.2009.10.002
CrossRef Google Scholar
|
|
[11]
|
N. Hu and Z. Du, Bifurcation of periodic orbits emanated from a vertex in discontinuous planar systems, Commun. Nonlinear Sci. Numer. Simulat., 2013, 18, 3436–3448.
Google Scholar
|
|
[12]
|
J. Itikawa, J. Llibre, A. Mereu and R. Oliveira, Limit cycles in uniform isochronous centers of discontinuous differential systems with four zones, Discrete and Continuous Dynamical Systems Series B, 2017, 22, 3259–3272. doi: 10.3934/dcdsb.2017136
CrossRef Google Scholar
|
|
[13]
|
M. Kukucka, Non-smooth dynamical systems, Springer-Verlag, Berlin, Heidelberg, 2000.
Google Scholar
|
|
[14]
|
S. Li and C. Liu, A linear estimate of the number of limit cycles for some planar piecewise smooth quadratic differential system, J. Math. Anal. Appl., 2015, 428, 1354–1367.
Google Scholar
|
|
[15]
|
F. Liang, M. Han and V. Romanovski, Bifurcation of limit cycles by perturbing a piecewise linear Hamiltonian system with a homoclinic loop, Nonlinear Anal., 2012, 75, 4355–4374. doi: 10.1016/j.na.2012.03.022
CrossRef Google Scholar
|
|
[16]
|
Y. Liu and V. Romanovski, Limit cycle bifurcations in a class of piecewise smooth systems with a double homoclinic loop, Appl. Math. Comput., 2014, 248, 235–245.
Google Scholar
|
|
[17]
|
X. Liu and M. Han, Bifurcation of limit cycles by perturbing piecewise Hamiltonian systems, Internat. J. Bifur. Chaos Appl. Sci. Engrg, 2010, 20, 1379–1390. doi: 10.1142/S021812741002654X
CrossRef Google Scholar
|
|
[18]
|
J. Llibre and A. Mereu, Limit cycles for discontinuous quadratic differetnial systems, J. Math. Anal. Appl., 2014, 413, 763–775. doi: 10.1016/j.jmaa.2013.12.031
CrossRef Google Scholar
|
|
[19]
|
J. Llibre, A. Mereu and D. Novaes, Averaging theory for discontinuous piecewise differential systems, J. Diff. Eqs., 2015, 258, 4007–4032. doi: 10.1016/j.jde.2015.01.022
CrossRef Google Scholar
|
|
[20]
|
J. Llibre, D. Novaes and M. Teixeira, On the birth of limit cycles for non-smooth dynamical systems, Bull. Sci. Math., 2015, 139, 229–244. doi: 10.1016/j.bulsci.2014.08.011
CrossRef Google Scholar
|
|
[21]
|
J. Llibre and M. Teixerira, Limit cycles for m-piecewise discontinuous polynomial Liénard differential equations, Z. Angew. Math. Phys., 2015, 66, 51–66. doi: 10.1007/s00033-013-0393-2
CrossRef Google Scholar
|
|
[22]
|
Y. Wang, M. Han and D. Constantinescu, On the limit cycles of perturbed discontinuous planar systems with 4 switching lines, Chaos Solitons Fractals, 2016, 83, 158–177. doi: 10.1016/j.chaos.2015.11.041
CrossRef Google Scholar
|
|
[23]
|
L. Wei, F. Liang and S. Lu, Limit cycle bifurcations near a generalized homoclinic loop in piecewise smooth systems with a hyperbolic saddle on a switch line, Appl. Math. Comput., 2014, 243, 298–310.
Google Scholar
|
|
[24]
|
Y. Xiong, Limit cycle bifurcations by perturbing piecewise smooth Hamiltonian systems with multiple parameters, J. Math. Anal. Appl., 2015, 421, 260–275. doi: 10.1016/j.jmaa.2014.07.013
CrossRef Google Scholar
|
|
[25]
|
Y. Xiong, Limit cycle bifurcations by perturbing non-smooth Hamiltonian systems with 4 switching lines via multiple parameters, Nonlinear Analysis: Real World Applications, 2018, 41, 384–400. doi: 10.1016/j.nonrwa.2017.10.020
CrossRef Google Scholar
|
|
[26]
|
Y. Xiong and J. Hu, Limit cycle bifurcations in perturbations of planar piecewise smooth systems with multiply lines of critical points, J. Math. Anal. Appl., 2019, 474, 194–218. doi: 10.1016/j.jmaa.2019.01.039
CrossRef Google Scholar
|
|
[27]
|
J. Yang and L. Zhao, Limit cycle bifurcations for piecewise smooth Hamiltonian systems with a generalized eye-figure loop, Int. J. Bifur. Chaos, 2016, 26, 1650204(14pages).
Google Scholar
|
|
[28]
|
J. Yang and L. Zhao, Limit cycle bifurcations for piecewise smooth integrable differential systems, Discrete and Continuous Dynamical Systems Series B, 2017, 22, 2417–2425. doi: 10.3934/dcdsb.2017123
CrossRef Google Scholar
|
|
[29]
|
J. Yang and L. Zhao, Bounding the number of limit cycles of discontinuous differential systems by using Picard-Fuchs equations, J. Diff. Eqs., 2018, 264, 5734–5757. doi: 10.1016/j.jde.2018.01.017
CrossRef Google Scholar
|




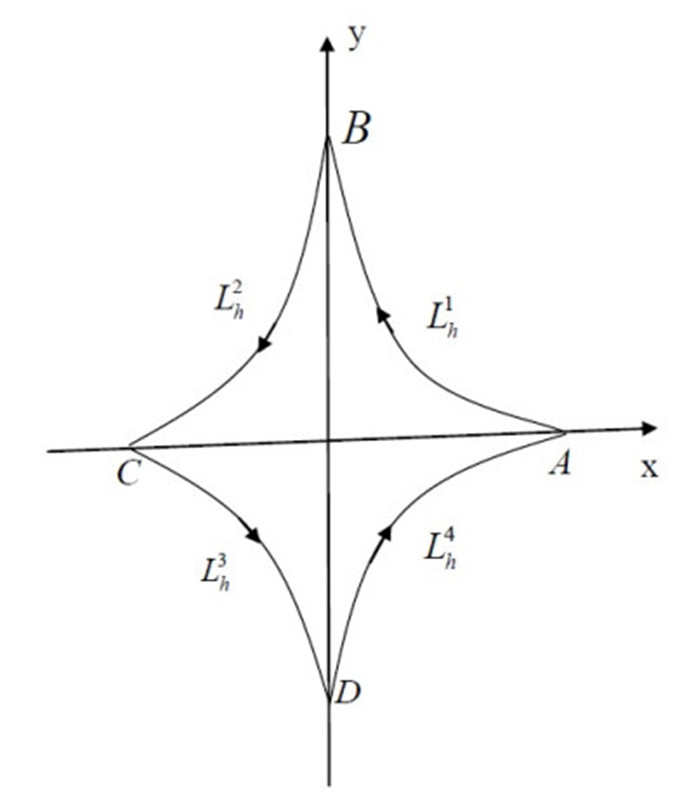

 DownLoad:
DownLoad: