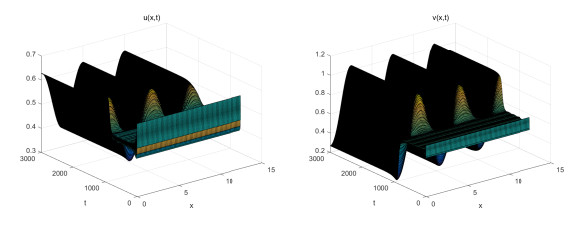
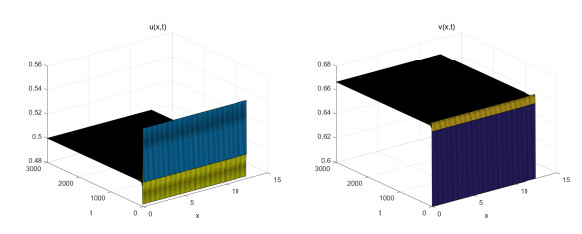
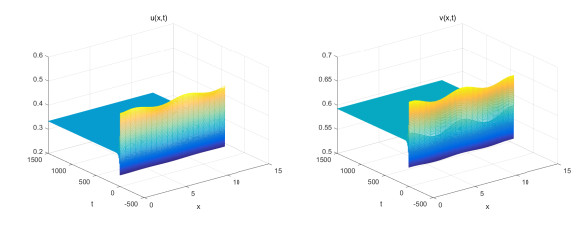
|
[1]
|
E. Beretta, S. Fortunata and T. Yasuhiro, Global stability and periodic orbits for two-patch predator-prey diffusion-delay models, Mathematical Biosciences, 1987, 85(2), 153-183. doi: 10.1016/0025-5564(87)90051-4
CrossRef Google Scholar
|
|
[2]
|
X. Cao and W. Jiang, Interactions of Turing and Hopf bifurcations in an additional food provided diffusive predator-prey model, Journal of Applied Analysis and Computation, 2019, 9(4), 1277-1304. doi: 10.11948/2156-907X.20180224
CrossRef Google Scholar
|
|
[3]
|
C. Cao and W. Jiang, Turing-Hopf bifurcation and spatiotemporal patterns in a diffusive predator-prey system with Crowley-Martin functional response, Nonlinear Analysis Real World Applications, 2018, 43, 428-450. doi: 10.1016/j.nonrwa.2018.03.010
CrossRef Google Scholar
|
|
[4]
|
C. Cosner, D. Deangelis, J. Ault J and D. Olson, Effects of Spatial Grouping on the Functional Response of Predators, Theoretical Population Biology, 1999, 56(1), 65-75. doi: 10.1006/tpbi.1999.1414
CrossRef Google Scholar
|
|
[5]
|
Y. Dai, P. Yang, Z. Luo and Y. Lin, Bogdanov-Takens bifurcation in a delayed Michaelis-Menten type ratio-dependent predator-prey system with prey harvesting, 2019, 9(4), 1333-1346.
Google Scholar
|
|
[6]
|
S. Gourley, Instability in a predator-prey system with delay and spatial averaging, Ima Journal of Applied Mathematics, 1996, 56(2), 121-132. doi: 10.1093/imamat/56.2.121
CrossRef Google Scholar
|
|
[7]
|
B. Hassard, N. Kazarinoff and Y. Wan, Theory and applications of Hopf bifurcation, Cambridge University Press, Cambridge-New York, 1981.
Google Scholar
|
|
[8]
|
H. Jiang and X. Tang, Hopf bifurcation in a diffusive predator-prey model with herd behavior and prey harvesting, 2019 9(2), 671-690.
Google Scholar
|
|
[9]
|
R. Kimun, K. Wonlyul and H. Mainul, Bifurcation analysis in a predator-prey system with a functional response increasing in both predator and prey densities, Nonlinear Dynamics, 2018, 94(3), 1639-1656. doi: 10.1007/s11071-018-4446-0
CrossRef Google Scholar
|
|
[10]
|
A. Martin and S. Ruan, Predator-prey models with delay and prey harvesting, Journal of Mathematical Biology, 2001, 43(3), 247-267. doi: 10.1007/s002850100095
CrossRef Google Scholar
|
|
[11]
|
H. Shi and S. Ruan, Spatial, temporal and spatiotemporal patterns of diffusive predator-prey models with mutual interference, Ima Journal of Applied Mathematics, 2018, 80(5), 1534-1568.
Google Scholar
|
|
[12]
|
Y. Song, H. Jiang and Y. Yuan, Turing-Hopf bifurcation in the reaction-diffusion system with delay and application to a diffusive predator-prey model, Journal of Applied Analysis and Computation, 2019, 9(3), 1132-1164. doi: 10.11948/2156-907X.20190015
CrossRef Google Scholar
|
|
[13]
|
Y. Song, S. Wu and H. Wang, Spatiotemporal dynamics in the single population model with memory-based diffusion and nonlocal effect, Journal of Differential Equations, 2019, 267(11), 6316-6351. doi: 10.1016/j.jde.2019.06.025
CrossRef Google Scholar
|
|
[14]
|
J. Wang, J. Shi and J. Wei, Dynamics and pattern formation in a diffusive predator-prey system with strong Allee effect in prey, Journal of Differential Equations, 2011, 251(4), 1276-1304.
Google Scholar
|
|
[15]
|
J. Wu, Theory and Applications of Partial Functional Differential Equations. Springer Press, Berlin, 1996.
Google Scholar
|
|
[16]
|
F. Yi, J. Wei and J. Shi, Bifurcation and spatiotemporal patterns in a homogeneous diffusive predator-prey system, Journal of Differential Equations, 2009, 246(5), 1944-1977. doi: 10.1016/j.jde.2008.10.024
CrossRef Google Scholar
|




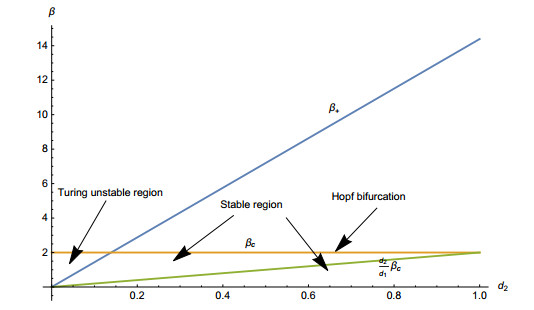

 DownLoad:
DownLoad: