2020 Volume 10 Issue 5
Article Contents
Article navigation > Journal of Applied Analysis & Computation > 2020 > 10(5): 2085-2103
Next Article Previous Article

Lizhi Fei, Lan Zou, Xingwu Chen. GLOBAL ANALYSIS FOR AN EPIDEMICAL MODEL OF VECTOR-BORNE PLANT VIRUSES WITH DISEASE RESISTANCE AND NONLINEAR INCIDENCE[J]. Journal of Applied Analysis & Computation, 2020, 10(5): 2085-2103. doi: 10.11948/20190329
Citation: Lizhi Fei, Lan Zou, Xingwu Chen. GLOBAL ANALYSIS FOR AN EPIDEMICAL MODEL OF VECTOR-BORNE PLANT VIRUSES WITH DISEASE RESISTANCE AND NONLINEAR INCIDENCE[J]. Journal of Applied Analysis & Computation, 2020, 10(5): 2085-2103. doi: 10.11948/20190329
shu

GLOBAL ANALYSIS FOR AN EPIDEMICAL MODEL OF VECTOR-BORNE PLANT VIRUSES WITH DISEASE RESISTANCE AND NONLINEAR INCIDENCE

  • 1.

    School of Mathematics, Sichuan University, Chengdu 610064, China

  • Corresponding author: Email: xingwu.chen@hotmail.com(X. Chen)
  • Fund Project: The authors were supported by NSFC(Nos. 11871355, 11831012, 11771168)
    Abstract
  • Vector-borne disease models play an important role in understanding the mechanism of plant disease transmission. In this paper, we study a vector-borne model with plant disease resistance, disease exposed period and nonlinear incidence. We compute the basic reproduction number, determine the implicit locations of equilibria and then investigate their global stability by generalizing a classic geometric approach to higher dimensional systems. Higher dimensions cause greater difficulties such as the construction of the transformation matrix and the estimate of the $Lozinski\tilde{\iota}$ measure in this geometric approach. For a complete control of vector-borne diseases, a quantitative way is provided by the given expression of the basic reproduction number, from which we need not only increasing plant disease resistance but also decreasing the contact rate between infected plants and susceptible vectors instead of a single one of them.
    • FullText(HTML)
  • 加载中
    • References
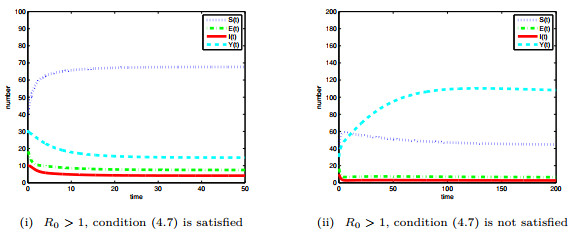
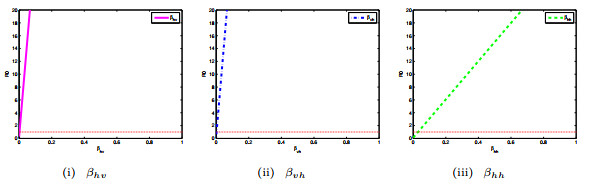
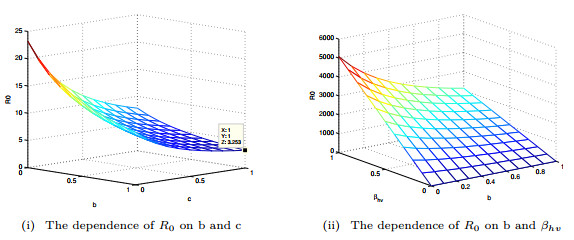
  • [1] L. Boiteux and L. Giordano, Genetic basis of resistance against two Tospovirus species in tomato (Lycopersicon esculentum), Euphytica, 1993, 71(1), 151-154.

    Google Scholar

    [2] C. Brittlebank, Tomato diseases, J. Depar. Agri. Vict., 1919, 17, 1348-1352.

    Google Scholar

    [3] G. Butler, H. Freedman and P. Waltman, Uniformly persistent systems, Proc. Amer. Math. Soc., 1986, 96(3), 425-430. doi: 10.1090/S0002-9939-1986-0822433-4

    CrossRef Google Scholar

    [4] K. Chen, Z. Xu, L. Yan and G. Wang, Characterization of a new strain of Capsicum chlorosis virus from peanut (Arachis hypogaea L.) in China, J. Phyt., 2007, 155(3), 178-181.

    Google Scholar

    [5] W. A. Coppel, Stability and Asymptotic Behavior of Differential Equations, Heath, Boston, 1965.

    Google Scholar

    [6] N. Cunniffe and C. Gilligan, Invasion, persistence and control in epidemic models for plant pathogens: the effect of host demography, J. Roy. Soc. Inter., 2010, 7(44), 439-451. doi: 10.1098/rsif.2009.0226

    CrossRef Google Scholar

    [7] A. Czech, M. Szklarczyk, Z. Gajewski, et al, Selection of tomato plants resistant to a local Polish isolate of tomato spotted wilt virus (TSWV), J. Appl. Genet., 2003, 44(4), 473-480.

    Google Scholar

    [8] O. Diekmann, J. Heesterbeek and J. Metz, On the definition and the computation of the basic reproduction ratio $R_{0}$ in models for infectious diseases in heterogeneous populations, J. Math. Biol., 1990, 28(4), 365-382.

    Google Scholar

    [9] M. Ding, Y. Luo, Q. Fang, Z. Zhang and Z. Zhao, First report of Groundnut yellow spot virus infecting Capsicum annuum in China, J. Plant Path., 2007, 89(2), 305.

    Google Scholar

    [10] P. Driessche and J. Watmough, Reproduction numbers and sub-threshold endemic equilibria for compartmental models of disease transmission, Math. Bios., 2002, 180(1), 29-48.

    Google Scholar

    [11] X. Feng, S. Ruan, Z. Teng and K. Wang, Stability and backward bifurcation in a malaria transmission model with applications to the control of malaria in China, Math. Bios., 2015, 266, 52-64. doi: 10.1016/j.mbs.2015.05.005

    CrossRef Google Scholar

    [12] L. Ferrand, M. M. S. Almeida, et al, Biological and molecular characterization of tomato spotted wilt virus (TSWV) resistance-breaking isolates from Argentina, Plant Pathology, 2019, 68(9), 1587-1601. doi: 10.1111/ppa.13087

    CrossRef Google Scholar

    [13] F. R. Gantmacher, The theory of matrices, Amer. Math. Soc., New York, 1959.

    Google Scholar

    [14] R. Gupta1, S. Kwon and S. Kim, An insight into the tomato spotted wilt virus (TSWV), tomato and thrips interaction, Plant Biot. Repo., 2018, 12(3), 157-163. doi: 10.1007/s11816-018-0483-x

    CrossRef Google Scholar

    [15] J. Hale, Asymptotic behavior of dissipative systems, Bull. Amer. Math. Soc., 1990, 22, 175-183. doi: 10.1090/S0273-0979-1990-15875-6

    CrossRef Google Scholar

    [16] M. Jeger, F. Van den Bosch and N. McRoberts, Modelling transmission characteristics and epidemic development of the tospovirus-thrip interaction, Arth.-Plant Interactions, 2015, 9(2), 107-120. doi: 10.1007/s11829-015-9363-2

    CrossRef Google Scholar

    [17] J. Jia and J. Xiao, Stability analysis of a disease resistance SEIRS model with nonlinear incidence rate, Adv. Diff. Equa., 2018, DOI: 10.1186/s13662-018-1494-1.

    CrossRef Google Scholar

    [18] Y. Kuang, D. Ben-Arieh, S. Zhao and C. Wu, Using spatial games to model and simulate tomato spotted wilt virus-western flowers thrip dynamic system, Int. J. Mode. Simu., 2018, 38(4), 243-253.

    Google Scholar

    [19] M. Li and J. Muldowney, On R.A. Smith's autonomous convergence theorem, Rock. moun. J. math., 1995, 25(1), 365-379. doi: 10.1216/rmjm/1181072289

    CrossRef Google Scholar

    [20] M. Li and J. Muldowney, A geometric approach to global stability problems, SIAM J. Math. Anal., 1996, 27(4), 1070-1083.

    Google Scholar

    [21] Y. Li, Z. Zhang, S. Guan, H. Pen, J. Li and Y. Zou, Kinds of tobacco viral pathogens and the infection cycle at Binchuan county, J. Yunnan Agri. Univ., 1997, 12(4), 263-268.

    Google Scholar

    [22] S. Morsello, A. Beaudoin, R. Groves, et al, The influence of temperature and precipitation on spring dispersal of Frankliniella fusca changes as the season progresses, Ento. Expe. Appl., 2010, 134(3), 260-271. doi: 10.1111/j.1570-7458.2009.00959.x

    CrossRef Google Scholar

    [23] R. Olatinwo, J. Paz, S. Brown, et al, A predictive model for spotted wilt epidemics in peanut based on local weather conditions and the tomato spotted wilt virus risk index, Ecol. Epid., 2008, 98(10), 1066-1074.

    Google Scholar

    [24] O. Pamella, P. Dany and P. Hans-Michael, Predictive Models for Tomato Spotted Wilt Virus Spread Dynamics, Considering Frankliniella occidentalis Specific Life Processes as Influenced by the Virus, PLoS ONE, 2016, 11(5), e0154533, (20 pages).

    Google Scholar

    [25] F. Riesz, Sur les Fonctions Subharmoniques et Leur Rapport 'a la Th¡äeorie du Potentiel, Acta. Math., 1930, 54(1), 321-360.

    Google Scholar

    [26] H. Robert and J. Martin, Logarithmic norms and projections applied to linear differential systems, J. Math. Anal. Appl., (1974), 45(2), 432-454. doi: 10.1016/0022-247X(74)90084-5

    CrossRef Google Scholar

    [27] G. Samuel, J. Bald and H. Pittman, Investigations on 'spotted wilt' of tomatoes, Aust. Coun. Scie. Indu. Rese. Bull., 1930, 44, 1-64.

    Google Scholar

    [28] A. Shaw, M. Igoe, et al, Modeling Approach Influences Dynamics of a Vector-Borne Pathogen System, Bull. Math. Biol., 2019, 81(6), 2011-2028. doi: 10.1007/s11538-019-00595-z

    CrossRef Google Scholar

    [29] R. Shi, H. Zhao and S. Tang, Global dynamic analysis of a vector-borne plant disease model, Adv. Diff. Equa., 2014, DOI: 10.1186/1687-1847-2014-59.

    CrossRef Google Scholar

    [30] H. Smith and P. Waltman, The Theory of the Chemostat: Dynamics of Microbial Competition, Cambridge University Press, Cambridge, 1995.

    Google Scholar

    [31] D. Su, X. Yuan, Y. Xie, S. Wang and H. Ding, Tomato spotted wilt virus in tomato in Chengdu and Dukou, Acta. Phyt. Sini., 1987, 17(4), 255-256.

    Google Scholar

    [32] H. Thieme, Persistence under relaxed point-dissipativity (with application to an endemic model), SIAM J. Math. Anal., 1993, 24(2), 407-435.

    Google Scholar

    [33] R. Varga, Iterative analysis, Prentice-Hall, Englewood Cliffs, NJ, 1962.

    Google Scholar

    [34] A. Whitfield, D. Ullman and T. German, Tospovirus-thrips interactions, Annu. Revi. Phyt., 2005, 43, 459-489. doi: 10.1146/annurev.phyto.43.040204.140017

    CrossRef Google Scholar

    [35] C. Wu, S. Zhao, Y. Kuang, et al, New mathematical models for vector-borne disease: transmission of tomato spotted wilt virus, Bridging research and good practices towards patient welfare, Taipei: CRC Press, 2014, 32, 259-268.

    Google Scholar

    [36] L. Xia, S. Gao, Q. Zou and J. Wang, Analysis of a nonautonomous plant disease model with latent period, Appl. Math. Comp., 2013, 223, 147-159. doi: 10.1016/j.amc.2013.08.011

    CrossRef Google Scholar

    [37] J. Ye, Y. Gong and R. Fang, Research progress and perspective of tripartite interaction of virus-vector plant in vector-borne viral diseases, Bull. Chin. Acad. Sci., 2017, 32(8), 845-855.

    Google Scholar

    [38] J. Zhang and Z. Ma, Global dynamics of an SEIR epidemic model with saturating contact rate, Math. Bios., 2003, 185(1), 15-32.

    Google Scholar

    • Supplements
    • Cited by

Figures(4)  /  Tables(1)

Export Citation
PDF
XML

Article Metrics

Article views(3431) PDF downloads(598) Cited by(0)

Access History

Other Articles By Authors

Catalog

    /

    DownLoad:  Full-Size Img  PowerPoint
    Return
    Published by Wilmington Scientific Publisher