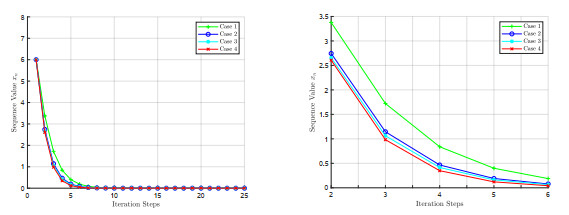
|
[1]
|
F. Alvarez and H. Attouch, An inertial proximal method for maximal monotone operators via discretization of a nonlinear oscillator with damping, Set-Valued Anal., 2001, 9(1-2), 3-11.
Google Scholar
|
|
[2]
|
C. Byrne, Iterative oblique projection onto convex sets and the split feasibility problem, Inverse Problems, 2002, 18(2), 441-453. doi: 10.1088/0266-5611/18/2/310
CrossRef Google Scholar
|
|
[3]
|
C. Byrne, Y. Censor, A. Gibali and S. Reich, The split common null point problem, J. Nonlinear Convex Anal., 2012, 13(4), 759-775.
Google Scholar
|
|
[4]
|
L. Ceng, A. Petruşel, C. Wen and J. C. Yao, Inertial-like subgradient extragradient methods for variational inequalities and fixed points of asymptotically nonexpansive and strictly pseudocontractive mappings, Mathematics, 2019, 7(9), 860. doi: 10.3390/math7090860
CrossRef Google Scholar
|
|
[5]
|
Y. Censor, T. Bortfeld, B. Martin and A. Trofimov, A unified approach for inversion problems in intensity-modulated radiation therapy, Phys. Med. Biol., 2006, 51(10), 2353-2365. doi: 10.1088/0031-9155/51/10/001
CrossRef Google Scholar
|
|
[6]
|
Y. Censor and T. Elfving, A multiprojection algorithm using Bregman projections in a product space, Numer. Algorithms, 1994, 8(2), 221-239. doi: 10.1007/BF02142692
CrossRef Google Scholar
|
|
[7]
|
Y. Censor, T. Elfving, N. Kopf and T. Bortfeld, The multiple-sets split feasibility problem and its applications for inverse problems, Inverse Problems, 2005, 21(6), 2071. doi: 10.1088/0266-5611/21/6/017
CrossRef Google Scholar
|
|
[8]
|
Y. Censor, A. Gibali and S. Reich, Algorithms for the split variational inequality problem, Numer. Algorithms, 2012, 59(2), 301-323. doi: 10.1007/s11075-011-9490-5
CrossRef Google Scholar
|
|
[9]
|
Y. Censor, A. Motova and A. Segal, Perturbed projections and subgradient projections for the multiple-sets split feasibility problem, J. Math. Anal. Appl., 2007, 327(2), 1244-1256. doi: 10.1016/j.jmaa.2006.05.010
CrossRef Google Scholar
|
|
[10]
|
Y. Censor and A. Segal, The split common fixed point problem for directed operators, J. Convex Anal., 2009, 16(2), 587-600.
Google Scholar
|
|
[11]
|
S. Y. Cho, Strong convergence analysis of a hybrid algorithm for nonlinear operators in a Banach space, J. Appl. Anal. Comput., 2018, 8(1), 19-31.
Google Scholar
|
|
[12]
|
S. Y. Cho, B. A. Bin Dehaish and X. Qin, Weak convergence of a splitting algorithm in Hilbert spaces, J. Appl. Anal. Comput., 2017, 7(2), 427-438.
Google Scholar
|
|
[13]
|
I. Cioranescu, Geometry of Banach Spaces, Duality Mappings and Nonlinear Problems, Springer Science & Business Media, 2012.
Google Scholar
|
|
[14]
|
P. L. Combettes and S. A. Hirstoaga, Equilibrium programming in Hilbert spaces, J. Nonlinear Convex Anal., 2005, 6(1), 117-136.
Google Scholar
|
|
[15]
|
V. H. Dang, New inertial algorithm for a class of equilibrium problems, Numer. Algorithms, 2019, 80(4), 1413-1436. doi: 10.1007/s11075-018-0532-0
CrossRef Google Scholar
|
|
[16]
|
L. Liu, A hybrid steepest descent method for solving split feasibility problems involving nonexpansive mappings, J. Nonlinear Convex Anal., 2019, 20(3), 471-488.
Google Scholar
|
|
[17]
|
L. Liu, X. Qin and R. P. Agarwal, Iterative methods for fixed points and zero points of nonlinear mappings with applications, Optimization, 2019, 1-21.
Google Scholar
|
|
[18]
|
Z. Ma, L. Wang and S. Chang, On the split feasibility problem and fixed point problem of quasi-$\phi$-nonexpansive mapping in Banach spaces, Numer. Algorithms, 2019, 80(4), 1203-1218. doi: 10.1007/s11075-018-0523-1
CrossRef Google Scholar
|
|
[19]
|
P. E. Maingé and A. Moudafi, Convergence of new inertial proximal methods for DC programming, SIAM J. Optim., 2008, 19(1), 397-413. doi: 10.1137/060655183
CrossRef Google Scholar
|
|
[20]
|
B. Martinet, Brève communication. Régularisation d'inéquations variationnelles par approximations successives, Revue française d'informatique et de recherche opérationnelle. Série rouge, 1970, 4(R3), 154-158.
Google Scholar
|
|
[21]
|
X. Qin and N. T. An, Smoothing algorithms for computing the projection onto a Minkowski sum of convex sets, Comput. Optim. Appl., 2019, 74(3), 821-850. doi: 10.1007/s10589-019-00124-7
CrossRef Google Scholar
|
|
[22]
|
X. Qin, A. Petrusel and J. C. Yao, CQ iterative algorithms for fixed points of nonexpansive mappings and split feasibility problems in Hilbert spaces, J. Nonlinear Convex Anal., 2018, 19(1), 157-165.
Google Scholar
|
|
[23]
|
X. Qin and J. C. Yao, A viscosity iterative method for a split feasibility problem, J. Nonlinear Convex Anal., 2019, 20(8), 1497-1506.
Google Scholar
|
|
[24]
|
Y. Shehu, O. Iyiola and E. Akaligwo, Modified inertial methods for finding common solutions to variational inequality problems, Fixed Point Theory, 2019, 20(2), 683-702. doi: 10.24193/fpt-ro.2019.2.45
CrossRef Google Scholar
|
|
[25]
|
Y. Shehu, F. Ogbuisi and O. Iyiola, Convergence analysis of an iterative algorithm for fixed point problems and split feasibility problems in certain Banach spaces, Optimization, 2016, 65(2), 299-323. doi: 10.1080/02331934.2015.1039533
CrossRef Google Scholar
|
|
[26]
|
W. Takahashi, Mann and Halpern iterations for the split common fixed point problem in Banach spaces, Linear Nonlinear Anal., 2017, 3, 1-18.
Google Scholar
|
|
[27]
|
W. Takahashi, Weak and strong convergence theorems for new demimetric mappings and the split common fixed point problem in Banach spaces, Numer. Funct. Anal. Optim., 2018, 39(10), 1011-1033. doi: 10.1080/01630563.2018.1466803
CrossRef Google Scholar
|
|
[28]
|
W. Takahashi and J. C. Yao, Strong convergence theorems by hybrid methods for the split common null point problem in Banach spaces, Fixed Point Theory Appl., 2015, 2015(1), 87. doi: 10.1186/s13663-015-0324-3
CrossRef Google Scholar
|
|
[29]
|
J. Tang, S. Chang, L. Wang and X. Wang, On the split common fixed point problem for strict pseudocontractive and asymptotically nonexpansive mappings in Banach spaces, J. Inequal. Appl., 2015, 2015(1), 305.
Google Scholar
|
|
[30]
|
H. Xu, Inequalities in Banach spaces with applications, Nonlinear Anal., 1991, 16(12), 1127-1138. doi: 10.1016/0362-546X(91)90200-K
CrossRef Google Scholar
|
|
[31]
|
X. Zhang, L. Wang, Z. Ma and L. Qin, The strong convergence theorems for split common fixed point problem of asymptotically nonexpansive mappings in Hilbert spaces, J. Inequal. Appl., 2015, 2015(1), 1.
Google Scholar
|




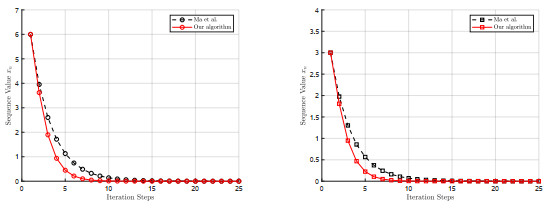

 DownLoad:
DownLoad: