BIFURCATION OF LIMIT CYCLES FROM A COMPOUND LOOP WITH FIVE SADDLES

Lijuan Sheng; Maoan Han

doi:10.11948/20190342

2019 Volume 9 Issue 6

Article Contents

Article navigation > Journal of Applied Analysis & Computation > 2019 > 9(6): 2482-2495

Next Article Previous Article

Lijuan Sheng, Maoan Han. BIFURCATION OF LIMIT CYCLES FROM A COMPOUND LOOP WITH FIVE SADDLES[J]. Journal of Applied Analysis & Computation, 2019, 9(6): 2482-2495. doi: 10.11948/20190342

Citation:

Lijuan Sheng, Maoan Han. BIFURCATION OF LIMIT CYCLES FROM A COMPOUND LOOP WITH FIVE SADDLES[J]. Journal of Applied Analysis & Computation, 2019, 9(6): 2482-2495. doi: 10.11948/20190342

BIFURCATION OF LIMIT CYCLES FROM A COMPOUND LOOP WITH FIVE SADDLES

Lijuan Sheng¹,
Maoan Han^2,1, ,

1.
Department of Mathematics, Shanghai Normal University, Shanghai 200234, China
2.
Department of Mathematics, Zhejiang Normal University Jinhua, Zhejiang 321004, China

Corresponding author: Email address:mahan@shnu.edu.cn(M. Han)
Fund Project: The authors were supported by National Natural Science Foundation of China (Nos. 11801372, 11931016, 11771296, 11971317 and 11871042)

Abstract

Abstract

We concern the number of limit cycles of a polynomial system with degree nine. We prove that under different conditions, the system can have 12 and 20 limit cycles bifurcating from a compound loop with five saddles. Our method relies upon the Melnikov function method and the method of stability-changing of a double homoclinic loop proposed by the authors[J. Yang, Y. Xiong and M. Han, Nonlinear Anal-Theor., 2014, 95, 756-773].
- Limit cycle /
- bifurcation /
- Melnikov function /
- homoclinic loop
MSC: 34C07

FullText(HTML)

References

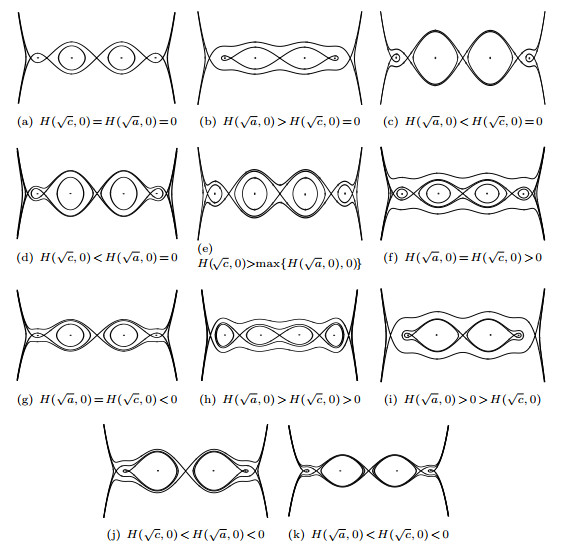
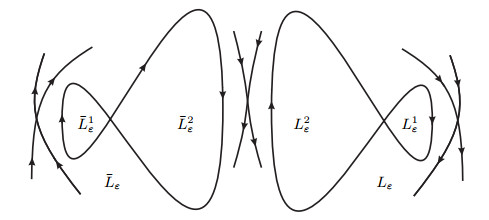
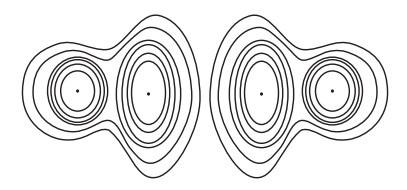
[1]	V. I. Arnold, Loss of stability of self-oscillations close to resonance and versal deformations of equivariant vector fields, Functional Analysis and Its Applications, 1977, 11, 85–92. doi: 10.1007/BF01081886 CrossRef Google Scholar
[2]	M. Caubergh, F. Dumortier and R. Roussarie, Alien limit cycles near a Hamiltonian 2-saddle cycle, C. R. Acad. Sci. Paris, Ser. I, 2005, 340(8), 587–592. doi: 10.1016/j.crma.2005.03.009 CrossRef Google Scholar
[3]	L. Chen and M. Wang, The relative position and the number of limit cycles of a quadratic differential system, Acta Math. Sinica, 1979, 22(6), 751–758. Google Scholar
[4]	B. Coll, F. Dumortier and R. Prohens, Alien limit cycles in Lienard equations, Journal of Differential Equations, 2013, 254(3), 1582–1600. Google Scholar
[5]	F. Dumortier and R. Roussarie, Abelian integrals and limit cycles, Journal of Differential Equations, 2006, 227(1), 116–165. Google Scholar
[6]	M. Han, Cyclicity of planar homoclinic loops and quadratic integrable systems, Science in China Series A: Mathematics, 1997, 40(12), 1247–1258. doi: 10.1007/BF02876370 CrossRef Google Scholar
[7]	M. Han, Bifurcation Theory of Limit Cycles, Science Press, Beijing, 2013. Google Scholar
[8]	M. Han, S. Hu and X. Liu, On the stability of double homoclinic and heteroclinic cycles, Nonlinear Analysis: Theory, Methods & Applications, 2003, 53(5), 701–713. Google Scholar
[9]	M. Han and J. Li, Lower bounds for the Hilbert number of polynomial systems, Journal of Differential Equations, 2012, 252(4), 3278–3304. Google Scholar
[10]	M. Han, J. Yang, A. Tarta and G. Yang, Limit cycles near homoclinic and heteroclinic loops, J. Dynam. Differential Equations, 2008, 20, 923–944. doi: 10.1007/s10884-008-9108-3 CrossRef Google Scholar
[11]	M. Han and Y. Ye, On the coefficients appearing in the expansion of Melnikov function in homoclinic bifurcations, Ann. Differ. Equ., 1998, 14(2), 156–162. Google Scholar
[12]	M. Han and Z. Zhang, Cyclicity 1 and 2 conditions for a 2-polycycle of integrable systems on the plane, Journal of Differential Equations, 1999, 155(2), 245–261. Google Scholar
[13]	M. Han and H. Zhu, The loop quantities and bifurcations of homoclinic loops, Journal of Differential Equations, 2007, 234(2), 339–359. Google Scholar
[14]	J. Huang, H. Liang and J. Llibre, Non-existence and uniqueness of limit cycles for planar polynomial differential systems with homogeneous nonlinearities, Journal of Differential Equations, 2018, 265(9), 3888–3913. doi: 10.1016/j.jde.2018.05.019 CrossRef Google Scholar
[15]	C. Li, C. Liu and J. Yang, A cubic system with thirteen limit cycles, Journal of Differential Equations, 2009, 246(9), 3609–3619. doi: 10.1016/j.jde.2009.01.038 CrossRef Google Scholar
[16]	J. Li, Hilbert's 16th problem and bifurcations of planar vector fields, Int. J. Bifurcat. Chaos, 2003, 13(1), 47–106. doi: 10.1142/S0218127403006352 CrossRef Google Scholar
[17]	J. Li and Y. Liu, New results on the study of z_q-equivariant planar polynomial vector fields, Qualitative Theory of Dynamical Systems, 2010, 9(1–2), 167–219. doi: 10.1007/s12346-010-0024-7 CrossRef Google Scholar
[18]	J. Llibre, R. Ramirez, V. Ramirez and N. Sadovskaia, The 16th Hilbert problem restricted to circular algebraic limit cycles, Journal of Differential Equations, 2016, 260(7), 5726–5760. doi: 10.1016/j.jde.2015.12.019 CrossRef Google Scholar
[19]	R. Roussarie, On the number of limit cycles which appear by perturbation of separatrix loop of planar vector fields, Bol. Soc. Brasil. Mat., 1986, 17(2), 67–101. doi: 10.1007/BF02584827 CrossRef Google Scholar
[20]	L. Sheng, M. Han and Y. Tian, On the number of limit cycles bifurcating from a compound polycycle, Int. J. Bifurcat. Chaos, Accepted. Google Scholar
[21]	S. Shi, A concrete example of the existence of four limit cycles for plane quadratic systems, Sci. Sinica, 1980, 23(2), 153–158. Google Scholar
[22]	X. Sun and L. Zhao, Perturbations of a class of hyper-elliptic Hamiltonian systems of degree seven with nilpotent singular points, Applied Mathematics and Computation, 2016, 289, 194–203. doi: 10.1016/j.amc.2016.04.018 CrossRef Google Scholar
[23]	Y. Tian and M. Han, Hopf and homoclinic bifurcations for near-Hamiltonian systems, Journal of Differential Equations, 2017, 262(4), 3214–3234. doi: 10.1016/j.jde.2016.11.026 CrossRef Google Scholar
[24]	J. Yang, Y. Xiong and M. Han, Limit cycle bifurcations near a 2-polycycle or double 2-polycycle of planar systems, Nonlinear Analysis-Theory Methods & Applications, 2014, 95, 756–773. Google Scholar
[25]	J. Yang, P. Yu and M. Han, Limit cycle bifurcations near a double homoclinic loop with a nilpotent saddle of order m, Journal of Differential Equations, 2019, 266(1), 455–492. doi: 10.1016/j.jde.2018.07.042 CrossRef Google Scholar
[26]	X. Zhang, The 16th Hilbert problem on algebraic limit cycles, Journal of Differential Equations, 2011, 251(7), 1778–1789. doi: 10.1016/j.jde.2011.06.008 CrossRef Google Scholar