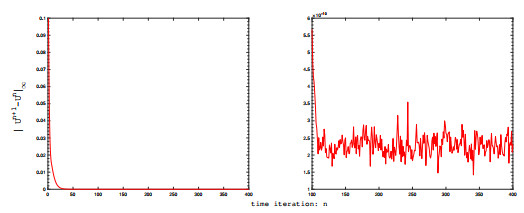
|
[1]
|
N. E. Alaa and M. Pierre, Convergence to equilibrium for discretized gradient-like systems with analytic features, IMA J. Numer. Anal., 2013, 33(4), 1291-1321. doi: 10.1093/imanum/drs042
CrossRef Google Scholar
|
|
[2]
|
S. Allen and J. Cahn, A microscopic theory for antiphase boundary motion and its application to antiphase domain coarsing, Acta. Metall., 1979, 27, 1084-1095. doi: 10.1016/0001-6160(79)90196-2
CrossRef Google Scholar
|
|
[3]
|
P. F. Antonietti, B. Merlet, M. Pierre and M. Verani, Convergence to equilibrium for a second-order time semi-discretization of the Cahn-Hilliard equation, AIMS Mathematics, 2016, 1(3), 178-194. doi: 10.3934/Math.2016.3.178
CrossRef Google Scholar
|
|
[4]
|
S. Badia, F. Guillén-González and J. V. Gutiérrez-Santacreu, Finite element approximation of nematic liquid crystal flows using a saddle-point structure, J. Comput. Physics, 2011, 230, 1686-1706. doi: 10.1016/j.jcp.2010.11.033
CrossRef Google Scholar
|
|
[5]
|
A. Bouchriti, M. Pierre and N. E. Alaa, Gradient stability of high-order BDF methods and some applications, J. Difference Equ. Appl., 2020, 0(0), 1-30.
Google Scholar
|
|
[6]
|
J. W. Cahn and J. E. Hilliard, Free energy of a nonuniform system. Ⅰ. Interfacial free energy, J. Chem. Phys., 1958, 28, 258-267. doi: 10.1063/1.1744102
CrossRef Google Scholar
|
|
[7]
|
Q. Cheng, J. Shen and X. Yang, Highly efficient and accurate numerical schemes for the epitaxial thin film growth models by using the SAV approach, J. Sci. Comput., 2019, 78(3), 1467-1487. doi: 10.1007/s10915-018-0832-5
CrossRef Google Scholar
|
|
[8]
|
K. R. Elder, M. Katakowski, M. Haataja and M. Grant, Modeling elasticity in crystal growth, Phys. Rev. Lett., 2002, 88, 245701. doi: 10.1103/PhysRevLett.88.245701
CrossRef Google Scholar
|
|
[9]
|
A. Ern and J. L. Guermond, Theory and practice of finite elements, 159 of Applied Mathematical Sciences, Springer-Verlag, New York, 2004.
Google Scholar
|
|
[10]
|
S. Gatti, M. Grasselli, A. Miranville and V. Pata, A construction of a robust family of exponential attractors, Proc. Amer. Math. Soc., 2006, 134(1), 117-127.
Google Scholar
|
|
[11]
|
D. Gilbarg and N. S. Trudinger, Elliptic partial differential equations of second order, Classics in Mathematics, Springer-Verlag, Berlin, 2001.
Google Scholar
|
|
[12]
|
H. Gomez and T. J. R. Hughes, Provably unconditionally stable, second order time-accurate, mixed variational methods for phase-field models, J. Comput. Phys., 2011, 230(13), 5310-5327. doi: 10.1016/j.jcp.2011.03.033
CrossRef Google Scholar
|
|
[13]
|
O. Goubet, Remarks on some dissipative sine-Gordon equations, Complex Var. Elliptic Equ., 2019, 0(0), 1-7.
Google Scholar
|
|
[14]
|
M. Grasselli and M. Pierre, Energy stable and convergent finite element schemes for the modified phase field crystal equation, ESAIM Math. Model. Numer. Anal., 2016, 50(5), 1523-1560. doi: 10.1051/m2an/2015092
CrossRef Google Scholar
|
|
[15]
|
F. Guillén-González and G. Tierra, On linear schemes for a Cahn-Hilliard diffuse interface model, J. Comput. Physics, 2013, 234, 140-171. doi: 10.1016/j.jcp.2012.09.020
CrossRef Google Scholar
|
|
[16]
|
A. Haraux, Systèmes dynamiques dissipatifs et applications, 17 of Recherches en Mathématiques Appliquées, Masson, Paris, 1991.
Google Scholar
|
|
[17]
|
A. Haraux and M. A. Jendoubi, The convergence problem for dissipative autonomous systems, SpringerBriefs in Mathematics, Springer, Cham; BCAM Basque Center for Applied Mathematics, Bilbao, 2015.
Google Scholar
|
|
[18]
|
C. Jiang, W. Cai and Y. Wang, A linearly implicit and local energy-preserving scheme for the sine-Gordon equation based on the invariant energy quadratization approach, J. Sci. Comput., 2019, 80(3), 1629-1655. doi: 10.1007/s10915-019-01001-5
CrossRef Google Scholar
|
|
[19]
|
M. D. Johnson, C. Orme, A. W. Hunt et al., Stable and unstable growth in molecular beam epitaxy, Phys. Rev. Lett., 1994, 72, 116-119. doi: 10.1103/PhysRevLett.72.116
CrossRef Google Scholar
|
|
[20]
|
O. Kavian, Introduction à la théorie des points critiques et applications aux problèmes elliptiques, 13 of Mathématiques & Applications (Berlin), Springer-Verlag, Paris, 1993.
Google Scholar
|
|
[21]
|
H. Khalfi, M. Pierre, N. E. Alaa and M. Guedda, Convergence to equilibrium of a DC algorithm for an epitaxial growth model, Int. J. Numer. Anal. Model., 2019, 16(3), 398-411.
Google Scholar
|
|
[22]
|
H. Kielhöfer, Bifurcation theory, 156 of Applied Mathematical Sciences, 2nd Edn, Springer, New York, 2012.
Google Scholar
|
|
[23]
|
Q. Li, L. Mei, X. Yang and Y. Li, Efficient numerical schemes with unconditional energy stabilities for the modified phase field crystal equation, Adv. Comput. Math., 2019, 45(3), 1551-1580. doi: 10.1007/s10444-019-09678-w
CrossRef Google Scholar
|
|
[24]
|
X. Li, J. Shen and H. Rui, Energy stability and convergence of SAV block-centered finite difference method for gradient flows, Math. Comp., 2019, 88(319), 2047-2068. doi: 10.1090/mcom/3428
CrossRef Google Scholar
|
|
[25]
|
Z. Liu and X. Li, Efficient modified techniques of invariant energy quadratization approach for gradient flows, Appl. Math. Lett., 2019, 98, 206-214. doi: 10.1016/j.aml.2019.06.006
CrossRef Google Scholar
|
|
[26]
|
M. Pierre and P. Rogeon, Convergence to equilibrium for a time semi-discrete damped wave equation, J. Appl. Anal. Comput., 2016, 6(4), 1041-1048.
Google Scholar
|
|
[27]
|
J. Shen and J. Xu, Convergence and error analysis for the scalar auxiliary variable (SAV) schemes to gradient flows, SIAM J. Numer. Anal., 2018, 56(5), 2895-2912. doi: 10.1137/17M1159968
CrossRef Google Scholar
|
|
[28]
|
J. Shen, J. Xu and J. Yang, The scalar auxiliary variable (SAV) approach for gradient flows, J. Comput. Phys., 2018, 353, 407-416. doi: 10.1016/j.jcp.2017.10.021
CrossRef Google Scholar
|
|
[29]
|
J. Shen and X. Yang, Numerical approximations of Allen-Cahn and Cahn-Hilliard equations, Discrete Contin. Dyn. Syst., 2010, 28(4), 1669-1691. doi: 10.3934/dcds.2010.28.1669
CrossRef Google Scholar
|
|
[30]
|
S. Sun, X. Jing and Q. Wang, Error estimates of energy stable numerical schemes for Allen-Cahn equations with nonlocal constraints, J. Sci. Comput., 2018, 79, 593-623.
Google Scholar
|
|
[31]
|
R. Temam, Infinite-dimensional dynamical systems in mechanics and physics, 68 of Applied Mathematical Sciences, 2nd Edn, Springer-Verlag, New York, 1997.
Google Scholar
|
|
[32]
|
G. Tierra and F. Guillén-González, Numerical methods for solving the Cahn-Hilliard equation and its applicability to related energy-based models, Arch. Comput. Methods Eng., 2015, 22(2), 269-289. doi: 10.1007/s11831-014-9112-1
CrossRef Google Scholar
|
|
[33]
|
X. Yang, Linear, first and second-order, unconditionally energy stable numerical schemes for the phase field model of homopolymer blends, J. Comput. Physics, 2016, 327, 294-316. doi: 10.1016/j.jcp.2016.09.029
CrossRef Google Scholar
|
|
[34]
|
S. Zelik, Asymptotic regularity of solutions of a nonautonomous damped wave equation with a critical growth exponent, Commun. Pure Appl. Anal., 2004, 3(4), 921-934. doi: 10.3934/cpaa.2004.3.921
CrossRef Google Scholar
|




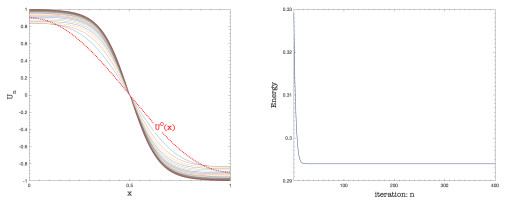

 DownLoad:
DownLoad: