| Citation: |
Lihong Huang, Huili Ma, Jiafu Wang, Chuangxia Huang. GLOBAL DYNAMICS OF A FILIPPOV PLANT DISEASE MODEL WITH AN ECONOMIC THRESHOLD OF INFECTED-SUSCEPTIBLE RATIO[J]. Journal of Applied Analysis & Computation, 2020, 10(5): 2263-2277. doi: 10.11948/20190409
|
GLOBAL DYNAMICS OF A FILIPPOV PLANT DISEASE MODEL WITH AN ECONOMIC THRESHOLD OF INFECTED-SUSCEPTIBLE RATIO
-
Abstract
This paper presents a Filippov plant disease model incorporating an economic threshold of infected-susceptible ratio, above which control strategies of replanting or removing are needed to be carried out. Based on the Filippov approach, we study the sliding mode dynamics and further the global dynamics. It is shown that there is a unique equilibrium, which is a disease-free equilibrium, an endemic equilibrium or a pseudo-equilibrium. Moreover, the equilibrium is proved to be globally asymptotically stable. Our results indicate that the control goal can be achieved by taking appropriate replanting and removing rate.-
Keywords:
- Filippov systems /
- plant disease model /
- economic threshold /
- stability
-
-
References
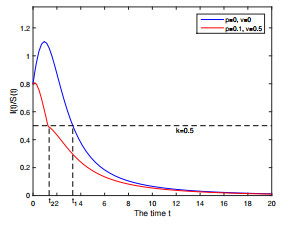
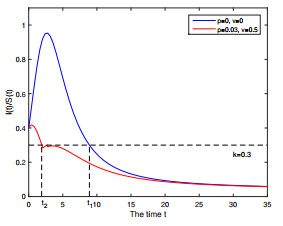
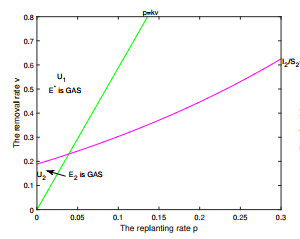
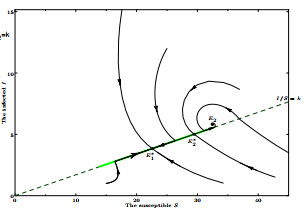
[1] C. Chen and Y. Kang, Sliding motion and global dynamics of a Filippov fire-blight model with economic thresholds, Nonlinear Anal.-Real World Appl., 2018, 39, 492-519. doi: 10.1016/j.nonrwa.2017.08.002 [2] T. Chen, L. Huang, P. Yu and W Huang, Bifurcation of limit cycles at infinity in piecewise polynomial systems, Nonlinear Anal.-Real World Appl., 2018, 41, 82-106. doi: 10.1016/j.nonrwa.2017.10.003 [3] Z. Cai, J. Huang and L. Huang, Periodic orbit analysis for the delayed Filippov system, Proc. Amer. Math. Soc., 2018, 146 (11), 4667-4682. doi: 10.1090/proc/13883 [4] Z. Cai, J. Huang and L. Huang, Generalized Lyapunov-Razumikhin method for retarded differential inclusions: Applications to discontinuous neural networks, Discrete Contin. Dyn. Syst.-Ser. B, 2017, 22(9), 3591-3614. doi: 10.3934/dcdsb.2017181 [5] N. Chong, Modeling avian influenza using Filippov systems to determine culling of infected birds and quarantine, Nonlinear Anal.-Real World Appl., 2015, 24, 196-218. doi: 10.1016/j.nonrwa.2015.02.007 [6] M. Chan and M. J. Jeger, An analytical model of plant virus disease dynamics with roguing and replanting, J. Appl. Ecol., 1994, 31(3), 413-427. doi: 10.2307/2404439 [7] X. Chen and L. Huang, A Filippov system describing the effect of prey refuge use on a ratio-dependent predator-prey model, J. Math. Anal. Appl., 2015, 428(2), 817-837. doi: 10.1016/j.jmaa.2015.03.045 [8] A. F. Filippov, Differential Equations with Discontinuous Right-Hand Sides, Kluwer Academic Publishers, Dordrecht, The Netherlands, 1988. [9] S. Fishman, R. Marcus, H. Talpaz, et al, Epidemiological and economic models for spread and control of citrus tristeza virus disease, Phytoparasitica, 1983, 11(1), 39-49. doi: 10.1007/BF02980710 [10] S. Fishman and R. Marcus, A model for spread of plant disease with periodic removals, J. Math. Biol., 1984, 21(2), 149-158. doi: 10.1007/BF00277667 [11] R. W. Gibson and V. Aritua, The perspective of sweetpotato chlorotic stunt virus in sweetpotato production in Africa: a review, 2002. [12] Z. Guo, L. Huang and X. Zou, Impact of discontinuous treatments on disease dynamics in an SIR epidemic model, Math. Biosci. Eng., 2013, 9(1), 97-110. [13] C. Huang, Z. Yang, T. Yi and X. Zou, On the basins of attraction for a class of delay differential equations with non-monotone bistable nonlinearities, J. Differ. Equ., 2014, 256(7), 2101-2114. doi: 10.1016/j.jde.2013.12.015 [14] H. Hu, X. Yuan, L. Huang and C. Huang, Global dynamics of an SIRS model with demographics and transfer from infectious to susceptible on heterogeneous networks, Math. Biosci. Eng, 2019, 16(5), 5729-5749. doi: 10.3934/mbe.2019286 [15] C. Huang, Y. Qiao, L. Huang and R. P. Agarwal, Dynamical behaviors of a food-chain model with stage structure and time delays, Adv. Differ. Equ., 2018, 2018(1), 186. doi: 10.1186/s13662-018-1589-8 [16] M. Han, H. Sun and Z. Balanov, Upper estimates for the number of periodic solutions to multi-dimensional systems, J. Differ. Equ., 2019, 266(12), 8281-8293. doi: 10.1016/j.jde.2018.12.034 [17] M. Han, On the maximum number of periodic solutions of piecewise smooth periodic equations by average method, J. Appl. Anal. Comput., 2017, 7(2), 788-794. [18] H. Hu and X. Zou, Existence of an extinction wave in the fisher equation with a shifting habitat, Proc. Amer. Math. Soc., 2017, 145(11), 4763-4771. doi: 10.1090/proc/13687 [19] F. Jiang and M. Han, Qualitative analysis of crossing limit cycles in discontinuous Liénard-type differential systems, Journal of Nonlinear Modeling and Analysis, 2019, 1(4), 527-543. [20] L. Sheng and M. Han, Bifurcation of limit cycles from a compound loop with five saddles, J. Appl. Anal. Comput., 2019, 9(6), 2482-2495. [21] R. N. Strange and P. R. Scott, Plant Disease: A threat to global food security, Annu. Rev. Phytopathol., 2005, 43(1), 83-116. doi: 10.1146/annurev.phyto.43.113004.133839 [22] Y. Tian, M. Han and F. Xu, Bifurcations of small limit cycles in Liénard systems with cubic restoring terms, J. Differ. Equ., 2019, 267(3), 1561-1580. doi: 10.1016/j.jde.2019.02.018 [23] H. R. Thieme and J. A. P. Heesterbeek, How to estimate the efficacy of periodic control of an infectious plant disease, Math. Biosci., 1989, 93(1), 0-29. [24] S. Tang, Y. Xiao and R. A. Cheke, Dynamical analysis of plant disease models with cultural control strategies and economic thresholds, Math. Comput. Simul., 2010, 80(5), 894-921. doi: 10.1016/j.matcom.2009.10.004 [25] M. Vurro, B. Bonciani and G. Vannacci, Emerging infectious diseases of crop plants in developing countries: impact on agriculture and socio-economic consequences, Food Security, 2010, 2(2), 113-132. doi: 10.1007/s12571-010-0062-7 [26] J. Wang, F. Zhang and L. Wang, Equilibrium, pseudoequilibrium and sliding-mode heteroclinic orbit in a Filippov-type plant disease model, Nonlinear Anal.-Real World Appl., 2016, 31, 308-324. doi: 10.1016/j.nonrwa.2016.01.017 [27] A. Wang and Y. Xiao, A Filippov system describing media effects on the spread of infectious diseases, Nonlinear Anal.-Hybrid Syst., 2014, 11(1), 84-97. [28] A. Wang and Y. Xiao, Sliding bifurcation and global dynamics of a Filippov epidemic model with vaccination, Int. J. Bifurcation Chaos, 2013, 23(8), 1350144. doi: 10.1142/S0218127413501447 [29] J. Wang, X. Chen and L. Huang, The number and stability of limit cycles for planar piecewise linear systems of node-saddle type, J. Math. Anal. Appl., 2019, 469(1), 405-427. doi: 10.1016/j.jmaa.2018.09.024 [30] J. Wang, C. Huang and L. Huang, Discontinuity-induced limit cycles in a general planar piecewise linear system of saddle-focus type, Nonlinear Anal.-Hybrid Syst., 2019, 33, 162-178. doi: 10.1016/j.nahs.2019.03.004 [31] J. Wang, S. He and L. Huang, Limit cycles induced by threshold nonlinearity in planar piecewise linear systems of node-focus or node-center type, Int. J. Bifur. Chaos, 2020, DOI:10.1142/S0218127420501606. [32] R. Yuan and Z. Wang, A HIV infection model with periodic multidrug therapy, Journal of Nonlinear Modeling and Analysis, 2019, 1(4), 573-593. [33] C. Yang, L. Huang and F. Li, Exponential synchronization control of discontinuous nonautonomous networks and autonomous coupled networks, Complexity, 2028, 2018, 1-10. [34] T. Zhao and Y. Xiao, Non-smooth plant disease models with economic thresholds, Math. Biosci., 2013, 241(1), 34-48. -
-
Figures(10)
Export File
Citation
Lihong Huang, Huili Ma, Jiafu Wang, Chuangxia Huang. GLOBAL DYNAMICS OF A FILIPPOV PLANT DISEASE MODEL WITH AN ECONOMIC THRESHOLD OF INFECTED-SUSCEPTIBLE RATIO[J]. Journal of Applied Analysis & Computation, 2020, 10(5): 2263-2277. doi: 10.11948/20190409
Format
Content
DownLoad:
-
Figure 1. The possible closed orbit containing a part of
$ \overline{\Sigma}_s $ if$ E_1 $ is real and$ E_2 $ is virtual. -
Figure 2. The possible closed orbit surrounding
$ \overline{\Sigma}_s $ . -
Figure 3. Global asymptotic stability of the disease-free equilibrium
$ E^1_0 $ , where the parameter are chosen as follows:$ \eta_1 = 0.6, \eta_2 = 0.9, A = 5, \beta = 0.1, p = 0.1, v = 0.6, k = 0.5 $ . -
Figure 4. Global asymptotic stability of the endemic equilibrium
$ E_1 $ , where the parameters are chosen as follows:$ \eta_1=0.3,\eta_2=0.8,A=8,\beta=0.04,p=0.1,v=0.5,k=0.5 $ . -
Figure 5. Global asymptotic stability of the endemic equilibrium
$ E_2 $ , where the parameters are fixed as follows:$ \eta_1=0.2, \eta_2=0.5, A=20, \beta=0.1, p=0.1, v=0.8, k=0.4 $ . -
Figure 6. Global asymptotic stability of the pseudo-equilibrium
$ E^* $ , where the parameters are fixed as follows:$ \eta_1 = 0.55, \eta_2 = 0.8, A = 15, \beta = 0.1, p = 0.5, v = 0.9, k = 0.6 $ . -
Figure 7. The graph of the infected-susceptible plants ratio
$ \frac{I(t)}{S(t)} $ with different control parameters$ p $ and$ v $ when$ R_1<1 $ , where the parameters are chosen as follows:$ \eta_1=0.6, \eta_2=0.8, A=10, \beta=0.04, k=0.5 $ and the initial value$ (S(0),I(0))=(20,16) $ . -
Figure 8. The graph of the infected-susceptible plants ratio
$ \frac{I(t)}{S(t)} $ with different control parameters$ p $ and$ v $ when$ R_1>1 $ and$ A\beta-k\eta_2^2-\eta_1\eta_2<0 $ . The parameters are chosen as follows:$ \eta_1=0.3, \eta_2=0.5, A=4, \beta=0.04, k=0.3 $ and the initial value$ (S(0),I(0))=(20,8) $ . -
Figure 9. The parameter region in the
$ p-v $ plane, where the equilibrium$ E^* $ or$ E_2 $ is globally asymptotically stable (GAS) if$ R_1>1 $ and$ A\beta-k\eta_2^2-\eta_1\eta_2>0 $ . The other parameters are picked up as follows:$ \eta_1=0.5, \eta_2=0.6, A=10, \beta=0.05, k=0.17 $ . Besides,${U_1} \cup {U_2} = \left\{ {(p,v)|p < \min \left\{ {kv,{\eta _1}} \right\},p \ge 0,v \ge 0} \right\}.$ . -
Figure 10. he system (2.1) is bistable if the parameters are chosen as:
$ \eta_1=0.5, \eta_2=0.6, A=10, \beta=0.05, k=0.17, p=0.4821,v=1.0241 $ .