| Citation: |
Xun Cao, Weihua Jiang. INTERACTIONS OF TURING AND HOPF BIFURCATIONS IN AN ADDITIONAL FOOD PROVIDED DIFFUSIVE PREDATOR-PREY MODEL[J]. Journal of Applied Analysis & Computation, 2019, 9(4): 1277-1304. doi: 10.11948/2156-907X.20180224
|
INTERACTIONS OF TURING AND HOPF BIFURCATIONS IN AN ADDITIONAL FOOD PROVIDED DIFFUSIVE PREDATOR-PREY MODEL
-
Abstract
Complex spatiotemporal dynamics of a diffusive predator-prey system involving additional food supply to predator and intra-specific competition among predator, are investigated. We establish critical conditions of the occurrence of Turing instability, which are necessary and sufficient. Furthermore, we also establish conditions of the occurrence of codimension-2 Turing-Hopf bifurcation and Turing-Turing bifurcation, by exploring interactions of Turing bifurcations and Hopf bifurcation. For Turing-Hopf bifurcation, by analyzing normal form truncated to order 3 which are derived by applying normal form method, it is shown that under proper conditions, diffusive predator-prey system generates interesting spatial, temporal and spatiotemporal patterns, including a pair of spatially inhomogeneous steady states, a spatially homogeneous periodic solution and a pair of spatially inhomogeneous periodic solutions. And numerical simulations are also shown to support theory analysis. Moreover, it is found that proper intra-specific competition among predator helps generate complex spatiotemporal dynamics. And, proper additional food supply to predator helps control the population fluctuations of predator and prey, while large quantity and high quality of additional food supply will lead to the extinction of prey and make predator change the source of food, which finally destroy the ecosystem. These investigations might help understand complex spatiotemporal dynamics of predator-prey system involving additional food supply to predator and intra-specific competition among predator, and help conserve species in an ecosystem via supplying suitable additional food. -
-
References
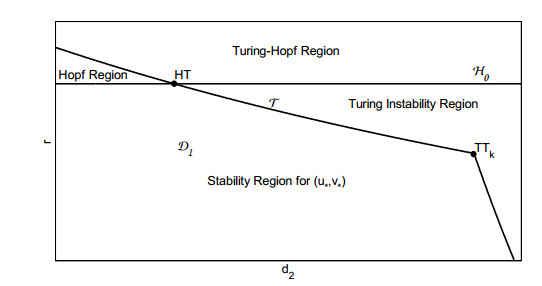
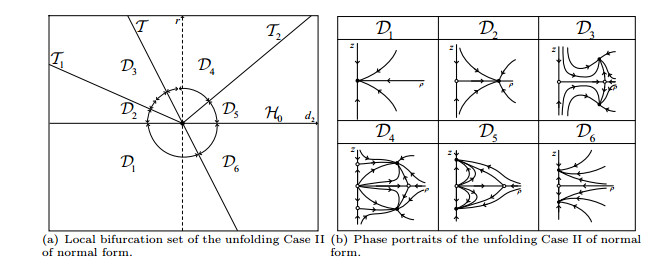
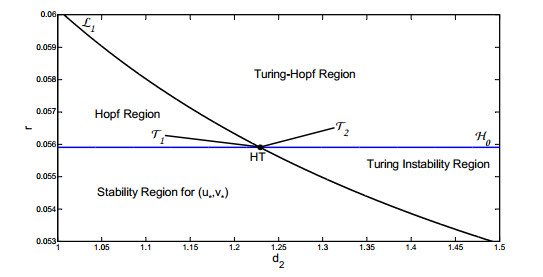
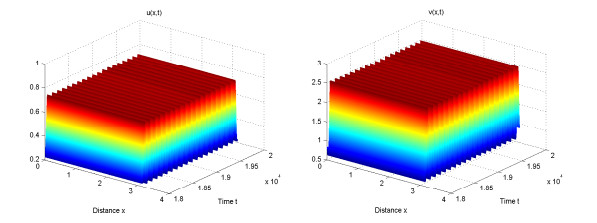
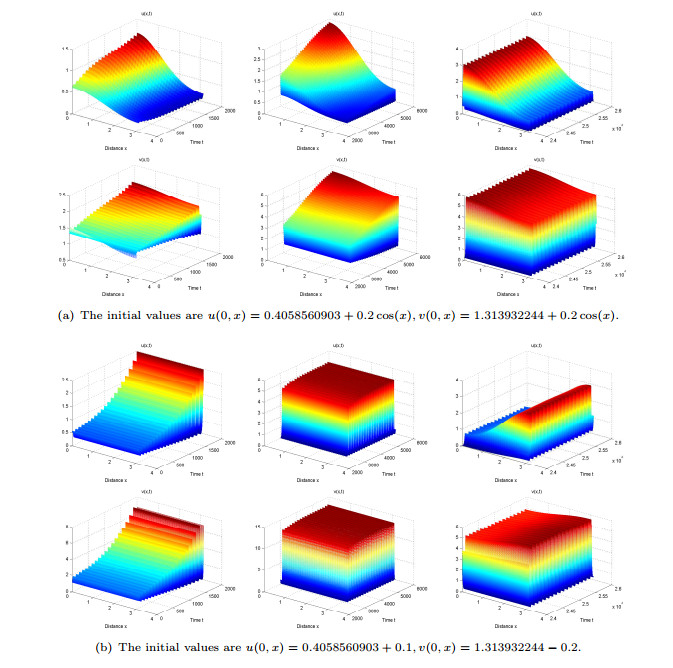
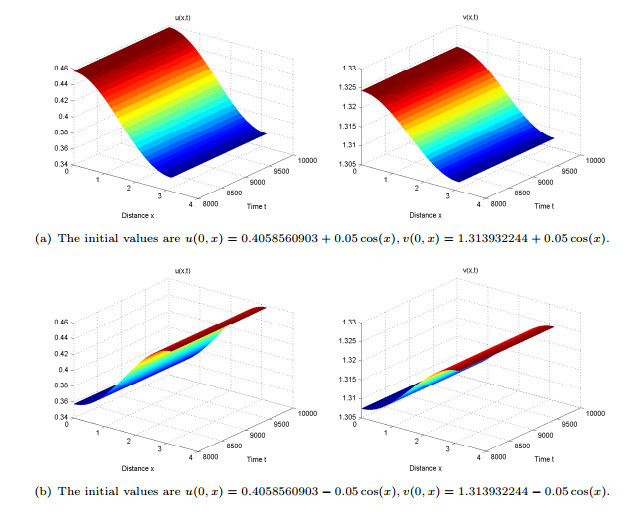
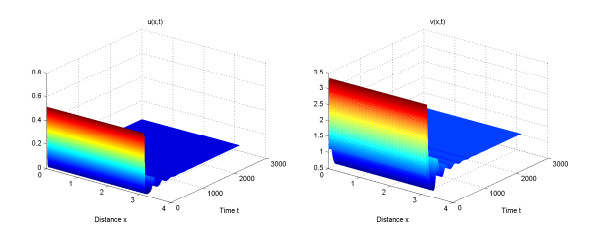
[1] M. Baurmann, T. Gross and U. Feudel, Instabilities in spatially extended predator-prey systems: Spatio-temporal patterns in the neighborhood of Turing-Hopf bifurcations, J. Theoret. Biol., 2007, 245(2), 220-229. doi: 10.1016/j.jtbi.2006.09.036 [2] A. D. Bazykin, A. I. Khibnik and B. Krauskopf, Nonlinear Dynamics of Interacting Populations, World Scientific, 1998. [3] B. I. Camara, M. Hague and H. Mokrani, Patterns formations in a diffusive ratio-dependent predator-prey model of interacting populations, Phys. A, 2016, 461, 374-383. doi: 10.1016/j.physa.2016.05.054 [4] S. Chakraborty, P. K. Tiwari, S. K. Sasmal et al., Interactive effects of prey refuge and additional food for predator in a diffusive predator-prey system, Appl. Math. Model., 2017, 47, 128-140. doi: 10.1016/j.apm.2017.03.028 [5] S. Chen, J. Shi and J. Wei, Bifurcation analysis of the Gierer-Meinhardt system with a saturation in the activator production, Appl. Anal., 2013, 93(6), 1115-1134. [6] H. Ddumba, J. Y. T. Mugisha, J. W. Gonsalves and G. I. H. Kerley, Periodicity and limit cycle perturbation analysis of a predator-prey model with interspecific species' interference, predator additional food and dispersal, Appl. Math. Comput., 2013, 219(15), 8338-8357. [7] A. Diouf, H. Mokrani, D. Ngom et al., Detection and computation of high codimension bifurcations in diffuse predator-prey systems, Phys. A, 2019, 516, 402-411. doi: 10.1016/j.physa.2018.10.027 [8] T. Faria, Normal forms and hopf bifurcation for partial differential equations with delays, Trans. Amer. Math. Soc., 2000, 352(5), 2217-2238. doi: 10.1090/S0002-9947-00-02280-7 [9] T. Faria, W. Huang and J. Wu, Smoothness of center manifolds for maps and formal adjoints for semilinear fdes in general Banach spaces, SIAM J. Appl. Math., 2002, 34(1), 173-203. doi: 10.1137/S0036141001384971 [10] S. Gakkhar and A. Singh, Control of chaos due to additional predator in the hastings-powell food chain model, J. Math. Anal. Appl., 2012, 385(1), 423-438. doi: 10.1016/j.jmaa.2011.06.047 [11] S. Ghorai and S. Poria, Pattern formation and control of spatiotemporal chaos in a reaction diffusion prey-predator system supplying additional food, Chaos Solitons Fractals, 2016, 85, 57-67. doi: 10.1016/j.chaos.2016.01.013 [12] J. Guckenheimer and P. Holmes, Nonlinear Oscillations, Dynamical Systems, and Bifurcations of Vector Fields, Springer, New York, 1983. [13] L. N. Guin and P. K. Mandal, Spatiotemporal dynamics of reaction-diffusion models of interacting populations, Appl. Math. Model., 2014, 38(17-18), 4417-4427. doi: 10.1016/j.apm.2014.02.022 [14] S. Guo and J. Man, Center manifolds theorem for parameterized delay differential equations with applications to zero singularities, Nonlinear Anal., 2011, 74(13), 4418-4432. doi: 10.1016/j.na.2011.04.003 [15] J. K. Hale, Theory of Functional Differential Equations, Springer-Verlag, 1977. [16] M. Haque, A detailed study of the Beddington-Deangelis predator-prey model, Math. Biosci., 2011, 234(1), 1-16. [17] C. S. Holling, The functional response of predators to prey density and its role in mimicry and population regulation, Memoirs of the Entomological Society of Canada, 1965, 97(45), 1-60. [18] W. Jiang, Q. An and J. Shi, Formulation of the normal forms of Turing-Hopf bifurcation in reaction-diffusion systems with time delay, submitted, 2018, arXiv: 1802.10286. [19] W. Jiang and Y. Yuan, Bogdanov-Takens singularity in van der pol's oscillator with delayed feedback, Phys. D, 2007, 227(2), 149-161. doi: 10.1016/j.physd.2007.01.003 [20] D. Kumar and S. P. Chakrabarty, A comparative study of bioeconomic ratio-dependent predator-prey model with and without additional food to predators, Nonlinear Dynam., 2015, 80(1-2), 23-38. doi: 10.1007/s11071-014-1848-5 [21] X. Li, W. Jiang and J. Shi, Hopf bifurcation and turing instability in the reaction-diffusion Holling-Tanner predator-prey model, IMA J. Appl. Math., 2011, 78(2), 287-306. [22] S. Ma and Z. Feng, Fold-hopf bifurcations of the Rose-Hindmarsh model with time delay, Int. J. Bifurc. Chaos, 2011, 21(2), 437-452. doi: 10.1142/S0218127411028490 [23] P. J. Pal, P. K. Mandal and K. K. Lahiri, A delayed ratio-dependent predator-prey model of interacting populations with Holling type iii functional response, Nonlinear Dynam., 2014, 76(1), 201-220. doi: 10.1007/s11071-013-1121-3 [24] B. Sahoo and S. Poria, Effects of additional food on an ecoepidemic model with time delay on infection, Appl. Math. Comput., 2014, 245, 17-35. [25] B. Sahoo and S. Poria, Effects of additional food in a delayed predator-prey model, Math. Biosci., 2015, 261, 62-73. doi: 10.1016/j.mbs.2014.12.002 [26] S. Samanta, R. Dhar, I. M. Elmojtaba and J. Chattopadhyay, The role of additional food in a predator-prey model with a prey refuge, J. Biol. Systems, 2016, 24(2-3), 345-365. [27] S. Sarwardi, M. Haque and P. K. Mandal, Persistence and global stability of bazykin predator-prey model with Beddington-Deangelis response function, Commun. Nonlinear Sci. Numer. Simul., 2014, 19(1), 189-209. doi: 10.1016/j.cnsns.2013.05.029 [28] S. K. Sasmal, Y. Kang and J. Chattopadhyay, Intra-specific competition in predator can promote the coexistence of an eco-epidemiological model with strong allee effects in prey, Biosystems, 2015, 137, 34-44. doi: 10.1016/j.biosystems.2015.09.003 [29] M. Sen, P. D. N. Srinivasu and M. Banerjee, Global dynamics of an additional food provided predator-prey system with constant harvest in predators, Appl. Math. Comput., 2015, 250, 193-211. [30] Y. Song, T. Zhang and Y. Peng, Turing-Hopf bifurcation in the reaction-diffusion equations and its applications, Commun. Nonlinear Sci. Numer. Simul., 2016, 33, 229-258. doi: 10.1016/j.cnsns.2015.10.002 [31] Y. Song and X. Zou, Bifurcation analysis of a diffusive ratio-dependent predator-prey model, Nonlinear Dynam., 2014, 78(1), 49-70. doi: 10.1007/s11071-014-1421-2 [32] P. D. N. Srinivasu and B. S. R. V. Prasad, Time optimal control of an additional food provided predator-prey system with applications to pest management and biological conservation, J. Math. Biol., 2010, 60(4), 591-613. doi: 10.1007/s00285-009-0279-2 [33] Y. Su and X. Zou, Transient oscillatory patterns in the diffusive non-local blowfly equation with delay under the zero-flux boundary condition, Nonlinearity, 2014, 27(1), 87-104. doi: 10.1088/0951-7715/27/1/87 [34] C. Wang, R. Liu, J. Shi and C. M. Del Rio, Spatiotemporal mutualistic model of mistletoes and birds, J. Math. Biol., 2014, 68(6), 1479-1520. doi: 10.1007/s00285-013-0664-8 [35] J. Wang and W. Jiang, Hopf-zero bifurcation of a delayed predator-prey model with dormancy of predators, J. Appl. Anal. Comput., 2017, 7(3), 1051-1069. [36] J. Wang, J. Wei and J. Shi, Global bifurcation analysis and pattern formation in homogeneous diffusive predator-prey systems, J. Differential Equations, 2016, 260(4), 3495-3523. doi: 10.1016/j.jde.2015.10.036 [37] S. Wiggins, Introduction to Applied Nonlinear Dynamical Systems and Chaos, Springer Science & Business Media, 2003. [38] J. Wu, Theory and Applications of Partial Functional Differential Equations, Springer, 1996. [39] X. Xu and J. Wei, Turing-Hopf bifurcation of a class of modified Leslie-Gower model with diffusion, Discrete Contin. Dyn. Syst. Ser. B, 2018, 23(2), 765-783. [40] L. Yang, M. Dolnik, A. M. Zhabotinsky and I. R. Epstein, Spatial resonances and superposition patterns in a reaction-diffusion model with interacting turing modes, Phys. Rev. Lett., 2002, 88(20). [41] R. Yang and Y. Song, Spatial resonance and turing-hopf bifurcations in the Gierer-Meinhardt model, Nonlinear Anal. Real World Appl., 2016, 31, 356-387. doi: 10.1016/j.nonrwa.2016.02.006 [42] R. Z. Yang, M. Liu and C. R. Zhang, A delayed-diffusive predator-prey model with a ratio-dependent functional response, Commun. Nonlinear Sci. Numer. Simul., 2017, 53, 94-110. doi: 10.1016/j.cnsns.2017.04.034 [43] F. Yi, J. Wei and J. Shi, Bifurcation and spatiotemporal patterns in a homogeneous diffusive predator-prey system, J. Differential Equations, 2009, 246(5), 1944-1977. doi: 10.1016/j.jde.2008.10.024 [44] R. Yuan, W. Jiang and Y. Wang, Saddle-node-hopf bifurcation in a modified leslie-gower predator-prey model with time-delay and prey harvesting, J. Math. Anal. Appl., 2015, 422(2), 1072-1090. doi: 10.1016/j.jmaa.2014.09.037 [45] T. Zhang, X. Liu, X. Meng and T. Zhang, Spatio-temporal dynamics near the steady state of a planktonic system, Comput. Math. Appl., 2018, 75(12), 4490-4504. doi: 10.1016/j.camwa.2018.03.044 -
-
Figures(10) / Tables(2)
Export File
Citation
Xun Cao, Weihua Jiang. INTERACTIONS OF TURING AND HOPF BIFURCATIONS IN AN ADDITIONAL FOOD PROVIDED DIFFUSIVE PREDATOR-PREY MODEL[J]. Journal of Applied Analysis & Computation, 2019, 9(4): 1277-1304. doi: 10.11948/2156-907X.20180224
Format
Content
DownLoad:
-
Figure 1. Equilibrium bifurcation:
$K=50, \eta=1, \beta=0.8, \gamma=0.4, d=0.2, l=1$ and$\xi=0.1, \xi=0.7, \xi=0.9$ respectively. And,$g$ is bifurcation parameter. System (2.1) might have no positive equilibrium, one positive equilibrium, two positive equilibria or three positive equilibria, depending on different combinations of parameters$\xi, g$ . -
Figure 2. Parameter region of the stability for coexistence equilibrium, and bifurcation curves in
$d_2\text{-}r$ plane. And$HT$ stands for$\left(k_0^*, 0\right)$ -mode Turing-Hopf bifurcation point$\left(d_{k_0^*}^*, r_0\right)$ . Moreover,$TT_k$ represents$(k, k+1)$ -mode Turing-Turing bifurcation point$\left(d_{k, k+1}^*, r_{k, k+1}^*\right)$ for$k\in\mathbb{N}$ , which locates on the critical Turing bifurcation curve$\mathcal{T}$ . -
Figure 3. Parameter region of the stability for coexistence equilibrium
$E_*$ and global bifurcation set in$d_2\text{-}r$ plane. -
Figure 4. Local bifurcation set (Left) and phase portraits (Right) at Turing-Hopf point
$\left(d_{k_0^*}^*, r_0\right)$ . -
Figure 5. Global bifurcation set with local bifurcation curves
$\mathcal{T}_1, \mathcal{T}_2$ at Turing-Hopf bifurcation point$\left(d_{k_0^*}^*, r_0\right)$ . -
Figure 6. For
$(d_2, r)=(1.0290, 0.05690)\in \mathcal{D}_2$ , a spatially homogeneous periodic solution is stable. The initial values are$u(0, x)=0.4058560903+0.1, v(0, x)=1.313932244-0.2$ . -
Figure 7. For
$(d_2, r)=(1.3290, 0.0659)\in\mathcal{D}_4$ , a pair of spatially inhomogeneous periodic solutions are stable. Graphs in the middle reflect transient patterns, which indicate that the spatially homogeneous periodic solution and a pair of spatially inhomogeneous steady states are unstable. -
Figure 8. For
$(d_2, r)=(1.3290, 0.0549)\in\mathcal{D}_6$ , a pair of spatially inhomogeneous steady states are stable. -
Figure 9. For intra-specific competition parameter
$g=0.04$ , there exist multiple stable coexistence equilibria for diffusive system (2.1). -
Figure 10. For the quantity of additional food supply
$\xi=0.5$ , spatially homogeneous steady states of diffusive system (2.1) are stable. The initial values are$u(0, x)=0.4058560903+0.1, v(0, x)=1.313932244-0.2$ .