RECOVERING A SPACE-DEPENDENT SOURCE TERM IN A TIME-FRACTIONAL DIFFUSION WAVE EQUATION

Ting Wei; Xiongbin Yan

doi:10.11948/20180318

2019 Volume 9 Issue 5

Article Contents

Article navigation > Journal of Applied Analysis & Computation > 2019 > 9(5): 1801-1821

Next Article Previous Article

Ting Wei, Xiongbin Yan. RECOVERING A SPACE-DEPENDENT SOURCE TERM IN A TIME-FRACTIONAL DIFFUSION WAVE EQUATION[J]. Journal of Applied Analysis & Computation, 2019, 9(5): 1801-1821. doi: 10.11948/20180318

Citation:

Ting Wei, Xiongbin Yan. RECOVERING A SPACE-DEPENDENT SOURCE TERM IN A TIME-FRACTIONAL DIFFUSION WAVE EQUATION[J]. Journal of Applied Analysis & Computation, 2019, 9(5): 1801-1821. doi: 10.11948/20180318

RECOVERING A SPACE-DEPENDENT SOURCE TERM IN A TIME-FRACTIONAL DIFFUSION WAVE EQUATION

Ting Wei^1, ,,
Xiongbin Yan¹

1.
School of Mathematics and Statistics, Lanzhou University, Lanzhou 730030

Corresponding author: Email address:tingwei@lzu.edu.cn(T. Wei)
Fund Project: This work is supported by the NSF of China (11371181, 11771192) and by Institute of Scientific Computation and Financial data Analysis in Shanghai University of Finance and Economics

Abstract

Abstract

This work is concerned with identifying a space-dependent source function from noisy final time measured data in a time-fractional diffusion wave equation by a variational regularization approach. We provide a regularity of direct problem as well as the existence and uniqueness of adjoint problem. The uniqueness of the inverse source problem is discussed. Using the Tikhonov regularization method, the inverse source problem is formulated into a variational problem and a conjugate gradient algorithm is proposed to solve it. The efficiency and robust of the proposed method are supported by some numerical experiments.
- Inverse source problem /
- Tikhonov regularization /
- conjugate gradient algorithm
MSC: 65M32, 35R11

FullText(HTML)

References

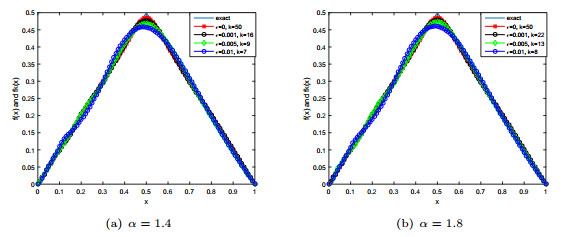
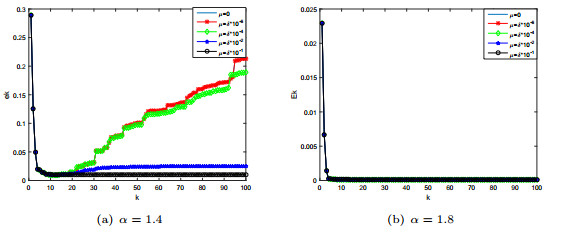
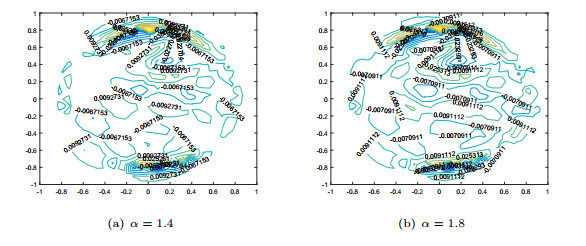
[1]	O. P. Agrawal, Solution for a fractional diffusion-wave equation defined in a bounded domain, Nonlinear Dynam., 2002, 29(1-4), 145-155. Fractional order calculus and its applications. Google Scholar
[2]	B. Berkowitz, H. Scher and S. E. Silliman, Anomalous transport in laboratory-scale, heterogeneous porous media, Water Resources Research, 2000, 36(1), 149-158. doi: 10.1029/1999WR900295 CrossRef Google Scholar
[3]	A. Chen and C. P. Li, Numerical solution of fractional diffusion-wave equation, Numer. Funct. Anal. Optim., 2016, 37(1), 19-39. Google Scholar
[4]	G. Chi, G. Li and X. Jia, Numerical inversions of a source term in the FAD with a Dirichlet boundary condition using final observations, Comput. Math. Appl., 2011, 62(4), 1619-1626. doi: 10.1016/j.camwa.2011.02.029 CrossRef Google Scholar
[5]	H. Y. Dai, L. L. Wei and X. D. Zhang, Numerical algorithm based on an implicit fully discrete local discontinuous Galerkin method for the fractional diffusion-wave equation, Numer. Algorithms, 2014, 67(4), 845-862. doi: 10.1007/s11075-014-9827-y CrossRef Google Scholar
[6]	R. Du, W. R. Cao and Z. Z. Sun, A compact difference scheme for the fractional diffusion-wave equation, Appl. Math. Model., 2010, 34(10), 2998-3007. doi: 10.1016/j.apm.2010.01.008 CrossRef Google Scholar
[7]	H. W. Engl, M. Hanke and A. Neubauer, Regularization of inverse problems, 375 of Mathematics and its Applications, Kluwer Academic Publishers Group, Dordrecht, 1996. Google Scholar
[8]	K. Fujishiro and Y. Kian, Determination of time dependent factors of coefficients in fractional diffusion equations, Math. Control Relat. Fields, 2016, 6(2), 251-269. doi: 10.3934/mcrf.2016003 CrossRef Google Scholar
[9]	D. Fujiwara, Concrete characterization of the domains of fractional powers of some elliptic differential operators of the second order, Proc. Japan Acad., 1967, 43(2), 82-86. doi: 10.3792/pja/1195521686 CrossRef Google Scholar
[10]	D. J. Jiang, Z. Y. Li, Y. K. Liu and M. Yamamoto, Weak unique continuation property and a related inverse source problem for time-fractional diffusion-advection equations, Inverse Problems, 2017, 33(5), 055013, 22. doi: 10.1088/1361-6420/aa58d1 CrossRef Google Scholar
[11]	H. Jiang, F. Liu, I. Turner and K. Burrage, Analytical solutions for the multi-term time-fractional diffusion-wave/diffusion equations in a finite domain, Comput. Math. Appl., 2012, 64(10), 3377-3388. doi: 10.1016/j.camwa.2012.02.042 CrossRef Google Scholar
[12]	Y. J. Jiang and J. T. Ma, High-order finite element methods for time-fractional partial differential equations, Journal of Computational and Applied Mathematics, 2011, 235(11), 3285-3290. doi: 10.1016/j.cam.2011.01.011 CrossRef Google Scholar
[13]	B. T. Jin, R. Lazarov, Y. K. Liu and Z. Zhou, The Galerkin finite element method for a multi-term time-fractional diffusion equation, J. Comput. Phys., 2015, 281, 825-843. doi: 10.1016/j.jcp.2014.10.051 CrossRef Google Scholar
[14]	Y. Kian, L. Oksanen, E. Soccorsi and M. Yamamoto, Global uniqueness in an inverse problem for time fractional diffusion equations, J. Differential Equations, 2018, 264(2), 1146-1170. doi: 10.1016/j.jde.2017.09.032 CrossRef Google Scholar
[15]	Y. Kian and M. Yamamoto, On existence and uniqueness of solutions for semilinear fractional wave equations, Fract. Calc. Appl. Anal., 2017, 20(1), 117-138. Google Scholar
[16]	A. Kilbas, H. Srivastava and J. Trujillo, Theory and applications of fractional differential equations, Elsevier, Amsterdam, 2006. Google Scholar
[17]	C. P. Li and F. H. Zeng, Finite difference methods for fractional differential equations, Internat. J. Bifur. Chaos Appl. Sci. Engrg., 2012, 22(4), 1230014, 28. doi: 10.1142/S0218127412300145 CrossRef Google Scholar
[18]	L. M. Li, D. Xu and M. Luo, Alternating direction implicit Galerkin finite element method for the two-dimensional fractional diffusion-wave equation, J. Comput. Phys., 2013, 255, 471-485. doi: 10.1016/j.jcp.2013.08.031 CrossRef Google Scholar
[19]	Y. K. Liu, W. Rundell and M. Yamamoto, Strong maximum principle for fractional diffusion equations and an application to an inverse source problem, Fract. Calc. Appl. Anal., 2016, 19(4), 888-906. Google Scholar
[20]	A. Lopushansky and H. Lopushanska, Inverse source Cauchy problem for a time fractional diffusion-wave equation with distributions, Electron. J. Differential Equations, 2017, Paper No. 182, 14. Google Scholar
[21]	Y. Luchko, Maximum principle for the generalized time-fractional diffusion equation, J. Math. Anal. Appl., 2009, 351(1), 218-223. Google Scholar
[22]	Y. Luchko, Some uniqueness and existence results for the initial-boundary-value problems for the generalized time-fractional diffusion equation, Comput. Math. Appl., 2010, 59(5), 1766-1772. doi: 10.1016/j.camwa.2009.08.015 CrossRef Google Scholar
[23]	R. Metzler and J. Klafter, The random walk's guide to anomalous diffusion: a fractional dynamics approach, Phys. Rep., 2000, 339(1), 1-77. Google Scholar
[24]	D. A. Murio, Implicit finite difference approximation for time fractional diffusion equations, Comput. Math. Appl., 2008, 56(4), 1138-1145. doi: 10.1016/j.camwa.2008.02.015 CrossRef Google Scholar
[25]	A. Pazy, Semigroups of linear operators and applications to partial differential equations, Springer-Verlag, Berlin, 1983. Google Scholar
[26]	I. Podlubny, Fractional differential equations, Academic Press, San Diego, 1999. Google Scholar
[27]	Z. S. Ruan, Z. J. Yang and X. L. Lu, An inverse source problem with sparsity constraint for the time-fractional diffusion equation, Adv. Appl. Math. Mech., 2016, 8(1), 1-18. doi: 10.4208/aamm.2014.m722 CrossRef Google Scholar
[28]	K. Sakamoto and M. Yamamoto, Initial value/boundary value problems for fractional diffusion-wave equations and applications to some inverse problems, J. Math. Anal. Appl., 2011, 382(1), 426-447. Google Scholar
[29]	M. Slodička and K. Šišková, An inverse source problem in a semilinear time-fractional diffusion equation, Comput. Math. Appl., 2016, 72(6), 1655-1669. Google Scholar
[30]	Z. Z. Sun and X. N. Wu, A fully discrete difference scheme for a diffusion-wave system, Appl. Numer. Math., 2006, 56(2), 193-209. doi: 10.1016/j.apnum.2005.03.003 CrossRef Google Scholar
[31]	K. Šišková and M. Slodička, Recognition of a time-dependent source in a time-fractional wave equation, Appl. Numer. Math., 2017, 121, 1-17. Google Scholar
[32]	W. Y. Wang, M. Yamamoto and B. Han, Numerical method in reproducing kernel space for an inverse source problem for the fractional diffusion equation, Inverse Prob., 2013, 29(9), 095009. doi: 10.1088/0266-5611/29/9/095009 CrossRef Google Scholar
[33]	T. Wei, X. L. Li and Y. S. Li, An inverse time-dependent source problem for a time-fractional diffusion equation, Inverse Problems, 2016, 32(8), 085003, 24. doi: 10.1088/0266-5611/32/8/085003 CrossRef Google Scholar
[34]	T. Wei and J. G. Wang, A modified quasi-boundary value method for an inverse source problem of the time-fractional diffusion equation, Applied Numerical Mathematics, 2014, 78(4), 95-111. Google Scholar
[35]	X. B. Yan and T. Wei, Inverse space-dependent source problem for a time-fractional diffusion equation by an adjoint problem approach, J. Inverse Ill-Posed Probl., 2019, 27(1), 1-16. doi: 10.1515/jiip-2017-0091 CrossRef Google Scholar
[36]	F. H. Zeng, Second-order stable finite difference schemes for the time-fractional diffusion-wave equation, J. Sci. Comput., 2015, 65(1), 411-430. doi: 10.1007/s10915-014-9966-2 CrossRef Google Scholar
[37]	Y. Zhang and X. Xu, Inverse source problem for a fractional diffusion equation, Inverse Prob., 2011, 27(3), 035010. doi: 10.1088/0266-5611/27/3/035010 CrossRef Google Scholar