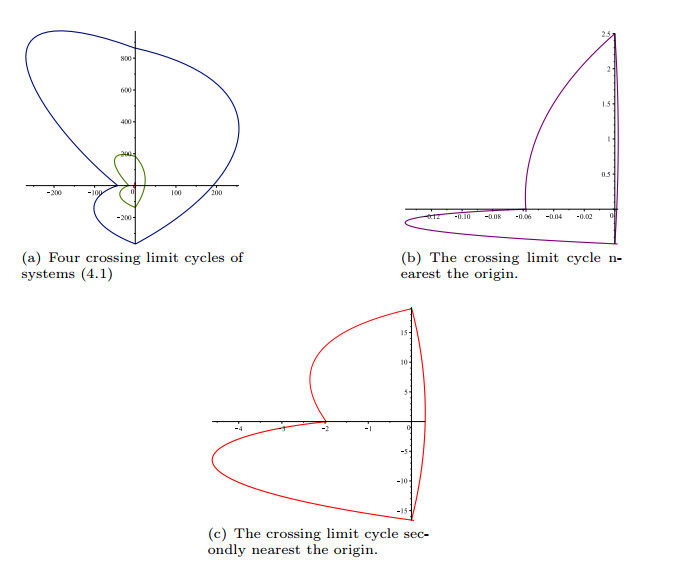
|
[1]
|
A. Amador, E. Freire, E. Ponce and J. Ros, On Discontinuous Piecewise Linear Models for Memristor Oscillators, Internat. J. Bifur. Chaos Appl. Sci. Engrg., 2017, 27, 1730022. doi: 10.1142/S0218127417300221
CrossRef Google Scholar
|
|
[2]
|
A. Andronov, A. Vitt and S. Khaikin, Theory of oscillations, Pergamon Press, Oxford, 1996.
Google Scholar
|
|
[3]
|
P. Cardin and J. Torregrosa, Limit cycles in planar piecewise linear differential systems with nonregular separtion line, Phys. D, 2016, 337, 67-82. doi: 10.1016/j.physd.2016.07.008
CrossRef Google Scholar
|
|
[4]
|
R. Euzébio and J. Llibre, On the number of limit cycles in discontinuous piecewise linear differential systems with two pieces separated by a straight line, J. Math. Anal. Appl., 2015, 424, 475-486. doi: 10.1016/j.jmaa.2014.10.077
CrossRef Google Scholar
|
|
[5]
|
E. Freire, E. Ponce, F. Rodrigo and F. Torres, Bifurcation sets of continuous piecewise linear systems with two zones, Internat. J. Bifur. Chaos Appl. Sci. Engrg., 1998, 8, 2073-2097. doi: 10.1142/S0218127498001728
CrossRef Google Scholar
|
|
[6]
|
E. Freire, E. Ponce and F. Torres, Cononical discontinuous planar piecewise linear systems, SIAM J. Appl. Dyn. Syst., 2012, 11, 181-211. doi: 10.1137/11083928X
CrossRef Google Scholar
|
|
[7]
|
E. Freire, E. Ponce and F. Torres, The discontinuous matching of two planar linear foci can have three nested crossing limit cycles, Publications Matemàtiques, 2014, 221-253.
Google Scholar
|
|
[8]
|
E. Freire, E. Ponce and F. Torres, A general mechanism to generate three limit cycles in planar Filippov systems with two zones, Nonlinear Dynam., 2014, 78, 251-263. doi: 10.1007/s11071-014-1437-7
CrossRef Google Scholar
|
|
[9]
|
E. Gu, Bifurcations and Chaos for 2D Discontinuous Dynamical Model of Financial Markets, Internat. J. Bifur. Chaos Appl. Sci. Engrg., 2017, 27, 1750185. doi: 10.1142/S0218127417501851
CrossRef Google Scholar
|
|
[10]
|
M. Han and W. Zhang, On Hopf bifurcation in non-smooth planar systems, J. Differential Equations, 2010, 248, 2399-2416. doi: 10.1016/j.jde.2009.10.002
CrossRef Google Scholar
|
|
[11]
|
S. Huan and X. Yang, The number of limit cycles in general planar piecewise linear systems, Discrete Contin. Dynam. Systems, 2012, 32, 2147-2164. doi: 10.3934/dcds.2012.32.2147
CrossRef Google Scholar
|
|
[12]
|
S. Huan and X. Yang, On the number of limit cycles in general planar piecewise linear systems of node-node types, J. Math. Anal. Appl., 2013, 411, 340-353.
Google Scholar
|
|
[13]
|
S. Huan and X. Yang, Existence of limit cycles in general planar piecewise linear systems of saddle-saddle dynamics, Nonlinear Anal., 2013, 92, 82-95. doi: 10.1016/j.na.2013.06.017
CrossRef Google Scholar
|
|
[14]
|
Y. Kuznetsov, S. Rinaldi and A. Gragnani, One-parameter bifurcations in planar Filippov systems, Internat. J. Bifur. Chaos Appl. Sci. Engrg., 2003, 13, 2157-2188. doi: 10.1142/S0218127403007874
CrossRef Google Scholar
|
|
[15]
|
J. Llibre and E. Ponce, Three limit cycles in discontinuous piecewise linear differential systems with two zones, Dyn. Contin. Discrete Impuls. Syst. Ser. B Appl. Algorithms, 2012, 19, 325-335.
Google Scholar
|
|
[16]
|
J. Llibre, M.A. Teixeira and J. Torregrosa, Lower bounds for the maximum number of limit cycles of discontinuous piecewise linear differential systems with a sstraight line of separaation, Internat. J. Bifur. Chaos Appl. Sci. Engrg., 2013, 23, 135006.
Google Scholar
|
|
[17]
|
J. Llibre, M. Ordóñz and E. Ponce, On the existence and uniqueness of limit cycles in a planar piecewise linear systems without symmetry, Nonlinear Analysis Series B: Real World Applications, 2013, 14, 2002-2012.
Google Scholar
|
|
[18]
|
J. Llibre, J. Medrado and O. Ramírez, Limit cycles of planar piecewise linear differential systems defined on two sectors, (Preprint submitted to Elsevier, November 15, 2016).
Google Scholar
|
|
[19]
|
J. Llibre, D. Novaes and M. Teixeira, Limit cycles bifurcating from the periodic orbits of a discontinuous piecewise linear differentiable center with two zones, Internat. J. Bifur. Chaos Appl. Sci. Engrg., 2015, 25, 1550144. doi: 10.1142/S0218127415501448
CrossRef Google Scholar
|
|
[20]
|
J. Llibre, D. Novaes and M. Teixeira, Maximum number of limit cycles for certain piecewise linear dynamical systems, Nonlinear Dynam., 2015, 82(3), 1159-1175. doi: 10.1007/s11071-015-2223-x
CrossRef Google Scholar
|
|
[21]
|
J. Llibre, and M. A. Teixeira, Piecewise linear differential systems without equilibria produce limit cycles? Nonlinear Dynam., 2017, 88, 157-164. doi: 10.1007/s11071-016-3236-9
CrossRef Google Scholar
|
|
[22]
|
J. Llibre, and M. A. Teixeira, Piecewise linear differential systems with only centers can create limit cycles? Nonlinear Dynam., 2018, 91, 249-255. doi: 10.1007/s11071-017-3866-6
CrossRef Google Scholar
|
|
[23]
|
R. Lum and L. Chua, Global properties of continuous piecewise-linear vector fields, Part I: Simplest case in $\mathbb{{R}} ^{2}$, Int. J. Circuit Theory Appl., 1991, 19, 251-307. doi: 10.1002/cta.4490190305
CrossRef Google Scholar
|
|
[24]
|
J. Stoer and R. Burlish, Introduction to numerical analysis, Springer-Verlag, New York, 1980.
Google Scholar
|
|
[25]
|
Q. Zhao, J. Yu, Limit cycles of a class of discontinuous planar piecewise linear systems with three regions of Y -type, J. Qual. Theory Dyn. Syst., 2019. DOI: https://doi.org/10.1007/s12346-019-00326-8.
Google Scholar
|




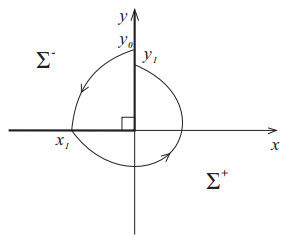

 DownLoad:
DownLoad: