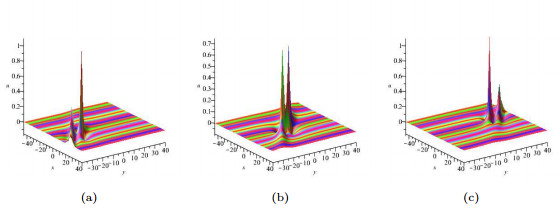
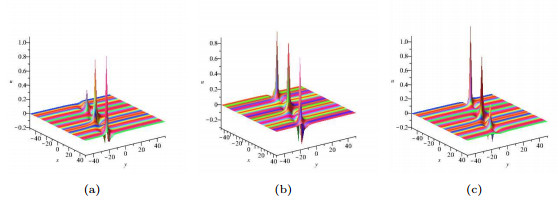
|
[1]
|
M. J. Ablowitz and P. A. Clarkson, Solitons, Nonlinear Evolution Equations and Inverse Scattering, Cambridge University Press, Cambridge, 1991.
Google Scholar
|
|
[2]
|
M. J. Ablowitz and J. Satsuma, Solitons and rational solutions of nonlinear evolution equations, J. Math. Phys., 1978, 19(10), 2180–2186. doi: 10.1063/1.523550
CrossRef Google Scholar
|
|
[3]
|
G. W. Bluman and S. Kumei, Symmetries and differential equations, Springer-Verlag, New York, 1989.
Google Scholar
|
|
[4]
|
Y. L. Cao, J. S. He and D. Mihalache, Families of exact solutions of a new extended (2+1)-dimensional Boussinesq equation, Nonlinear Dyn., 2018, 91(4), 2593–2605.
Google Scholar
|
|
[5]
|
M. J. Dong, S. F. Tian, X. W. Yan and L. Zou, Nonlocal symmetries, conservation laws and interaction solutions for the classical Boussinesq-Burgers equation, Nonlinear Dyn., 2018, 95(1), 273–291.
Google Scholar
|
|
[6]
|
M. J. Dong, S. F. Tian, X. B. Wang and T. T. Zhang, Lump-type solutions and interaction solutions in the (3+1)-dimensional potential Yu-Toda-Sasa-Fukuyama equation, Anal. Math. Phys., 2019, 9(3), 1511–1523. doi: 10.1007/s13324-018-0258-0
CrossRef Google Scholar
|
|
[7]
|
M. J. Dong, S. F. Tian, X. W. Yan and L. Zou, Solitary waves, homoclinic breather waves and rogue waves of the (3+1)-dimensional Hirota bilinear equation, Comput. Math. Appl., 2018, 75(3), 957–964.
Google Scholar
|
|
[8]
|
L. L. Feng, S. F. Tian and T. T. Zhang, Solitary wave, breather wave and rogue wave solutions of an inhomogeneous fifth-order nonlinear Schrödinger equation from Heisenberg ferromagnetism, Rocky Mountain J. Math., 2019, 49(1), 29–45.
Google Scholar
|
|
[9]
|
C. H. Gu, H. S. Hu and Z. X. Zhou, Darboux Transformation in Soliton Theory and its Geometric Applications, Shanghai Scientific and Technical Publishers, Shanghai, 1999.
Google Scholar
|
|
[10]
|
D. Guo, S. F. Tian and T. T. Zhang, Integrability, soliton solutions and modulation instability analysis of a (2+ 1)-dimensional nonlinear Heisenberg ferromagnetic spin chain equation, Comput. Math. Appl., 2019, 77(3), 770–778.
Google Scholar
|
|
[11]
|
D. Guo, S. F. Tian, T. T. Zhang and J. Li, Modulation instability analysis and soliton solutions of an integrable coupled nonlinear Schrödinger system, Nonlinear Dyn., 2018, 94(4), 2749–2761. doi: 10.1007/s11071-018-4522-5
CrossRef Google Scholar
|
|
[12]
|
J. Hietarinta, Hirota's bilinear method and soliton solutions, Phys. AUC, 2005, 15(1), 31–37.
Google Scholar
|
|
[13]
|
R. Hirota, The Direct Method in Soliton Theory, Cambridge University Press, 2004.
Google Scholar
|
|
[14]
|
D. J. Kaup, The lump solutions and the bäcklund transformation for the three-dimensional three-wave resonant interaction, J. Math. Phys., 1981, 22(6), 1176–1181. doi: 10.1063/1.525042
CrossRef Google Scholar
|
|
[15]
|
J. B. Li and Z. J. Qiao, Explicit soliton solutions of the Kaup-Kupershmidt equation through the dynamical system approach, J. Appl. Anal. Comput., 2011, 1(2), 243–250.
Google Scholar
|
|
[16]
|
X. Lü and W. X. Ma, Study of lump dynamics based on a dimensionally reduced Hirota bilinear equation, Nonlinear Dyn., 2016, 85(2), 1217–1222.
Google Scholar
|
|
[17]
|
W. X. Ma, Lump solutions to the Kadomtsev-Petviashvili equation, Phys. Lett. A, 2015, 379(36), 1975–1978. doi: 10.1016/j.physleta.2015.06.061
CrossRef Google Scholar
|
|
[18]
|
W. X. Ma, Y. Zhou and R. Dougherty, Lump-type solutions to nonlinear differential equations derived from generalized bilinear equations, Int. J. Modern Phys. B, 2016, 30(28n29), 1640018. doi: 10.1142/S021797921640018X
CrossRef Google Scholar
|
|
[19]
|
W. X. Ma, Z. Y. Qin and X. Lü, Lump solutions to dimensionally reduced p-gKP and p-gBKP equations, Nonlinear Dyn., 2016, 84(2), 923–931.
Google Scholar
|
|
[20]
|
W. X. Ma and Y. Zhou, Lump solutions to nonlinear partial differential equations via Hirota bilinear forms, J. Differential Equations, 2018, 264(4), 2633–2659. doi: 10.1016/j.jde.2017.10.033
CrossRef Google Scholar
|
|
[21]
|
S. V. Manakov, V. E. Zakharov, L. A. Bordag, A. R. Its and V. B. Matveev, Two-dimensional solitons of the kadomtsev-petviashvili equation and their interaction, Phys. Lett. A, 1977, 63(3), 205–206.
Google Scholar
|
|
[22]
|
V. B. Matveev and M. A. Salle, Darboux Transformations and Solitons, Springer, Berlin, 1991.
Google Scholar
|
|
[23]
|
W. Q. Peng, S. F. Tian, L. Zou and T. T. Zhang, Characteristics of the solitary waves and lump waves with interaction phenomena in a (2+1)-dimensional generalized Caudrey-Dodd-Gibbon-Kotera-Sawada equation, Nonlinear Dyn., 2018, 93(4), 1841–1851.
Google Scholar
|
|
[24]
|
W. Q. Peng, S. F. Tian and T. T. Zhang, Breather waves and rational solutions in the (3+1)-dimensional Boiti-Leon-Manna-Pempinelli equation, Comput. Math. Appl., 2019, 77(3), 715–723.
Google Scholar
|
|
[25]
|
W. Q. Peng, S. F. Tian and T. T. Zhang, Dynamics of breather waves and higher-order rogue waves in a coupled nonlinear Schrödinger equation, EPL, 2018, 123(5), 50005. doi: 10.1209/0295-5075/123/50005
CrossRef Google Scholar
|
|
[26]
|
W. Q. Peng, S. F. Tian and T. T. Zhang, On the Breather Waves, Rogue Waves and Solitary Waves to a Generalized (2+1)-dimensional Caudrey-Dodd-Gibbon-Kotera-Sawada Equation, Filomat, 2018, 32(14), 4959–4969. doi: 10.2298/FIL1814959P
CrossRef Google Scholar
|
|
[27]
|
C. Qian, J. G. Rao, Y. B. Liu and J. S. He, Rogue Waves in the Three-Dimensional Kadomtsev-Petviashvili Equation, Chin. Phys. Lett., 2016, 33(11), 110201. doi: 10.1088/0256-307X/33/11/110201
CrossRef Google Scholar
|
|
[28]
|
C. Y. Qin, S. F. Tian, L. Zou and T. T. Zhang, Lie symmetry analysis, conservation laws and exact solutions of the generalized time fractional Burgers equation, J. Appl. Anal. Comput., 2018, 8(6), 1727–1746.
Google Scholar
|
|
[29]
|
C. Y. Qin, S. F. Tian, X. B. Wang, T. T. Zhang and J. Li, Rogue waves, bright-dark solitons and traveling wave solutions of the (3+1)-dimensional generalized Kadomtsev-Petviashvili equation, Comput. Math. Appl., 2018, 75(12), 4221–4231.
Google Scholar
|
|
[30]
|
C. Y. Qin, S. F. Tian, L. Zou and W. X. Ma, Solitary wave and quasi-periodic wave solutions to a (3+1)-dimensional generalized Calogero-Bogoyavlenskii-Schiff equation, Adv. Appl. Math. Mech., 2018, 10(4), 948–977.
Google Scholar
|
|
[31]
|
J. G. Rao, Y. Cheng and J. S. He, Rational and Semirational Solutions of the Nonlocal Davey-Stewartson Equations, Stud. Appl. Math., 2017, 139(4), 569–598.
Google Scholar
|
|
[32]
|
S. Sahoo and S. S. Ray, The new exact solutions of variant types of time fractional coupled schrödinger equations in plasma physics, J. Appl. Anal. Comput., 2017, 7(3), 824–840.
Google Scholar
|
|
[33]
|
J. Satsuma and M. J. Ablowitz, Two-dimensional lumps in nonlinear dispersive systems, J. Math. Phys., 1979, 20(7), 1496–1503.
Google Scholar
|
|
[34]
|
D. R. Solli, C. Ropers, P. Koonath and B. Jalali, Optical rogue waves, Nature, 2007, 450(7172), 1054–1057. doi: 10.1038/nature06402
CrossRef Google Scholar
|
|
[35]
|
D. R. Solli, C. Ropers and B. Jalali, Active Control of Rogue Waves for Stimulated Supercontinuum Generation, Phys. Rev. Lett., 2008, 101(23), 233902. doi: 10.1103/PhysRevLett.101.233902
CrossRef Google Scholar
|
|
[36]
|
S. F. Tian, Initial-boundary value problems for the coupled modified Korteweg-de Vries equation on the interval, Commun. Pure Appl. Anal., 2018, 17(3), 923–957.
Google Scholar
|
|
[37]
|
S. F. Tian, Asymptotic behavior of a weakly dissipative modified two-component Dullin-Gottwald-Holm system, Appl. Math. Lett., 2018, 83, 65–72. doi: 10.1016/j.aml.2018.03.019
CrossRef Google Scholar
|
|
[38]
|
S. F. Tian, Initial-boundary value problems for the general coupled nonlinear Schrödinger equation on the interval via the Fokas method, J. Differential Equations, 2017, 262(1), 506–558.
Google Scholar
|
|
[39]
|
S. F. Tian, Infinite propagation speed of a weakly dissipative modified two-component Dullin-Gottwald-Holm system, Appl. Math. Lett., 2019, 89, 1-7. doi: 10.1016/j.aml.2018.09.010
CrossRef Google Scholar
|
|
[40]
|
S. F. Tian and H. Q. Zhang, Riemann theta functions periodic wave solutions and rational characteristics for the nonlinear equations, J. Math. Anal. Appl., 2010, 371(2), 585–608.
Google Scholar
|
|
[41]
|
S. F. Tian and H. Q. Zhang, On the integrability of a generalized variable-coefficient Kadomtsev-Petviashvili equation, J. Phys. A: Math. Theor., 2012, 45(5), 055203. doi: 10.1088/1751-8113/45/5/055203
CrossRef Google Scholar
|
|
[42]
|
S. F. Tian and H. Q. Zhang, On the Integrability of a Generalized Variable-Coefficient Forced Korteweg-de Vries Equation in Fluids, Stud. Appl. Math., 2014, 132(3), 212–246.
Google Scholar
|
|
[43]
|
S. F. Tian and T. T. Zhang, Long-time asymptotic behavior for the Gerdjikov-Ivanov type of derivative nonlinear Schrödinger equation with time-periodic boundary condition, Proc. Amer. Math. Soc., 2018, 146(4), 1713–1729.
Google Scholar
|
|
[44]
|
D. S. Wang, B. L. Guo and X. L. Wang, Long-time asymptotics of the focusing Kundu-Eckhaus equation with nonzero boundary conditions, J. Differential Equations, 2019, 266(9), 5209–5253. doi: 10.1016/j.jde.2018.10.053
CrossRef Google Scholar
|
|
[45]
|
D. S. Wang, J. Liu and L. Z. Wang, Non-autonomous matter-wave solitons in hybrid atomic-molecular Bose-Einstein condensates with tunable interactions and harmonic potential, Phys. Lett. A, 2018, 382(12), 799–805. doi: 10.1016/j.physleta.2018.01.014
CrossRef Google Scholar
|
|
[46]
|
D. S. Wang, Y. R. Shi, W. X. Feng and L. Wen, Dynamical and energetic instabilities of F = 2 spinor Bose-Einstein condensates in an optical lattice, Physica D, 2017, 351, 30–41.
Google Scholar
|
|
[47]
|
D. S. Wang and J. Liu, Integrability aspects of some two-component KdV systems, Appl. Math. Lett., 2018, 79, 211–219. doi: 10.1016/j.aml.2017.12.018
CrossRef Google Scholar
|
|
[48]
|
D. S. Wang and X. L. Wang, Long-time asymptotics and the bright N-soliton solutions of the Kundu-Eckhaus equation via the Riemann-Hilbert approach, Nonlinear Anal. Real World Appl., 2018, 41, 334–361. doi: 10.1016/j.nonrwa.2017.10.014
CrossRef Google Scholar
|
|
[49]
|
D. S. Wang, X. G. Li, C. K. Chan and J. Zhou, Double Wronskian solution and soliton properties of the nonisospectral BKP equation, Commun. Theor. Phys., 2016, 65(3), 259–265. doi: 10.1088/0253-6102/65/3/259
CrossRef Google Scholar
|
|
[50]
|
X. B. Wang, S. F. Tian and T. T. Zhang, Characteristics of the breather and rogue waves in a (2+1)-dimensional nonlinear Schrödinger equation, Proc. Amer. Math. Soc., 2018, 146(8), 3353–3365. doi: 10.1090/proc/13765
CrossRef Google Scholar
|
|
[51]
|
X. B. Wang and S. F. Tian, Lie symmetry analysis, conservation laws and analytical solutions of the time-fractional thin-film equation, Comput. Appl. Math., 2018, 37(5), 6270–6282. doi: 10.1007/s40314-018-0699-y
CrossRef Google Scholar
|
|
[52]
|
X. B. Wang, S. F. Tian, C. Y. Qin and T. T. Zhang, Characteristics of the breathers, rogue waves and solitary waves in a generalized (2+1)-dimensional Boussinesq equation, EPL, 2016, 115(1), 10002. doi: 10.1209/0295-5075/115/10002
CrossRef Google Scholar
|
|
[53]
|
H. Wang, S. Tian, T. Zhang and Y. Chen, Lump wave and hybrid solutions of a generalized (3+1)-dimensional nonlinear wave equation in liquid with gas bubbles, Front. Math. China, 2019, 14(3), 631–643.
Google Scholar
|
|
[54]
|
A. M. Wazwaz and G. Q. Xu, Negative-ordermodified KdV equations: multiple soliton and multiple singular soliton solutions, Math. Methods Appl. Sci., 2016, 39(4), 661–667.
Google Scholar
|
|
[55]
|
M. J. Xu, S. F. Tian, J. M. Tu, P. L. Ma and T. T. Zhang, On quasiperiodic wave solutions and integrability to a generalized (2+1)-dimensional Korteweg-de Vries equation, Nonlinear Dyn., 2015, 82(4), 2031–2049. doi: 10.1007/s11071-015-2297-5
CrossRef Google Scholar
|
|
[56]
|
X. W. Yan, S. F. Tian, M. J. Dong, X. B. Wang and T. T. Zhang, Nonlocal Symmetries, Conservation Laws and Interaction Solutions of the Generalised Dispersive Modified Benjamin-Bona-Mahony Equation, Z. Naturforsch A, 2018, 73(5), 399–405. doi: 10.1515/zna-2017-0436
CrossRef Google Scholar
|
|
[57]
|
X. W. Yan, S. F. Tian, X. B. Wang and T. T. Zhang, Solitons to rogue waves transition, lump solutions and interaction solutions for the (3+1)-dimensional generalized B-type Kadomtsev-Petviashvili equation in fluid dynamics, Int. J. Comput. Math., 2019, 96(9), 1839–1848. doi: 10.1080/00207160.2018.1535708
CrossRef Google Scholar
|
|
[58]
|
J. Y. Yang and W. X. Ma, Abundant lump-type solutions of the Jimbo-Miwa equation in (3+1)-dimensions, Comput. Math. Appl., 2017, 73(2), 220–225.
Google Scholar
|
|
[59]
|
J. Y. Yang, W. X. Ma and Z. Y. Qin, Lump and lump-soliton solutions to the (2+1)-dimensional Ito equation, Anal. Math. Phys., 2018, 8(3), 427–436. doi: 10.1007/s13324-017-0181-9
CrossRef Google Scholar
|
|
[60]
|
J. P. Yu and Y. L. Sun, Study of lump solutions to dimensionally reduced generalized KP equations, Nonlinear Dyn., 2017, 87(4), 2755–2763. doi: 10.1007/s11071-016-3225-z
CrossRef Google Scholar
|
|
[61]
|
Y. Zhang, Y. P. Liu and X. Y. Tang, M-lump and interactive solutions to a (3+1)-dimensional nonlinear system, Nonlinear Dyn., 2018, 93(4), 2533–2541. doi: 10.1007/s11071-018-4340-9
CrossRef Google Scholar
|




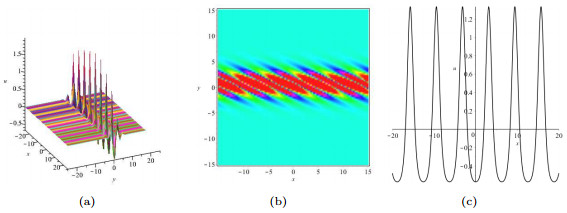

 DownLoad:
DownLoad: