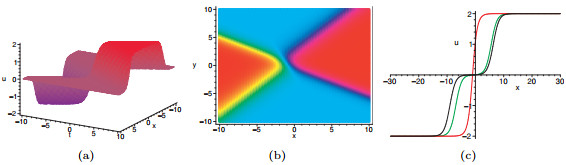
|
[1]
|
M. Boiti, L. Martina and O.K. Pashaev, F. Pempineli, Dynamics of multidimensional solitons, Phys. Lett. A, 1991, 160, 55-63. doi: 10.1016/0375-9601(91)90205-M
CrossRef Google Scholar
|
|
[2]
|
C. Dai and W. Huang, Multi-rogue wave and multi-braather solutions in PTsymmertric coupled waveguides, Appl. Math. Lett., 2014, 32, 35-40. doi: 10.1016/j.aml.2014.02.013
CrossRef Google Scholar
|
|
[3]
|
M. Dong, S. Tian, X. Yan and L. Zou, Solitary waves, homoclinic breather waves and rogue waves of the (3+1)-dimensional Hirota bilinear equation, Comput. Math. Appl., 2018, 75(3), 957-964. doi: 10.1016/j.camwa.2017.10.037
CrossRef Google Scholar
|
|
[4]
|
G. Ebadi, N. Y. Fard, A. H. Bhrawy, S. Kumar, H. Triki, A. Yildirim and A. Biswas, solitons and other solutions to the (3+1)-dimensional extended kadomtsev-petviashvili equation with power law nonlinearity, Rom. Rep. Phys., 2013, 65, 27-62.
Google Scholar
|
|
[5]
|
L. Feng and T. Zhang, Breather wave, rouge wave and solitary wave solutions of a coupled nonlinear Schödinger equation, Appl. Math. Lett., 2018, 78, 133-140. doi: 10.1016/j.aml.2017.11.011
CrossRef Google Scholar
|
|
[6]
|
D. Guo, S. Tian and T. Zhang, Integrability, soliton solutions and modulation instability analysis of a (2+1)-dimensional nonlinear Heisenberg ferromagnetic spin chain equation, Comput. Math. Appl., 2019, 77(3), 770-778. doi: 10.1016/j.camwa.2018.10.017
CrossRef Google Scholar
|
|
[7]
|
Y. Gurefe, E. Misirli, Y. Pandir, A. Sonmezoglu and M. Ekici, New Exact Solutions of the Davey-Stewartson Equation with Power-Law Nonlinearity, Bull. Malays. Math. Sci. Soc., 2015, 38, 1223-1234. doi: 10.1007/s40840-014-0075-z
CrossRef Google Scholar
|
|
[8]
|
C. Hamner, J. Chang, P. Engels and M. A. Hoefer, Generation of Dark-Bright Soliton Trains in Superfluid-Superfluid Counterflow, Phys. Rev. Lett., 2010, 106, 065302.
Google Scholar
|
|
[9]
|
H. Jiang, J. Xiang, C. Dai and Y. Wang, Nonautonomous bright soliton solutions on continuous wave and cnoidal wave backgrounds in blood vessels, Nonlinear Dyn., 2014, 75, 201-207. doi: 10.1007/s11071-013-1058-6
CrossRef Google Scholar
|
|
[10]
|
M. Jimbo and T. Miwa, Solitons and infinite dimensional lie algebras, Publ. Res. Inst. Mathe. Sci., 1983, 19, 943-1001. doi: 10.2977/prims/1195182017
CrossRef Google Scholar
|
|
[11]
|
R. S. Johnson and S. Thompson, A solution of the inverse scattering problem for the Kadomtsev-Petviashvili equation by the method of separation of variables, Phys. Lett., 1978, 66(4), 279-281. doi: 10.1016/0375-9601(78)90236-0
CrossRef Google Scholar
|
|
[12]
|
P. G. Korteweg and G. Vries, On the change of form of long waves advancing in a rectangular canal and on a new type of long stationary waves, Philos Mag., 1895, 39, 422-443. doi: 10.1080/14786449508620739
CrossRef Google Scholar
|
|
[13]
|
J. Li and Z. Qiao, Explicit soliton solutions of the Kaup-Kupershmidt equation through the dynamical system approach, J. Appl. Anal. Comput., 2011, 1(2), 243-250.
Google Scholar
|
|
[14]
|
C. Li, J. He and K. Porseizan, Rogue waves of the Hirota and the Maxwell-Bloch equations, Phys. Rev. E, 2013, 87, 012913. doi: 10.1103/PhysRevE.87.012913
CrossRef Google Scholar
|
|
[15]
|
W. Ma, Abundant lumps and their interaction solutions of (3+1)-dimensional linear PDEs, J. Geom. Phys., 2018, 133, 10-16. doi: 10.1016/j.geomphys.2018.07.003
CrossRef Google Scholar
|
|
[16]
|
W. Ma and Y. Zhou, Lump solutions to nonlinear partial differential equations via Hirota bilinear forms, J. Differential Equations, 2018, 264, 2633-2659. doi: 10.1016/j.jde.2017.10.033
CrossRef Google Scholar
|
|
[17]
|
W. Ma, Lump solutions to the Kadomtsev-Petviashvili equation, Phys. Lett. A, 2015, 379, 1975-1978. doi: 10.1016/j.physleta.2015.06.061
CrossRef Google Scholar
|
|
[18]
|
W. Ma, Z. Qin and X. La, Lump soltions to dimensionally reduced p-gKP and p-gBKP equations, Nonlinear Dyn., 2016, 84(2), 923-931. doi: 10.1007/s11071-015-2539-6
CrossRef Google Scholar
|
|
[19]
|
W. Ma and J. Yang, Lump solutions to the BKP equation by symbolic computation, Int. J. Mod. Phys. B, 2016, 30, 1640028. doi: 10.1142/S0217979216400282
CrossRef Google Scholar
|
|
[20]
|
W. Ma and Z. Zhu, Solving the (3+1)-dimensional generalized KP and BKP equations by the multiple exp-function algorithm, Appl. Math. Comput., 2012, 218, 11871-11879.
Google Scholar
|
|
[21]
|
W. Ma, Y. Zhang, Y. Tang and J. Tu, Hirota bilinear equations with linear subspaces of solutions, Appl. Math. Comput., 2012, 218(13), 7174-7183.
Google Scholar
|
|
[22]
|
W. Ma and L. Zhang, Lump solutions with higher-order rational dispersion relations, Pramana. J. Phys., 2020, 94, ID0043.
Google Scholar
|
|
[23]
|
W. Ma, Lump and interaction solutions to linear PDEs in (2+1) dimensions via symbolic computation, Modern Phys. Lett. B, 2019, 33(36), 1950457. doi: 10.1142/S0217984919504578
CrossRef Google Scholar
|
|
[24]
|
L. Na, Bäcklund transformation and multi-soliton solutions for the (3+1)- dimensional BKP equation with Bell polynomials and symbolic computation, Nonlinear Dyn., 2015, 82(1-2), 311-318. doi: 10.1007/s11071-015-2159-1
CrossRef Google Scholar
|
|
[25]
|
W. Peng, S. Tian and T. Zhang, On the Breather Waves, Rogue Waves and Solitary Waves to a Generalized (2+1)-dimensional Caudrey-Dodd-GibbonKotera-Sawada Equation, Filomat, 2018, 32(14), 4959-4969. doi: 10.2298/FIL1814959P
CrossRef Google Scholar
|
|
[26]
|
W. Peng, S. Tian and T. Zhang, Dynamics of breather waves and higher-order rogue waves in a coupled nonlinear Schrodinger equation, EPL (Europhysics Letters), 2018, 123(5), 50005. doi: 10.1209/0295-5075/123/50005
CrossRef Google Scholar
|
|
[27]
|
C. Qin, S. Tian, L. Zou and T. Zhang, Lie symmetry analysis, conservation laws and exact solutions of the generalized time fractional Burgers equation, J. Appl. Anal. Comput., 2018, 8(6), 1727-1746.
Google Scholar
|
|
[28]
|
C. Qin, S. Tian, L. Zou and T. Zhang, Lie symmetry analysis, conservation laws and exact solutions of fourth-order time fractional Burgers equation, J. Appl. Anal. Comput., 2018, 8(6), 1727-1746.
Google Scholar
|
|
[29]
|
C. Qin, S. Tian, L. Zou and W. Ma, Solitary Wave and Quasi-Periodic Wave Solutions to a (3+1)-Dimensional Generalized Calogero-Bogoyavlenskii-Schiff Equation, Adv. Appl. Math. Mech., 2018, 10, 948-977. doi: 10.4208/aamm.OA-2017-0220
CrossRef Google Scholar
|
|
[30]
|
S. Tian and H. Zhang, On the Integrability of a Generalized Variable-Coefficient Forced Korteweg-de Vries Equation in Fluids, Stud. Appl. Math., 2014, 132, 212-246. doi: 10.1111/sapm.12026
CrossRef Google Scholar
|
|
[31]
|
X. Wang, S. Tian, C. Qin and T. Zhang, Characteristics of the breather and rogue waves in a (2+1)-dimensional nonlinear Schrödinger equation, Proc. Amer. Math. Soc., 2018, 146(8), 3353-3365. doi: 10.1090/proc/13765
CrossRef Google Scholar
|
|
[32]
|
X. Wang, S. Tian, L. Zou and T. Zhang, Dynamics of solitary waves and periodic waves in a (3+1)-dimensional nonlinear evolution equation, East Asian J. Appl. Math., 2018, 8(3), 477-497. doi: 10.4208/eajam.221017.250218
CrossRef Google Scholar
|
|
[33]
|
X. Wang and S. Tian, Lie symmetry analysis, conservation laws and analytical solutions of the time-fractional thin-film equation, Comput. Appl. Math., 2018, 37(5), 6270-6282. doi: 10.1007/s40314-018-0699-y
CrossRef Google Scholar
|
|
[34]
|
X. Wang, S. Tian, C. Qin and T. Zhang, Characteristics of the breathers, rogue waves and solitary waves in a generalized(2+1)-dimensional Boussinesq equation, EPL(Europhysics Letters), 2016, 115, 10002. doi: 10.1209/0295-5075/115/10002
CrossRef Google Scholar
|
|
[35]
|
A. M. Wazwaz, Two forms of (3+1)dimensional B-type Kadomtsev-Petviashvili equation: multiple soliton solutions, Phys. Scr., 2012, 86(3), 035007. doi: 10.1088/0031-8949/86/03/035007
CrossRef Google Scholar
|
|
[36]
|
A. M. Wazwaz and G. Xu, Negative-order modified KdV equations: multiple soliton and multiple singular soliton solution, Math. Methods Appl. Sci., 2016, 39, 661-667. doi: 10.1002/mma.3507
CrossRef Google Scholar
|
|
[37]
|
X. Wen, Construction of new exact rational form non-travelling wave solutions to the (2+1)-dimensional generalized BroerKaup system, Appl. Math. Comput., 2010, 217, 1367-1375.
Google Scholar
|
|
[38]
|
J. Yang, W. Ma and Z. Qin, Abundant mixed lump-soliton solutions of the BKP equation, East Asian J. Appl. Math., 2018, 8(2), 224-232. doi: 10.4208/eajam.210917.051217a
CrossRef Google Scholar
|
|
[39]
|
X. Zhang and Y. Chen, Rogue wave and a pair of resonance stripe solitons to a reduced (3+1)-dimensional Jimbo-Miwa equation, Commun. Nonlinear Sci. Numer. Simul., 2017, 52, 24-31. doi: 10.1016/j.cnsns.2017.03.021
CrossRef Google Scholar
|




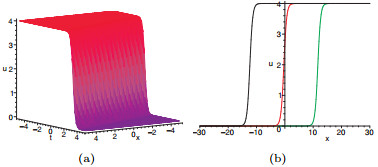

 DownLoad:
DownLoad: