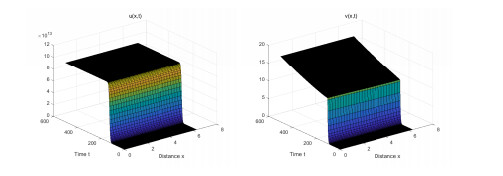
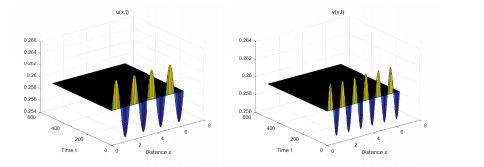
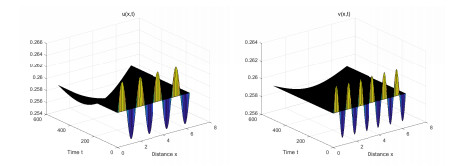
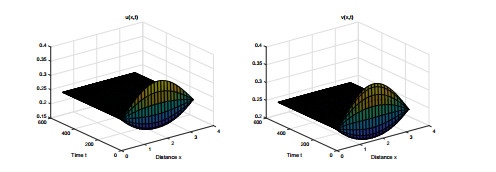
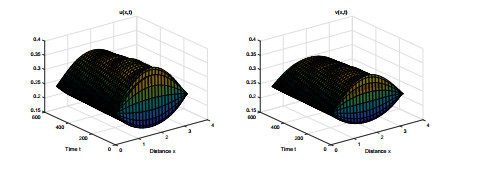
|
[1]
|
R. Arditi and L. R. Ginzburg, Coupling in predator-prey dynamics: Ratio-dependence, Journal of Theoretical Biology, 1989, 139(3), 311-326. doi: 10.1016/S0022-5193(89)80211-5
CrossRef Google Scholar
|
|
[2]
|
R. Arditi, L. R. Ginzburg and H. R. Akcakaya, Variation in plankton densities among lakes: a case for ratio-dependent models, The American Naturalist, 1991, 138(5), 1287-1296. doi: 10.1086/285286
CrossRef Google Scholar
|
|
[3]
|
R. Arditi, N. Perrin and H. Saiah, Functional responses and heterogeneities: an experimental test with cladocerans, Oikos., 1991, 60(1), 69-75. doi: 10.2307/3544994
CrossRef Google Scholar
|
|
[4]
|
R. Arditi and H. Saiah, Empirical evidence of the role of heterogeneity in ratio-dependent consumption, Ecology, 1992, 73(5), 1544-1551. doi: 10.2307/1940007
CrossRef Google Scholar
|
|
[5]
|
H. Beirao da Veiga, On the global regularity for singular p-systems under non-homogeneous dirichlet boundary conditions, Journal of Mathematical Analysis and Applications, 2013, 398, 527-533. doi: 10.1016/j.jmaa.2012.08.058
CrossRef Google Scholar
|
|
[6]
|
P. A. Braza, The bifurcation structure of the holling-tanner model for predator-prey interactions using two-timing, SIAM J. Appl. Math., 2003, 63(3), 889-904. doi: 10.1137/S0036139901393494
CrossRef Google Scholar
|
|
[7]
|
R. S. Cantrell and C. Cosner, Spatial Ecology via Reaction-Diffusion Equations, Academic Press, New York, 2003.
Google Scholar
|
|
[8]
|
C. Chen and L. Hung, Nonexistence of traveling wave solutions, exact and semi-exact traveling wave solutions for diffusive lotka-volterra systems of three competing species, Comm. Pure Appl Anal., 2017, 15(4), 1451-1469.
Google Scholar
|
|
[9]
|
S. Chen, J. Shi and J. Wei, Global stability and hopf bifurcation in a delayed diffusive leslie-gower predator-prey system, International Journal of Bifurcation and Chaos, 2012. 10.1142/S0218127412500617.
Google Scholar
|
|
[10]
|
L. Ciannelli, M. Hunsicker, M. Hidalgo et al., Theory, consequences and evidence of eroding population spatial structure in harvested marine fishes, Mar Ecol Prog Ser., 2013, 480, 227-243. doi: 10.3354/meps10067
CrossRef Google Scholar
|
|
[11]
|
J. B. Collings, Bifurcation and stability analysis of a temperature-dependent mite predator-prey interaction model incorporating a prey refuge, Bull. Math. Biol., 1995, 57(1), 63-76. doi: 10.1016/0092-8240(94)00024-7
CrossRef Google Scholar
|
|
[12]
|
Y. Du and S. B. Hsu, A diffusive predator-prey model in heterogeneous environment, Journal of Differential Equations, 2004, 203(2), 331-364. doi: 10.1016/j.jde.2004.05.010
CrossRef Google Scholar
|
|
[13]
|
A. P. Gutierrez, Physiological basis of ratio-dependent predator-prey theory: the metabolic pool model as a paradigm, Ecology, 1992, 73(5), 1552-1563. doi: 10.2307/1940008
CrossRef Google Scholar
|
|
[14]
|
J. Ha and S. Nakagiri, Damped sine-gordon equations with non-homogeneous dirichlet boundary conditions, Journal of Mathematical Analysis and Applications, 2001, 263(2), 708-720.
Google Scholar
|
|
[15]
|
M. Han, Bifurcation theory and methods of dynamical systems, Science Press, Beijing, 1995.
Google Scholar
|
|
[16]
|
M. P. Hassell, The dynamics of arthropod predator-prey systems, Monogr. Popul. Biol., 1978, 65(13), 1-237.
Google Scholar
|
|
[17]
|
L. Hauser and G. R. Carvalho, Paradigm shifts in marine fisheries genetics: ugly hypotheses slain by beautiful facts, Fish Fish., 2010, 9(4), 333-362.
Google Scholar
|
|
[18]
|
C. S. Holling, The functional response of predators to prey density and its role in mimicry and population regulation, Mem. Ent. Soc. Can., 1965, 97(45), 1-60.
Google Scholar
|
|
[19]
|
C. S. Holling, The functional response of invertebrate predators to prey density, Mem. Ent. Soc. Can., 1966, 98(48), 1-86.
Google Scholar
|
|
[20]
|
S. B. Hsu and T. W. Huang, Global stability for a class of predator-prey systems, SIAM J. Appl. Math., 1995, 55(3), 763-783. doi: 10.1137/S0036139993253201
CrossRef Google Scholar
|
|
[21]
|
W. Ko and K. Ryu, Non-constant positive steady-states of a diffusive predator-prey system in homogeneous environment, J. Math. Anal. Appl., 2007, 327, 539-549. doi: 10.1016/j.jmaa.2006.04.077
CrossRef Google Scholar
|
|
[22]
|
A. Korobeinikov, A lyapunov function for leslie-gower predator-prey models, Appl. Math. Lett., 2001, 14(6), 697-699. doi: 10.1016/S0893-9659(01)80029-X
CrossRef Google Scholar
|
|
[23]
|
Y. Lou and W. Ni, Diffusion, self-diffusion and cross-diffusion, J. Differential Equations, 1996, 131, 79-131. doi: 10.1006/jdeq.1996.0157
CrossRef Google Scholar
|
|
[24]
|
R. M. May, Limit cycles in predator-prey communities, Science, 1972, 177, 900-902. doi: 10.1126/science.177.4052.900
CrossRef Google Scholar
|
|
[25]
|
R. M. May, Stability and Complexity in Model Ecosystems, Academic Press, New York, 1973.
Google Scholar
|
|
[26]
|
R. Peng and M. Wang, Positive steady sates of the holling-tanner prey-predator model with diffusion, Proceedings of the Royal Society of Edinburgh, 2005, 135(1), 149-164. doi: 10.1017/S0308210500003814
CrossRef Google Scholar
|
|
[27]
|
H. Reiss, G. Hoarau and M. Dickey-Collas, Genetic population structure of marine fish: mismatch between biological and fisheries management units, Fish Fish., 2009, 10(4), 361-395. doi: 10.1111/j.1467-2979.2008.00324.x
CrossRef Google Scholar
|
|
[28]
|
D. Robichaud and G. Rose, Migratory behaviour and range in atlantic cod: inference from a century of tagging, Fish Fish., 2004, 5(3), 185-214. doi: 10.1111/j.1467-2679.2004.00141.x
CrossRef Google Scholar
|
|
[29]
|
E. Saez and E. Gonzalez-Olivares, Dynamics of a predator-prey model, Siam Journal on Applied Mathematics, 1999, 59(5), 1867-1878. doi: 10.1137/S0036139997318457
CrossRef Google Scholar
|
|
[30]
|
T. Saha and C. Chakrabarti, Dynamical analysis of a delayed ratio-dependent holling-tanner predator-prey model, Journal of Mathematical Analysis and Applications, 2009, 358(2), 389-402. doi: 10.1016/j.jmaa.2009.03.072
CrossRef Google Scholar
|
|
[31]
|
D. E. Schindler, H. Ray and C. Brandon, Population diversity and the portfolio effect in an exploited species, Nature, 2010, 465(7298), 609-612. doi: 10.1038/nature09060
CrossRef Google Scholar
|
|
[32]
|
S. M. Sohel Rana, Bifurcations and chaos control in a discrete-time predator-prey system of leslie type, Journal of Applied Analysis and Computation, 2019, 9(1), 31-44.
Google Scholar
|
|
[33]
|
J. Song, M. Hu and Y. Bai, Dynamic analysis of a non-autonomous ratio-dependent predator-prey model with additional food, Journal of Applied Analysis and Computation, 2018, 8(6), 1893-1909.
Google Scholar
|
|
[34]
|
N. Stenseth, P. Jorde and K. Chan, Ecological and genetic impact of atlantic cod larval drift in the skagerrak, Proc Roy Soc Biol., 2006, 273(1590), 1085-1092. doi: 10.1098/rspb.2005.3290
CrossRef Google Scholar
|
|
[35]
|
J. T. Tanner, The stability and the intrinsic growth rates of prey and predator populations, Ecology, 1975, 56(4), 855-867. doi: 10.2307/1936296
CrossRef Google Scholar
|
|
[36]
|
J. Wang, J. Shi and J. Wei, Dynamics and pattern formation in a diffusive predator-prey system with strong allee effect in prey, J. Differential Equations, 2011, 251(4), 1276-1304.
Google Scholar
|
|
[37]
|
J. Wang, J. Shi and J. Wei, Global bifurcation analysis and pattern formation in homogeneous diffusive predator-prey systems, J. Differential Equations, 2016, 260(4), 3495-3523. doi: 10.1016/j.jde.2015.10.036
CrossRef Google Scholar
|
|
[38]
|
L. Wang, J. Watmough and F. Yu, Bifurcation analysis and transient spatio-temporal dynamics for a diffusive plant-herbivore system with dirichlet boundary conditions, Mathematical Biosciences and Engineering, 2015, 12(4), 699-715. doi: 10.3934/mbe.2015.12.699
CrossRef Google Scholar
|
|
[39]
|
D. J. Wollkind, J. B. Collings and J. A. Logan, Metastability in a temperature-dependent model system for predator-prey mite outbreak interactions on fruit trees, Bull. Math. Biol., 1988, 50(4), 379-409. doi: 10.1016/S0092-8240(88)90005-5
CrossRef Google Scholar
|
|
[40]
|
S. Wu, J. Wang and J. Shi, Dynamics and pattern formation of a diffusive predator-prey model with predator-taxis, Math. Models. Methods. Appl. Sci., 2018, 28(11), 2275-2312. doi: 10.1142/S0218202518400158
CrossRef Google Scholar
|
|
[41]
|
Y. Yamada, Stability of steady states for prey-predator diffusion equations with homogeneous dirichlet conditions, SIAM J. Math. Anal., 1990, 21(2), 327-345. doi: 10.1137/0521018
CrossRef Google Scholar
|
|
[42]
|
F. Yi, J. Wei and J. Shi, Bifurcation and spatiotemporal patterns in a homogeneous diffusive predator-prey system, J. Diff. Eqs., 2009, 246(4), 1944-1977.
Google Scholar
|




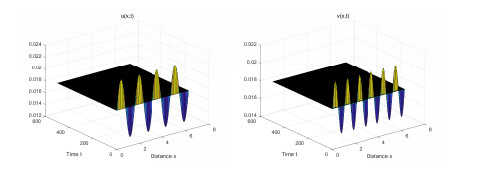

 DownLoad:
DownLoad: