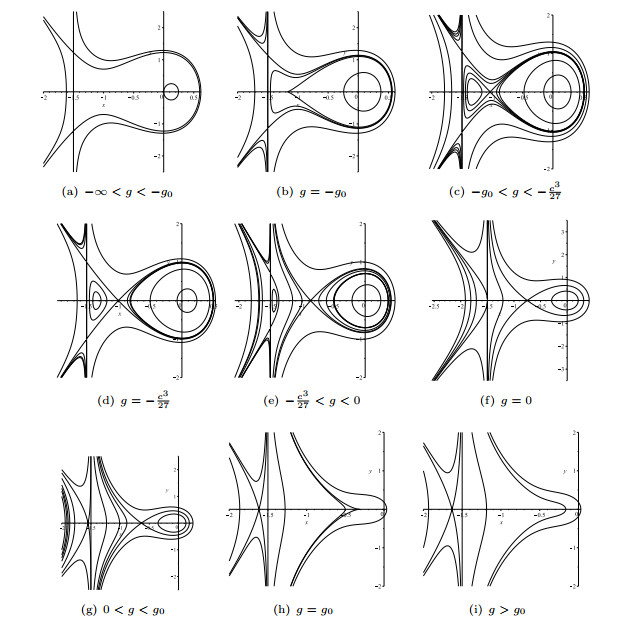
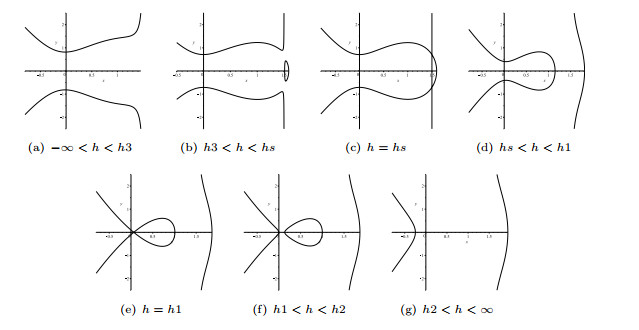
|
[1]
|
M. Antonowicz and A. P. Fordy, Coupled KDV equation with multi-Hamiltonian structures, Physica D, 1987, 28(3), 345-357. doi: 10.1016/0167-2789(87)90023-6
CrossRef Google Scholar
|
|
[2]
|
M. Antonowicz and A. P. Fordy, Factorisation of energy dependent Schrödinger operators: Miura maps and modified systems, Commun. Math. Phys, 1989, 124(3), 465-486.
Google Scholar
|
|
[3]
|
P. F. Byrd and M. D. Fridman, Handbook of Elliptic Integrals for Engineers and Sciensists, Springer, Berlin, 1971.
Google Scholar
|
|
[4]
|
A. N. W. Hone, V. Novikov and C. Verhoeven, An integrable hierarchy with a perturbed H'enon-Heiles system, Inverse Problems, 2006, 22, 2001-2020. doi: 10.1088/0266-5611/22/6/006
CrossRef Google Scholar
|
|
[5]
|
A. N. W. Hone, V. Novikov and C. Verhoeven, An extended Hónon-Heiles system, Physics Letters A, 2008, 372, 1440-1444. doi: 10.1016/j.physleta.2007.09.063
CrossRef Google Scholar
|
|
[6]
|
J. Li, Singular nonlinear travelling wave equations: bifurcations and exact solutions, Science Press, Beijing, 2013.
Google Scholar
|
|
[7]
|
J. Li, W. Zhu and G. Chen, Understanding peakons, periodic peakons and compactons via a shallow water wave equation, Int. J. Bifurcation and Chaos, 2016, 26(12), 1650207. doi: 10.1142/S0218127416502072
CrossRef Google Scholar
|
|
[8]
|
J. Li, Notes on exact traveling wave solutions for a long wave-short wave model, Journal of Applied Analysis and Computation, 2015, 5(1), 138-140.
Google Scholar
|
|
[9]
|
J. Liang and J. Li, Bifurcations and exact solutions of nonlinear Schr$\ddot{o}$dinger equation with an anti-cubic nonlinearity, Journal of Applied Analysis and Computation, 2018, 8(4), 1194-1210.
Google Scholar
|
|
[10]
|
J. Li and G. Chen, On a class of singular nonlinear traveling wave equations, Int. J. Bifurcation and Chaos, 2007, 17(11), 4049-4065. doi: 10.1142/S0218127407019858
CrossRef Google Scholar
|
|
[11]
|
J. Li, J. Wu and H. Zhu, Travelling waves for an integrable higher order KdV type wave equations, Int. J. Bifurcation and Chaos, 2006, 16(8), 2235-2260. doi: 10.1142/S0218127406016033
CrossRef Google Scholar
|
|
[12]
|
A. V. Mikhailov, V. S. Novikov and J. P. Wang, On classification of integrable non-evolutionary equations, Stud. Appl. Math, 2007, 118(4), 419-457.
Google Scholar
|
|
[13]
|
D. Temesgen and J. Li, Existence of kink and unbounded traveling wave solutions of the Casimir equation for the Ito system, Journal of Applied Analysis and Computation, 2017, 7(2), 632-643.
Google Scholar
|
|
[14]
|
D. Temesgen and J. Li, Dynamical behaviour and exact solutions of thirteenth order derivative nonlinear Schr$\ddot{o}$dinger equation, Journal of Applied Analysis and Computation, 2018, 8(1), 250-271.
Google Scholar
|




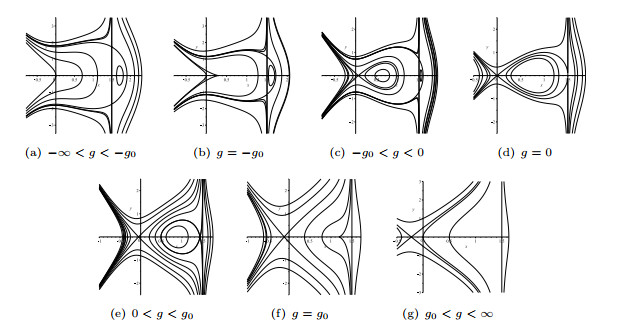

 DownLoad:
DownLoad: