| Citation: |
Asma Issasfa, Ji Lin. LUMP AND MIXED ROGUE-SOLITON SOLUTIONS TO THE 2+1 DIMENSIONAL ABLOWITZ-KAUP-NEWELL-SEGUR EQUATION[J]. Journal of Applied Analysis & Computation, 2020, 10(1): 314-325. doi: 10.11948/20190160
|
LUMP AND MIXED ROGUE-SOLITON SOLUTIONS TO THE 2+1 DIMENSIONAL ABLOWITZ-KAUP-NEWELL-SEGUR EQUATION
-
Abstract
In this paper, the 2+1 dimensional Ablowitz-Kaup-Newell-Segur (AKNS) equation which obtained from the potential Boiti-Leon-Manna-Pempi nelli (pBLMP) equation, is introduced. Through the bilinear method and ansatz technique, the rational solutions consisting of rogue wave and lump soliton solutions are constructed, where we discuss the condition of guaranteeing the positiveness and analyticity of the lump solutions. The collection of a quadratic function with an exponential function describing rationalexponential solutions is proved, the interaction consisting of one lump and one soliton with fission and fusion phenomena. The second kind of interaction comprises the line rogue wave and soliton solution, which is inelastic. With the usage of the extended homoclinic test approach, the homoclinic breather-wave solution is derived. The characteristics of these various solutions are exhibited and illustrated graphically. -
-
References
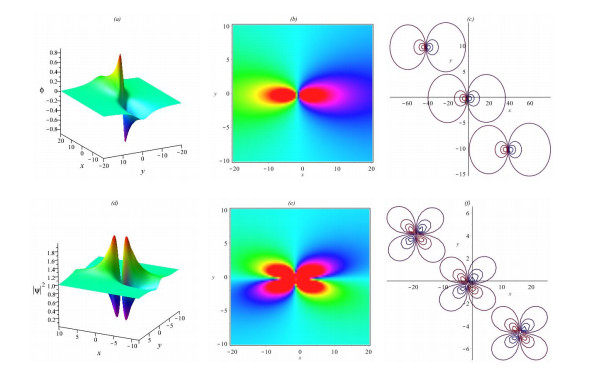
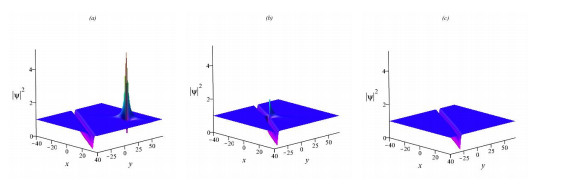
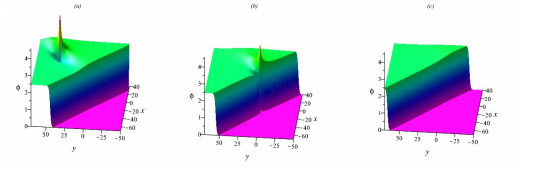
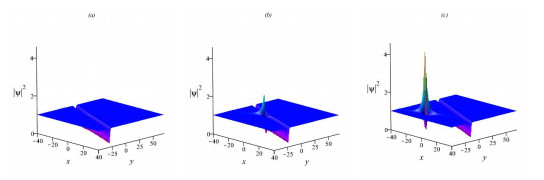
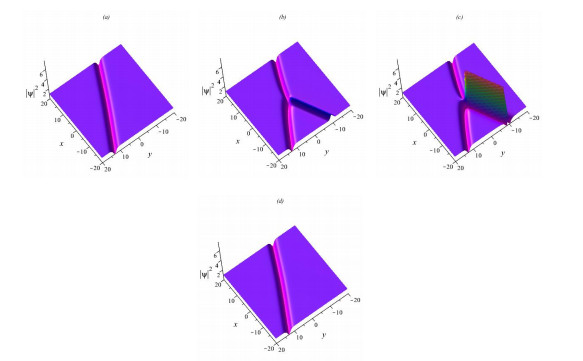
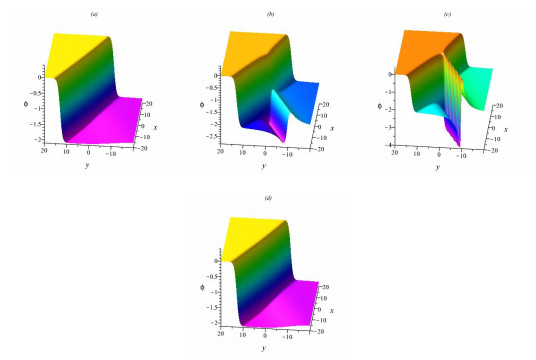
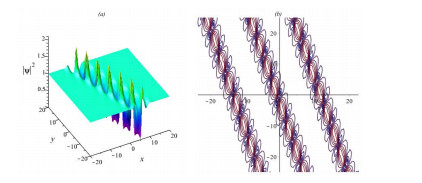
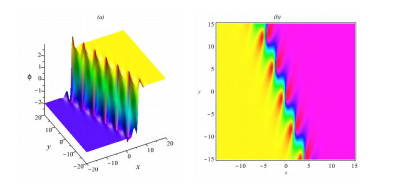
[1] J. Ablowitz, D.J. Kaup, A.C. Newell and H. Segur, The inverse scattering transform-fourier analysis for nonlinear problems, Studies in Applied Mathematics, 1974, 53, 249–315. doi: 10.1002/sapm1974534249 [2] S. Batwa, W.X. Ma, Lump solutions to a (2+1)-dimensional fifth-order KdV-like equation, Advances in Mathematical Physics, 2018, 1–6. [3] Y. Chen, D.J. Zhang and J.B. Bo, New double wronskian solutions of the AKNS equation, Science in China Series A: Mathematics, 2008, 51, 55–69. doi: 10.1007/s11425-007-0165-6 [4] Y. Cui, Z.Q. Lao, Multiple rogue wave and breather solutions for the(3+1)-dimensional KPI equation, Comput. Math. Appl, 1971, 76(5), 1099–1107. [5] C. Freeman, J.J.C. Nimmo, Soliton solutions of the KdV and KP equations: the wronskian technique, Phys. Lett. A, 1983, 95, 1–3. doi: 10.1016/0375-9601(83)90764-8 [6] S. Gardner, J.M. Greene, M.D. Kruskal and R.M. Miura, Method for solving the Korteweg de Vries equation, Phys. Rev. Lett, 1967, 19, 1095–1097. doi: 10.1103/PhysRevLett.19.1095 [7] R. Gilsona, J.J.C. Nimmo, Lump solutions of the BKP equation, Phys. Lett. A., 1990, 147, 472–476. doi: 10.1016/0375-9601(90)90609-R [8] R. Hirota, Exact solution of the Korteweg-de Vries equation for multiple collisions of solitons, Phys. Rev. Lett, 1971, 27, 1192. doi: 10.1103/PhysRevLett.27.1192 [9] R. Hirota, The direct method in soliton theory, Cambridge University Press, Cambridge, UK, 2004. [10] B. Hu, H.Y. Wang, Construction of dKP and BKP equations with self-consistent sources, Inverse Problems, 2006, 22, 1903–1920. doi: 10.1088/0266-5611/22/5/022 [11] L. Ji, Z.N. Zhu, Soliton solutions of an integrable nonlocal modified Korteweg-de Vries equation through inverse scattering transform, J. Math. Anal. Appl, 2017, 453, 973–984. doi: 10.1016/j.jmaa.2017.04.042 [12] J. Kaup, The lump solutions and the Bäcklund transformation for the three-dimensional three-wave resonant interaction, J. Math. Phys., 1981, 22, 1176. doi: 10.1063/1.525042 [13] Z. Qiao, Darboux transformation and explicit solutions for two integrable equations, J. Math. Anal. Appl, 2011, 380, 794–806. doi: 10.1016/j.jmaa.2011.01.078 [14] Y. Lou, X.R. Hu and Y. Chen, Nonlocal symmetries related to Bäcklund transformation and their applications, J. Phys. A: Math. Theor., 2012, 45, 155209. doi: 10.1088/1751-8113/45/15/155209 [15] J. Lin, X.W. Jin, X.L. Gao and S.Y. Lou, Commun.Theor.Phys., 2018, 70, 119. doi: 10.1088/0253-6102/70/2/119 [16] J.q. Lv, S.d. Bilige, Lump solutions of a (2+1)-dimensional bSK equation, Nonlinear. Dyn., 2017, 90, 2119–2124. [17] Y. Lou, X.B. Hu, Infinitely many lax pair and symmetry constraints of the KP equation, J. Math. Phys., 1997, 38, 6407–6427. [18] Y. Lou, C.L. Chen and X.Y. Tang, (2+1)-dimensional (M+N)-component AKNS system: painlevé integrability, infinitely many symmetries, similarity reductions and exact solutions, J. Math. Phys., 2002, 43, 4078–4109. doi: 10.1063/1.1490407 [19] B. Matveev, M.A. Salle, Darboux transformations and solitons, Springer-Verlag, Berlin, 1991. [20] X. Ma, Lump solutions to the Kadomtsev-Petviashvili equation, Phys. Lett. A., 2015, 379, 1975. doi: 10.1016/j.physleta.2015.06.061 [21] C. Ma, A.P. Deng, Lump solution of (2+1)-dimensional Boussinesq equation, Commun. Theor. Phys., 2016, 65, 546–552. doi: 10.1088/0253-6102/65/5/546 [22] C. Nimmo, N.C. Freeman, A method of obtaining the N-soliton solution of the Boussinesq equation in terms of a wronskian, Phys. Lett. A, 1983, 95, 4–6. doi: 10.1016/0375-9601(83)90765-X [23] Y. Ohta, J.K. Yang, Rogue waves in the Davey-Stewartson I equation, Phys. Rev. E., 2012, 86, 036604. doi: 10.1103/PhysRevE.86.036604 [24] O. Roshid, W.X. Ma, Dynamics of mixed lump-solitary waves of an extended (2+1)-dimensional shallow water wave model, Phys. Lett. A., 2018, 382, 3262–3268. doi: 10.1016/j.physleta.2018.09.019 [25] B. Ren, X.j. Xu and J. Lin, Symmetry group and exact solutions for the 2+1 dimensional Ablowitz-Kaup-Newell-Segur equation, J. Math. Phys., 2009, 50, 123505. doi: 10.1063/1.3268588 [26] J. Satsuma, Hirota bilinear method for nonlinear evolution equations, Lect. Notes Phys, 2003, 632, 171–222. [27] J. Satsuma, M. J. Ablowitz, Two-dimensional lumps in nonlinear dispersive systems, J. Math. Phys., 1979, 20, 1496–1503. doi: 10.1063/1.524208 [28] Y. Sun, New exact solutions of the (2+1)-dimensional AKNS equation, J. Appl. Math. Phys, 2015, 3, 1391–1405. doi: 10.4236/jamp.2015.311167 [29] M. Wazwaz, Multiple-soliton solutions for the Boussinesq equation, Appl. Math., 2007, 192, 479–486. [30] Y. Yang, W.X. Ma, Lump solutions to the BKP equation by symbolic computation, Int. J. M. Phys. B., 2016, 30, 28–29. [31] Q. Zhao, W.X. Ma, Mixed lump-kink solutions to the KP equation, Comput. Math. Appl, . 2017, 74, 1399. [32] B. Zhang, W.X. Ma, Mixed lump-kink solutions to the BKP equation, Comput. Math. Appl., 2017, 74, 591. doi: 10.1016/j.camwa.2017.05.010 -
-
Figures(10)
Export File
Citation
Asma Issasfa, Ji Lin. LUMP AND MIXED ROGUE-SOLITON SOLUTIONS TO THE 2+1 DIMENSIONAL ABLOWITZ-KAUP-NEWELL-SEGUR EQUATION[J]. Journal of Applied Analysis & Computation, 2020, 10(1): 314-325. doi: 10.11948/20190160
Format
Content
DownLoad:
- Figure 1. Lump solution of Eq. (1.1) for case1, with the parameters $ a_3 = \frac{1}{2}, a_4 = 0, a_5=1, a_7 = -1, a_8=3, b_3 = \frac{1}{2}, $ at $t=0$, (a) $|\psi|^2$, (b) Density plot of $|\psi|^2$ at $t=0$
- Figure 2. Lump solution of Eq. (1.1) for case1, with the parameters $a_3 = 1, a_4 = 2, a_5=2, a_7 = -1, a_8=3, b_3 = \frac{1}{2}, $ (a) $\phi$, (b) Density plot of $\phi$ at $t=0$, (c) Contour plots of $\phi$ at $t=-80, t=0, t=80$, (d) $|\psi|^2$, (e) Density plot of $|\psi|^2$ at $t=0$, (f) Contour plots of $|\psi|^2$ at $t=-35, t=0, t=35$
- Figure 3. Perspective view of rational-exponential solution $|\psi|^2$ for Eq. (1.1), with the parameter $a_4=1, a_9=2, a_5=0.25, a_8=5, k_1=1.25 (a) t=-30 (b) t=-6 (c) t=20$, in the $(x, y)$-plane
- Figure 4. Perspective view of rational-exponential solution $|\psi|^2$ for Eq. (1.1), with the parameter $a_4=1, a_9=2, a_5=0.25, a_8=5, k_1=-1 (a) t=-30 (b) t=-10 (c) t=20$, in the $(x, y)$-plane
- Figure 5. $|\psi|^2$ for Eq. (1.1), with the parameter $a_4=0.5, a_9=2, a_8=-1.5, k_1=-1, (a) t=-30 (b) t=0 (c) t=3 (d) t=30$, in the $(x, y)$-plane
- Figure 6. Perspective view of rational-exponential solution $|\psi|^2$ for Eq. (1.1), with the parameter $a_4=1, a_9=2, a_5=0.25, a_8=5, k_1=1.25 (a) t=-30 (b) t=-6 (c) t=20$, in the $(x, y)$-plane
-
Figure 7. Perspective view of rational-exponential solution
$ |\psi|^2 $ for Eq. (1.1), with the parameter$ a_4 = 0.5, a_9 = 2, a_8 = -1.5, k_1 = -1, (a) t = -30 (b) t = 0 (c) t = 3 (d) t = 30 $ , in the$ (x, y) $ -plane -
Figure 8. Perspective view of rational-exponential solution
$ \phi $ for Eq. (1.1), with the parameter$ a_4 = 0.5, a_9 = 2, a_8 = -1.5, k_1 = -1, (a) t = -30 (b) t = 0 (c) t = 3 (d) t = 30 $ , in the$ (x, y) $ -plane - Figure 9. Periodic solitary-wave solution for $|\psi|^2$ of Eq. (1.1), with the parameters $ P=1, P_1=-1.5, b_0=-2, $ at $t=1$, (a) plot $|\psi|^2$, (b) Contour plot of $|\psi|^2$ at $t=-10, t=0, t=10$
-
Figure 10. Kinky periodic-wave solution for
$ \phi $ of Eq. (1.1), with the parameters$ P = 1, P_1 = -1.5, b_0 = -2, $ at$ t = 1 $ , (a) plot of$ \phi $ , (b) Density plot of$ \phi $