QUADRATIC HARVESTING DOMINATED OPTIMAL STRATEGY FOR A STOCHASTIC SINGLE-SPECIES MODEL

Dianli Zhao; Haidong Liu; Yanli Zhou; Sanling Yuan

doi:10.11948/20190174

2020 Volume 10 Issue 4

Article Contents

Article navigation > Journal of Applied Analysis & Computation > 2020 > 10(4): 1256-1266

Next Article Previous Article

Dianli Zhao, Haidong Liu, Yanli Zhou, Sanling Yuan. QUADRATIC HARVESTING DOMINATED OPTIMAL STRATEGY FOR A STOCHASTIC SINGLE-SPECIES MODEL[J]. Journal of Applied Analysis & Computation, 2020, 10(4): 1256-1266. doi: 10.11948/20190174

Citation:

Dianli Zhao, Haidong Liu, Yanli Zhou, Sanling Yuan. QUADRATIC HARVESTING DOMINATED OPTIMAL STRATEGY FOR A STOCHASTIC SINGLE-SPECIES MODEL[J]. Journal of Applied Analysis & Computation, 2020, 10(4): 1256-1266. doi: 10.11948/20190174

QUADRATIC HARVESTING DOMINATED OPTIMAL STRATEGY FOR A STOCHASTIC SINGLE-SPECIES MODEL

1.
College of Science, University of Shanghai for Science and Technology, Shanghai 200093, China
2.
School of Mathematical Sciences, Qufu Normal University, Qufu 273165, China
3.
College of Arts and Science, Shanghai University of Medicine and Health Sciences, Shanghai 201318, China

Corresponding author: Email address:Tc_zhaodianli@usst.edu.cn(D. Zhao)

Abstract

Abstract

A stochastic population model with the mixed harvesting strategy is formulated and studied in this paper. Sufficient and necessary conditions for survival of the species are derived firstly. Then, based on the ergodic stationary distribution, the optimal strategy is identified. Results show that the linear harvesting effort threatens to the survival of the species; the quadratic harvesting strategy occupies an absolute advantage in the harvesting and excludes the linear part out of the optimal harvesting strategy. It's interest to see all these occur only in the random environments. Computer simulations are carried out to support the obtained results.
- Stochastic single-species model /
- ergodicity /
- optimal harvesting strategy /
- threshold

FullText(HTML)

References

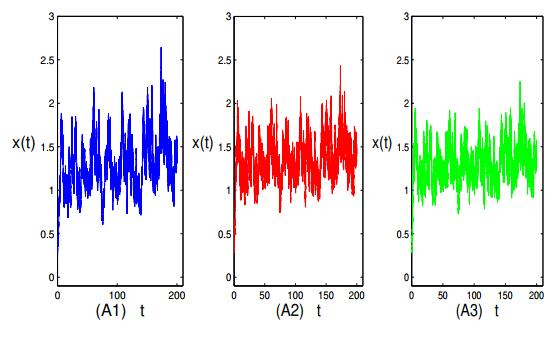
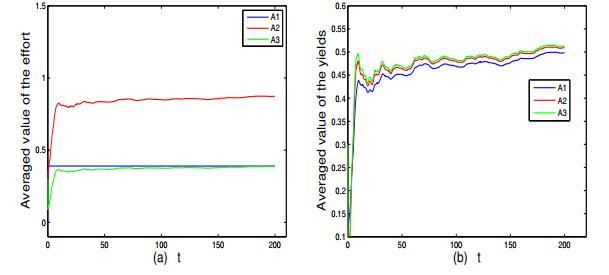
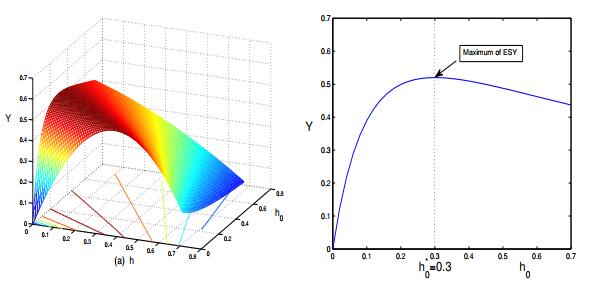
[1]	J. Bao, X. Mao, G. Yin and C. Yuan, Competitive Lotka-Volterra population dynamics with jumps, Nonlinear Anal., 2011, 74, 6601-6616. doi: 10.1016/j.na.2011.06.043 CrossRef Google Scholar
[2]	J. R. Beddington and R. M. May, Harvesting natural populations in a randomly fluctuating environment, Science, 1977, 197, 463-465. doi: 10.1126/science.197.4302.463 CrossRef Google Scholar
[3]	C. W. Clark, Mathematical bioeconomics: the optimal management of renewable resources, Wiley-Interscience, New York, USA, 1976. Google Scholar
[4]	C. W. Clark, Bioeconomic modeling and fisheries management, John Wiley & Sons, New York, USA, 1985. Google Scholar
[5]	J. Geng, M. Liu and Y. Zhang, Stability of a stochastic one-predator-two-prey population model with time delays, Commun. Nonlin. Scien. Numer. Simul., 2017, 53, 65-82. doi: 10.1016/j.cnsns.2017.04.022 CrossRef Google Scholar
[6]	R. P. Gupta, M. Banerjee and P. Chandra, Period doubling cascades of prey-predator model with nonlinear harvesting and control of over exploitation through taxation, Commun. Nonlin. Scien. Numer. Simul., 2014, 19, 2382-2405. doi: 10.1016/j.cnsns.2013.10.033 CrossRef Google Scholar
[7]	R. P. Gupta and P. Chandra, Dynamical properties of a prey-predator-scavenger model with quadratic harvesting, 2017, 49, 202-214. Google Scholar
[8]	D. J. Higham, An algorithmic introduction to numerical simulation of stochastic differential equations, SIAM Rev. 2001, 43, 525-546. doi: 10.1137/S0036144500378302 CrossRef Google Scholar
[9]	D. Li, The stationary distribution and ergodicity of a stochastic generalized logistic system, Statist. Probab. Lett., 2013, 83, 580-583. doi: 10.1016/j.spl.2012.11.006 CrossRef Google Scholar
[10]	M. Li, B. Chen and H. Ye, A bioeconomic differential algebraic predator-prey model with nonlinear prey harvesting, Applied Mathematical Modelling, 2017, 42, 17-28. doi: 10.1016/j.apm.2016.09.029 CrossRef Google Scholar
[11]	W. Li and K. Wang, Optimal harvesting policy for general stochastic Logistic population model, J. Math. Anal. Appl., 2010, 368, 420-428. doi: 10.1016/j.jmaa.2010.04.002 CrossRef Google Scholar
[12]	M. Liu, Optimal harvesting policy of a stochastic predator-prey model with time delay, Appl. Math. Lett., 2015, 48, 102-108. doi: 10.1016/j.aml.2014.10.007 CrossRef Google Scholar
[13]	M. Liu and C. Bai, Optimal harvesting of a stochastic logistic model with time delay, J. Nonlinear Sci., 2015, 25, 277-289. doi: 10.1007/s00332-014-9229-2 CrossRef Google Scholar
[14]	M. Liu and M. Deng, Analysis of a stochastic hybrid population model with Allee effect, Appl. Math. Comp., 2020, 364, 124582.https://doi.org/10.1016/j.amc.2019.124582. doi: 10.1016/j.amc.2019.124582 CrossRef Google Scholar
[15]	L. Liu and X. Meng, Optimal harvesting control and dynamics of two-species stochastic model with delays, Adv Differ Equ., 2017, 2017, 18. https://doi.org/10.1186/s13662-017-1077-6. doi: 10.1186/s13662-017-1077-6 CrossRef Google Scholar
[16]	J. Lv, Y. Zhang and X. Zou, Recurrence and strong stochastic persistence of a stochastic single-species model, Applied Mathematics Letters, 2019, 89, 64-69. doi: 10.1016/j.aml.2018.09.007 CrossRef Google Scholar
[17]	X. Mao and C. Yuan, Stochastic differential equations with Markovian switching, Imperial College Press, London, 2006. Google Scholar
[18]	R. M. May, J. R. Beddington, J. W. Horwood and J. G. Shepherd, Exploiting natural populations in an uncertain world, Math. Biosci., 1978, 42, 219-252. doi: 10.1016/0025-5564(78)90097-4 CrossRef Google Scholar
[19]	P. Panja, S. K. Mondal and D. K. Jana, Effects of toxicants on Phytoplankton-Zooplankton-Fish dynamics and harvesting, Chaos, Solitons and Fractals, 2017, 104, 389-399. doi: 10.1016/j.chaos.2017.08.036 CrossRef Google Scholar
[20]	J. G. Shepherd and J. W. Horwood, The sensitivity of exploited populations to environmental noise, and the implications for management, J. Cons. Int. Explor. Mer., 1979, 38, 318-323. doi: 10.1093/icesjms/38.3.318 CrossRef Google Scholar
[21]	R. K. Upadhyay, P. Roy and J. Datta, Complex dynamics of ecological systems under nonlinear harvesting: Hopf bifurcation and Turing instability, 2015, 79, 2251-2270. Google Scholar
[22]	H. Wang and M. Liu, Stationary distribution of a stochastic hybrid phytoplankton-zooplankton model with toxin-producing phytoplankton, Appl. Math. Lett., 2020, 101, 106077. https://doi.org/10.1016/j.aml.2019.106077. doi: 10.1016/j.aml.2019.106077 CrossRef Google Scholar
[23]	D. Zhao, S. Yuan and H. Liu, Stochastic dynamics of the delayed chemostat with Levy noises, Intern. J. Biomath., 2019, 12, 1-32. Google Scholar
[24]	D. Zhao and H. Liu, Coexistence in a two species chemostat model with Markov switchings, Appl. Math. Lett., 2019, 94, 266-271. doi: 10.1016/j.aml.2019.03.005 CrossRef Google Scholar
[25]	C. Zhu and G. Yin, Asympotic properties of hybrid diffusion systems, SIAM J. Contrl Optim., 2007, 46, 1155-1179. doi: 10.1137/060649343 CrossRef Google Scholar
[26]	X. Zou and K. Wang, Optimal harvesting for a stochastic regime-switching logistic diffusion system with jumps, Nonlinear Anal. Hybrid Syst., 2014, 13, 32-44. doi: 10.1016/j.nahs.2014.01.001 CrossRef Google Scholar