A NEW THIRD ORDER EXPONENTIALLY FITTED DISCRETIZATION FOR THE SOLUTION OF NON-LINEAR TWO POINT BOUNDARY VALUE PROBLEMS ON A GRADED MESH

R. K. Mohanty; Geetan Manchanda; Gunjan Khurana; Arshad Khan

doi:10.11948/20190187

2020 Volume 10 Issue 5

Article Contents

Article navigation > Journal of Applied Analysis & Computation > 2020 > 10(5): 1741-1770

Next Article Previous Article

R. K. Mohanty, Geetan Manchanda, Gunjan Khurana, Arshad Khan. A NEW THIRD ORDER EXPONENTIALLY FITTED DISCRETIZATION FOR THE SOLUTION OF NON-LINEAR TWO POINT BOUNDARY VALUE PROBLEMS ON A GRADED MESH[J]. Journal of Applied Analysis & Computation, 2020, 10(5): 1741-1770. doi: 10.11948/20190187

Citation:

R. K. Mohanty, Geetan Manchanda, Gunjan Khurana, Arshad Khan. A NEW THIRD ORDER EXPONENTIALLY FITTED DISCRETIZATION FOR THE SOLUTION OF NON-LINEAR TWO POINT BOUNDARY VALUE PROBLEMS ON A GRADED MESH[J]. Journal of Applied Analysis & Computation, 2020, 10(5): 1741-1770. doi: 10.11948/20190187

A NEW THIRD ORDER EXPONENTIALLY FITTED DISCRETIZATION FOR THE SOLUTION OF NON-LINEAR TWO POINT BOUNDARY VALUE PROBLEMS ON A GRADED MESH

1.
Department of Applied Mathematics, South Asian University, Akbar Bhawan Chanakyapuri, 110021 New Delhi, India
2.
Department of Mathematics, Faculty of Natural Sciences, Jamia Millia Islamia, Jamia Nagar, 110025 New Delhi, India
3.
Department of Mathematics, IP College for Women, Civil Lines, 110054 New Delhi, India

Corresponding author: Email: rmohanty@sau.ac.in(R. K. Mohanty)

Abstract

Abstract

This paper puts forward a novel graded mesh implicit scheme resting upon full step discretization of order three for computation of non-linear two point boundary value problems. The suggested method is compact and employs three nodal points for the unknown function $ u(x) $ in spatial axis. We have also performed error analysis of the cited method. The given method was tried (implemented) upon multiple problems in Cartesian and Polar coordinates with extremely favorable outcomes. This method, though meant for scalar equations, was further extended to compute the vector equations of two point nonlinear boundary value problems. To check the validity of the proposed scheme, we applied it to multiple problems and obtained supporting numerical computations.
MSC: 65L10, 65L12, 65L20

FullText(HTML)

References

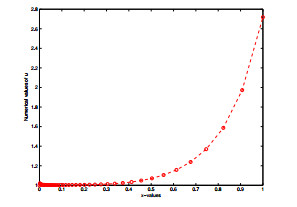
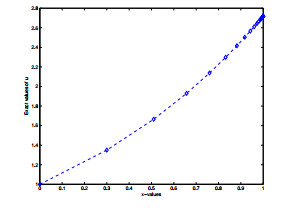
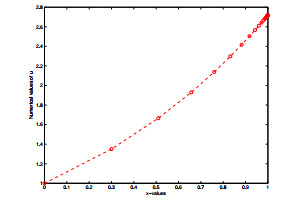
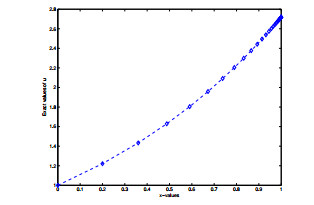
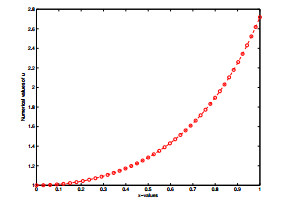
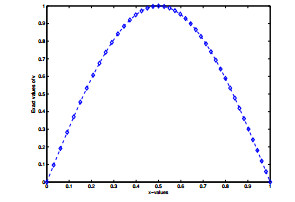
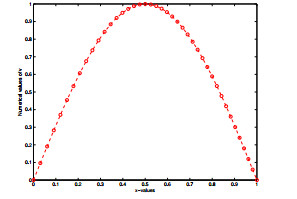
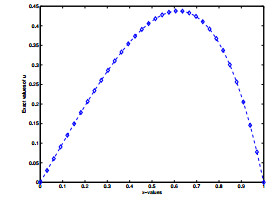
[1]	T. Aziz and M. Kumar, A fourth-order finite-difference method based on nonuniform mesh for a class of singular two-point boundary value problems, J. Comput. Appl. Math., 2001, 136(1-2), 337-342. doi: 10.1016/S0377-0427(00)00624-5 CrossRef Google Scholar
[2]	J. Cartwright, V. Eguiluz, E. Hernandez-Garcia and O. Piro, Dynamics of elastic excitable media, Internat. J. Bifur. Chaos Appl. Sci. Engrg., 1999, 9(11), 2197-2202. doi: 10.1142/S0218127499001620 CrossRef Google Scholar
[3]	M. M. Chawla, A fourth-order tridiagonal finite difference method for general non-linear two-point boundary value problems with mixed boundary conditions, IMA J. Appl. Math., 1978, 21(1), 83-93. doi: 10.1093/imamat/21.1.83 CrossRef Google Scholar
[4]	M. M. Chawla, A sixth order tri-diagonal finite difference method for general non-linear two point boundary value problems, IMA J. Appl. Math, 1979, 24(1), 35-42. doi: 10.1093/imamat/24.1.35 CrossRef Google Scholar
[5]	M. M. Chawla, A fourth-order finite difference method based on uniform mesh for singular two-point boundary value problem, J. Comput. Appl. Math., 1987, 17(3), 359-364. doi: 10.1016/0377-0427(87)90112-9 CrossRef Google Scholar
[6]	J. S.R. Chisholm and A. K. Common, A class of second-order differential equations and related first-order systems, J. Phys. A: Math. Gen., 1987, 20(16), 5459-5472. doi: 10.1088/0305-4470/20/16/020 CrossRef Google Scholar
[7]	M. Dehgan, A. Mohebbi, and Z. Asgari, Fourth-order compact solution of the non-linear Klein -Gordon equation, Numer. Algor., 2009, 52(4), 523-540. doi: 10.1007/s11075-009-9296-x CrossRef Google Scholar
[8]	M. Dehghan and M. Tatari, Finding approximate solutions for a class of thirdorder non-linear boundary value problems via the decomposition method of a domain, Int. J. Comput. Math, 2010, 87(6), 1256-1263. doi: 10.1080/00207160802270853 CrossRef Google Scholar
[9]	A. R. Elcrat, On the radial flow of a viscous fluid between porous disks, Arch. Ration. Mech. Anal, 1976, 61(1), 91-96. doi: 10.1007/BF00251865 CrossRef Google Scholar
[10]	D. J. Evans and R. K. Mohanty, Alternating group explicit method for the numerical solution of non-linear singular two point boundary value problems using a fourth order finite difference method, International Journal of Computer Mathematics, 2002, 79(10), 1121-1133. doi: 10.1080/00207160212704 CrossRef Google Scholar
[11]	F. Ghomanjani and S. Shateyi, Alternative methods for solving nonlinear twopoint boundary value problems, Open Physics, 2018, 16(1), 371-374. doi: 10.1515/phys-2018-0050 CrossRef Google Scholar
[12]	V. V. Gobulev, Lectures on Analytical Theory of Differential Equations. Moscow, Gostekhizdat, 1950. Google Scholar
[13]	K. Guckenheimer, J. Hoffman and W. Weckesser, Numerical computation of canards. Internat. J. Bifur. Chaos Appl. Sci. Engrg., 2000, 10(12), 2669-2687. doi: 10.1142/S0218127400001742 CrossRef Google Scholar
[14]	L. A. Hageman and D. M. Young, Applied Iterative Methods, Dover Publications, New York, 2004. Google Scholar
[15]	M. K. Jain, S. R. K. Iyengar, and G. S. Subramanyam, Variable mesh methods for the numerical-solution of 2-point singular perturbation problems, Comput. Meth. Appl. Mech. Eng., 1984, 42(3), 273-286. doi: 10.1016/0045-7825(84)90009-4 CrossRef Google Scholar
[16]	H. B. Keller, Numerical Methods for two points boundary value problems, Waltham Mass: Blaisdell Pub. Co, New York, 1968. Google Scholar
[17]	R. K. Mohanty, A family of variable mesh methods for the estimates of (du/dr) and the solution of non-linear two point boundary value problems with singularity, J. Comput. and Appl. Math., 2005, 182(1), 173-187. doi: 10.1016/j.cam.2004.11.045 CrossRef Google Scholar
[18]	R. K. Mohanty and Noopur Khosla, A third order accurate variable mesh TAGE iterative method for the numerical solution of two point non-linear singular boundary value problems, Int. J. Comp. Math., 2005, 82(10), 1261-1273. Google Scholar
[19]	R. K. Mohanty, A class of non-uniform mesh three point arithmetic average discretization for y'' = f(x, y, y')and the estimates of y', J. Appl. Math. Comput., 2006, 183(1), 477-485. doi: 10.1016/j.amc.2006.05.071 CrossRef Google Scholar
[20]	R. K. Mohanty and Noopur Khosla, Application of TAGE iterative algorithms to an efficient third order arithmetic average variable mesh discretization for two point non-linear boundary value problems, Appl. Math. Comput., 2006, 172(1), 148-162. Google Scholar
[21]	R. K. Mohanty, N. Jha, and V. Chauhan, Arithmetic average geometric mesh discretizations for fourth and sixth order nonlinear two point boundary value problems, Neural Parallel Sci. Comput., 2013, 21(3-4), 393-410. Google Scholar
[22]	R.K. Mohanty, G. Manchanda and A. Khan, Operator compact exponential approximation for the solution of system of 2D second order quasi-linear elliptic partial differential equations, 2019. DOI: 10.1186/s13662-019-1968-9. Google Scholar
[23]	R.K. Mohanty, G. Manchanda and A. Khan, Compact half step approximation in exponential form for 2D second order quasi-linear elliptic partial differential equations, J. Differ. Eqs. Applics., 2019, 25, 716-749. doi: 10.1080/10236198.2019.1624737 CrossRef Google Scholar
[24]	R. K. Mohanty, G. Manchanda, A. Khan and G. Khurana A new high accuracy method in exponential form based on off-step discretization for non-linear two point boundary value problems, J. Differ. Eqs. Appl., 2020, 26(2), 171-202. doi: 10.1080/10236198.2019.1710140 CrossRef Google Scholar
[25]	D. O. Regan, Solvability of some fourth and higher order singular boundary value problems, J. Math. Anal. Appl., 1990, 161, 78-116. Google Scholar
[26]	P. Roul and D. Biswal, A new numerical approach for solving a class of singular two-point boundary value problems, Numer. Algor., 2017, 75, 531-552. doi: 10.1007/s11075-016-0210-z CrossRef Google Scholar
[27]	S. S. Siddiqi and E. H. Twizell, Spline solutions of linear sixth order boundary value problems, Int. J. Comput. Math., 1996, 60(3-4), 295-304. doi: 10.1080/00207169608804493 CrossRef Google Scholar
[28]	S. Selim, G. Elver and M. San Numerical behaviour of singular two point BVPs in a comparative way, Int. J. Optimiz. Contrl.: Th. Applics., 2017, 7(3), 288-292. Google Scholar
[29]	A. I. Selverston and P. F. Rowat, Modeling the gastric mill central pattern generator of the lobster with a relaxation-oscillator network, J. Neurophysiol., 1993, 70(3), 1030-1053. doi: 10.1152/jn.1993.70.3.1030 CrossRef Google Scholar
[30]	H. Smith, An Introduction to Delay Differential Equations with Applications to the Life Sciences, Springer, New York, 2011. Google Scholar
[31]	E. H. Twizell and S. I. A. Tirmizi, Multiderivative methods for non-linear second-order boundary value problems, J. Comput. Appl. Math., 1987, 17, 299-307. doi: 10.1016/0377-0427(87)90107-5 CrossRef Google Scholar
[32]	R. A. Usmani, Finite Difference Methods for a certain two point boundary value problem, Indian J. Pure Appl. Math., 1983, 14(3), 398-411. Google Scholar
[33]	R. A. Usmani, The use of quartic splines in the numerical solution of a fourth -order boundary value problem, J. Comput. Appl. Math., 1992, 44(2), 187-200. doi: 10.1016/0377-0427(92)90010-U CrossRef Google Scholar
[34]	R. S. Varga, Matrix Iterative Analysis, Springer, Berlin, Germany, 2000. Google Scholar
[35]	M. Xu, J. Niu, Y. Lin and Q. Xue.Numerical solution of non linear singular boundary value problems, J. Comput. Appl. Math., 2018, 331, 42-51. doi: 10.1016/j.cam.2017.09.040 CrossRef Google Scholar
[36]	H. Zhu, J. Niu, R. Zhang and Y. Lin A new approach for solving nonlinear singular boundary value problems, Math. Model. Anal., 2018, 23(1), 33-43. Google Scholar