MODULATION INSTABILITY AND OPTICAL SOLITONS OF RADHAKRISHNAN-KUNDU-LAKSHMANAN MODEL

Nauman Raza; Ahmad Javid

doi:10.11948/20190203

2020 Volume 10 Issue 4

Article Contents

Article navigation > Journal of Applied Analysis & Computation > 2020 > 10(4): 1375-1395

Next Article Previous Article

Nauman Raza, Ahmad Javid. MODULATION INSTABILITY AND OPTICAL SOLITONS OF RADHAKRISHNAN-KUNDU-LAKSHMANAN MODEL[J]. Journal of Applied Analysis & Computation, 2020, 10(4): 1375-1395. doi: 10.11948/20190203

Citation:

Nauman Raza, Ahmad Javid. MODULATION INSTABILITY AND OPTICAL SOLITONS OF RADHAKRISHNAN-KUNDU-LAKSHMANAN MODEL[J]. Journal of Applied Analysis & Computation, 2020, 10(4): 1375-1395. doi: 10.11948/20190203

MODULATION INSTABILITY AND OPTICAL SOLITONS OF RADHAKRISHNAN-KUNDU-LAKSHMANAN MODEL

Nauman Raza¹,
Ahmad Javid^1, ,

1.
Department of Mathematics, University of the Punjab, Lahore, Pakistan

Corresponding author: ahmadjavid001@gmail.com(A. Javid)

Abstract

Abstract

This paper studies the solitons of Radhakrishnan-Kundu-Lakshma- nan (RKL) model with power law nonlinearity. The modified simple equation method and $ \exp(-\varphi({q}) $) method are presented as integration mechanisms. Dark, bright, singular and periodic soliton solutions are extracted as well as the constraint conditions for their existence. A prized discussion on the stability of these soliton profiles on the basis of index of the power law nonlinearity is also carried out with the help of physical description of solutions. The integration techniques have been proved to be extremely efficient and robust to find new optical solitary wave solutions for various nonlinear evolution equations describing optical pulse propagation. Moreover, using linear stability analysis, modulation instability of the RKL model is studied. Different effects contributing to the modulation instability spectrum gain are analyzed.
- Optical solitons /
- constraints /
- modified simple equation method /
- ($ \exp(-\varphi({q}) $) method /
- modulation
MSC: 78A60, 35Q51, 35Q55

FullText(HTML)

References

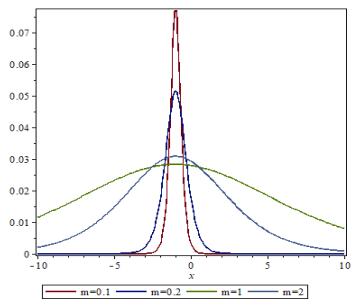
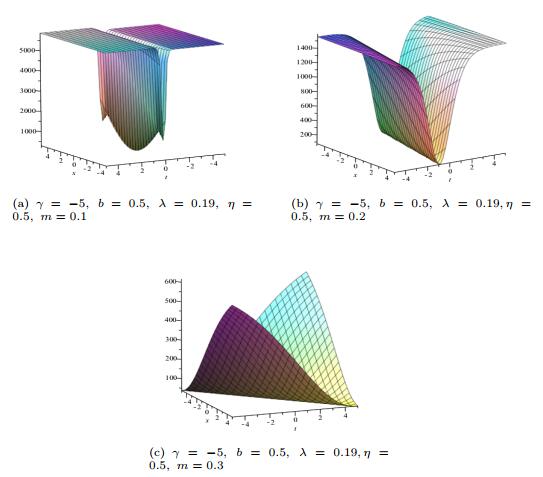
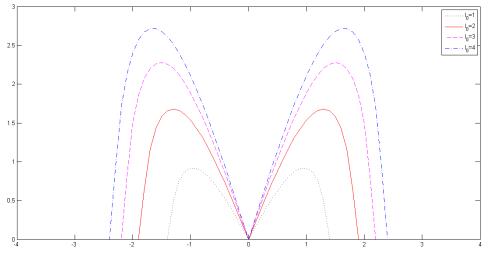
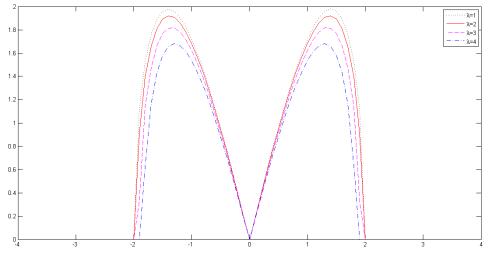
[1]	A. H. Arnous, M. Mirzazadeh, Q. Zhou, S. P. Moshokoa, A. Biswas and M. Belic, Soliton solutions to resonant nonlinear schrodingers equation with time-dependent coefficients by modified simple equation method, Optik., 2016, 127, 11450–11459. doi: 10.1016/j.ijleo.2016.09.055 CrossRef Google Scholar
[2]	G. P. Agrawal, Nonlinear Fiber Optics (5th Edition), Academic Press, New York 2013. Google Scholar
[3]	A. Biswas and S. Konar, Introduction to Non-Kerr Law Optical Solitons, CRC Press, Boca Raton, 2006. Google Scholar
[4]	A. Biswas, 1-Soliton solution of the generalized Radhakrishnan, Kundu, Lakshmanan equation, Phys. Lett. A., 2009, 373(30), 2546–2548. doi: 10.1016/j.physleta.2009.05.010 CrossRef Google Scholar
[5]	M. Ekici, A. Sonmezoglu, Q. Zhou, A. Biswas, M. Z. Ullah, M. Asma, S. P. Moshokoa and M. Belic, Optical solitons in DWDM system by extended trial equation method, Optik., 2017, 141, 157–167. doi: 10.1016/j.ijleo.2017.05.057 CrossRef Google Scholar
[6]	M. Ekici, A. Sonmezoglu, Q. Zhou, S. P. Moshokoa, M. Z. Ullah, A. Biswas and M. Belic, Solitons in magneto-optic waveguides by extended trial function scheme, Superlattices Microstruct., 2017, 107, 197–218. doi: 10.1016/j.spmi.2017.04.021 CrossRef Google Scholar
[7]	M. Ekici, M. Mirzazadeh, A. Sonmezoglu, M. Z. Ullah, Q. Zhou, S. P. Moshokoa, A. Biswas and M. Belic, Nematicons in liquid crystals by extended trial equation method, J. Nonlinear Opt. Phys. Mater., 2017, 26, 1750005. doi: 10.1142/S0218863517500059 CrossRef Google Scholar
[8]	M. Ekici, M. Mirzazadeh, A. Sonmezoglu, M. Z. Ullah, Q. Zhou, H. Triki, S. P. Moshokoa and A. Biswas, Optical solitons with anti-cubic nonlinearity by extended trial equation method, Optik., 2017, 136, 368–373. doi: 10.1016/j.ijleo.2017.02.004 CrossRef Google Scholar
[9]	D. D. Ganji and M. Abdollahzadeh, Exact traveling solutions for the Lax's seventh-order KdV equation by sech method and rational exp-function method, Appl. Math. Comput., 2008, 206(1), 438–444. Google Scholar
[10]	X. Geng and Y. Lv, Darboux transformation for an integrable generalization of the nonlinear Schrdinger equation, Nonlinear Dyn., 2012, 69, 1621–1630. doi: 10.1007/s11071-012-0373-7 CrossRef Google Scholar
[11]	D. D. Ganji and M. Abdollahzadeh, Exact traveling solutions for the Lax's seventh-order KdV equation by sech method and rational exp-function method, Appl. Math. Comput., 2008, 206(1), 438–444. Google Scholar
[12]	D. D. Ganji, A. Asgari and Z.Z. Ganji, Exp-function based solution of nonlinear Radhakrishnan, Kundu and Laskshmanan (RKL) equation, Acta Appl. Math., 2008, 104(2), 201–209. Google Scholar
[13]	A. Javid and N. Raza, Singular and dark optical solitons to the well posed Lakshmanan, Porsezian, Daniel model, Optik., 2018, 171, 120–129. doi: 10.1016/j.ijleo.2018.06.021 CrossRef Google Scholar
[14]	R. Kohl, A. Biswas, D. Milovic and E. Zerrad, Optical soliton perturbation in a non-Kerr law media, Opt. Laser Technol., 2008, 40(4), 647–662. doi: 10.1016/j.optlastec.2007.10.002 CrossRef Google Scholar
[15]	L. Q. Kong and C. Q. Dai, Some discussions about variable separation of nonlinear models using Riccati equation expansion method, Nonlinear Dyn., 2015, 81(3), 1553–1561. Google Scholar
[16]	R. Kohl, D. Milovic, E. Zerrad and A. Biswas, Optical solitons by He's variational principle in a non-Kerr law media, J. Infrared Millim. Terahertz Waves., 2009, 30(5), 526–537. doi: 10.1007/s10762-009-9467-9 CrossRef Google Scholar
[17]	S. Kumar, K. Singh and R. K. Gupta, Coupled Higgs field equation and Hamiltonian amplitude equation: Lie classical approach and G'/G-expansion method, Pramana, 2012, 79, 41–60. doi: 10.1007/s12043-012-0284-7 CrossRef Google Scholar
[18]	R. Kohl, A. Biswas, D. Milovic and E. Zerrad, Optical soliton perturbation in a non-Kerr law media, Opt. Laser Technol., 2008, 40(4), 647–662. doi: 10.1016/j.optlastec.2007.10.002 CrossRef Google Scholar
[19]	R. Kohl, D. Milovic, E. Zerrad and A. Biswas, Optical solitons by Hes variational principle in a non-Kerr law media, J. Infrared Millim. Terahertz Waves., 2009, 30(5), 526–537. doi: 10.1007/s10762-009-9467-9 CrossRef Google Scholar
[20]	C. S. Liu, Representations and classification of traveling wave solutions to sinh-Gordon equation, Commun. Theor. Phys., 2008, 49(1), 153–158. doi: 10.1088/0253-6102/49/1/33 CrossRef Google Scholar
[21]	C. S. Liu, Applications of complete discrimination system for polynomial for classifications of traveling wave solutions to nonlinear differential equations, Comput. Phys. Commun., 2010, 181(2), 317–324. Google Scholar
[22]	M. Mirzazadeh, M. Eslami, E. Zerrad, M. F. Mahmood, A. Biswas and M. Belic, Optical solitons in nonlinear directional couplers by sine-cosine function method and Bernoulli's equation approach, Nonlinear Dyn., 2015, 81(4), 1933–1949. Google Scholar
[23]	M. Mirzazadeh, M. Eslami, E. Zerrad, M. F. Mahommd, A. Biswas and M. Belic, Optical solitons in nonlinear directional couplers by sine-cosine function method and Bernoullis equation approach, Nonlinear Dyn., 2015, 81(4), 1933–1949. doi: 10.1007/s11071-015-2117-y CrossRef Google Scholar
[24]	N. Raza and A. Javid, Optical dark and singular solitons to the Biswas-Milovic equation in nonlinear optics with spatio-temporal dispersion, Optik., 2018, 158, 1049–1057. doi: 10.1016/j.ijleo.2017.12.186 CrossRef Google Scholar
[25]	V. N. Serkin, A. Hasegawa and T. L. Belyaeva, Nonautonomous solitons in external potentials, Phys. Rev. Lett., 2007, 98, 074102. doi: 10.1103/PhysRevLett.98.074102 CrossRef Google Scholar
[26]	B. Sturdevant, D. A. Lott and A. Biswas, Topological 1-soliton solution of generalized Radhakrishnan Kundu Lakshmanan equation with nonlinear dispersion, Mod. Phys. Lett. B, 2010, 24, 1825–1831. doi: 10.1142/S0217984910024109 CrossRef Google Scholar
[27]	A. W. Snyder and D. J. Mitchell, Spatial solitons of the power law nonlinearity, Optics Lett., 1993, 18(2), 101–103. doi: 10.1364/OL.18.000101 CrossRef Google Scholar
[28]	S. N. Sarkar, Variation analysis of spatial solitons of power law nonlinearity, Fib. Int. Optic., 2001, 20(2), 191–196. Google Scholar
[29]	V. A. Vysloukh and N. A. Sukhotskova, Influence of third-order dispersion on the generation of a train of picosecond pulses in fiber waveguides due to self-modulation instability, Sov. J. Quantum Electron., 1987, 17(11), 1509–1511. doi: 10.1070/QE1987v017n11ABEH010977 CrossRef Google Scholar
[30]	Y. Y. Wang and C. Q. Dai, Caution with respect to `new' variable separation solutions and their corresponding localized structures, Appl. Math. Model., 2016, 40(5–6), 3475–3482. doi: 10.1016/j.apm.2015.09.006 CrossRef Google Scholar
[31]	G. Q. Xu, Extended auxiliary equation method and its applications to three generalized NLS equations, Abstr. Appl. Anal., 2014, 2014, 1–7. Google Scholar
[32]	Y. Xiang, S. Wen, X. Dai, Z. Tang, W. Su and D. Fan, Modulation instability induced by nonlinear dispersion in nonlinear metamaterials, Opt. Lett., 2007, 24(12), 3058–3063. Google Scholar
[33]	J. L. Zhang and M. L. Wang, Various exact solutions for two special type RKL models, Chaos Solitons Fractals, 2008, 37(1), 215–226. Google Scholar