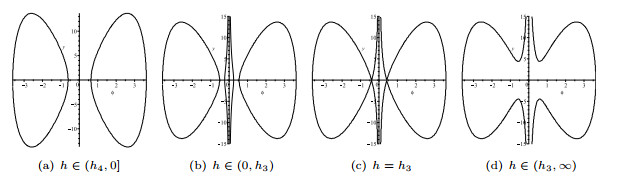
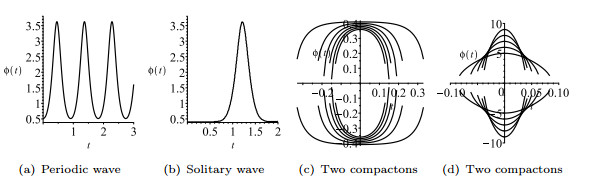
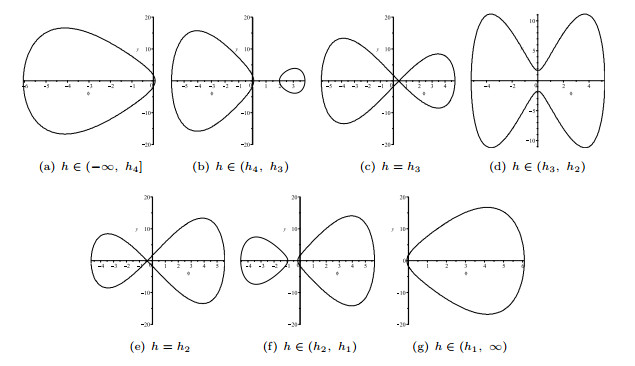|
[1]
|
P. F. Byrd and M. D. Fridman, Handbook of Elliptic Integrals for Engineers and Sciensists, Springer, Berlin, 1971.
Google Scholar
|
|
[2]
|
D. Dias and P. Milewski, On the fully-nonlinear shallow-water generalized serre equations, Physics Letters A, 2010, 374(8), 1049-1053. doi: 10.1016/j.physleta.2009.12.043
CrossRef Google Scholar
|
|
[3]
|
A. E. Green and P. M. Naghdi, A derivation of equations for wave propagation in water of variable depth, Journal of Fluid Mechanics, 1976, 78(2), 237-246. doi: 10.1017/S0022112076002425
CrossRef Google Scholar
|
|
[4]
|
F. Kogelbauer and M. B. Rubin, A class of exact nonlinear traveling wave solutions for shallow water with a non-stationary bottom surface, European Journal of Mechanics / B Fluids, 2019, 76, 26-31. doi: 10.1016/j.euromechflu.2018.12.005
CrossRef Google Scholar
|
|
[5]
|
J. Li, Geometric properties and exact travelling wave solutions for the generalized Burger-Fisher equation and the Sharma-Tasso-Olver equation, Journal of Nonlinear Modeling and Analysis, 2019, 1, 1-10.
Google Scholar
|
|
[6]
|
J. Li, Singular Nonlinear Traveling Wave Equations: Bifurcations and Exact Solutions, Science Press, Beijing, 2013.
Google Scholar
|
|
[7]
|
J. Li and G. Chen, On a class of singular nonlinear traveling wave equations, International Journal of Bifurcation and Chaos, 2007, 17, 4049-4065. doi: 10.1142/S0218127407019858
CrossRef Google Scholar
|
|
[8]
|
J. Li, W. Zhou and G. Chen, Understanding peakons, periodic peakons and compactons via a shallow water wave equation, International Journal of Bifurcation and Chaos, 2016, 26(12), 1650207. doi: 10.1142/S0218127416502072
CrossRef Google Scholar
|
|
[9]
|
P. Naghdi and M. Rubin, On the transition to planing of a boat, Journal of Fluid Mechanics, 1981, 103, 345-374. doi: 10.1017/S0022112081001377
CrossRef Google Scholar
|
|
[10]
|
P. Naghdi and M. Rubin, On inviscid flow in a waterfall, Journal of Fluid Mechanics, 1981, 103, 375-387. doi: 10.1017/S0022112081001389
CrossRef Google Scholar
|
|
[11]
|
F. J. Seabra-Santos, D. P. Renouard and A. M. Temperville, Numerical and experimental study of the transformation of a solitary wave over a shelf or isolated obstacle, Journal of Fluid Mechanics, 1987, 176, 117-134. doi: 10.1017/S0022112087000594
CrossRef Google Scholar
|
|
[12]
|
Y. Zhou, J. Song and T. Han, Solitary waves, periodic peakons, pseudo-peakons and compactons given by three ion-acoustic wave models in electron plasmas, Journal of Applied Analysis and Computation, 2019, 9(2), 810-828.
Google Scholar
|




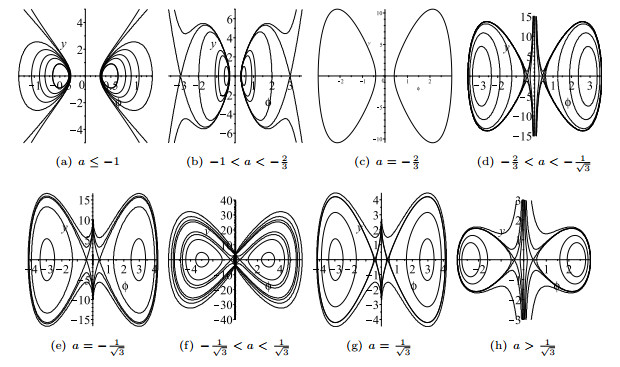

 DownLoad:
DownLoad: