INTERNAL LAYERS FOR A QUASI–LINEAR SINGULARLY PERTURBED DELAY DIFFERENTIAL EQUATION

Tao Feng; Ming-kang Ni

doi:10.11948/20190337

2020 Volume 10 Issue 4

Article Contents

Article navigation > Journal of Applied Analysis & Computation > 2020 > 10(4): 1666-1682

Next Article Previous Article

Tao Feng, Ming-kang Ni. INTERNAL LAYERS FOR A QUASI–LINEAR SINGULARLY PERTURBED DELAY DIFFERENTIAL EQUATION[J]. Journal of Applied Analysis & Computation, 2020, 10(4): 1666-1682. doi: 10.11948/20190337

Citation:

Tao Feng, Ming-kang Ni. INTERNAL LAYERS FOR A QUASI–LINEAR SINGULARLY PERTURBED DELAY DIFFERENTIAL EQUATION[J]. Journal of Applied Analysis & Computation, 2020, 10(4): 1666-1682. doi: 10.11948/20190337

INTERNAL LAYERS FOR A QUASI–LINEAR SINGULARLY PERTURBED DELAY DIFFERENTIAL EQUATION

Tao Feng¹,
Ming-kang Ni^1, ,

1.
School of Mathematical Sciences, East China Normal University, Shanghai, China

Corresponding author: Email address: xiaovikdo@163.com (M. Ni)
Fund Project: The authors were supported by National Natural Science Foundation of China (11871217), supported in part by Science and Technology Commission of Shanghai Municipality (No. 18dz2271000)

Abstract

Abstract

The current paper is mainly concerned with the internal layers for a quasi–linear singularly perturbed differential equation with time delays. By using the method of boundary layer functions and the theory of contrast structures, the existence of a uniformly valid smooth solution is proved, and the asymptotic expansion is constructed. As an application, a concrete example is presented to demonstrate the effectiveness of our result.
- Delay /
- internal layers /
- boundary layer functions /
- contrast structures /
- asymptotic expansion
MSC: 34E20, 34B15

FullText(HTML)

References

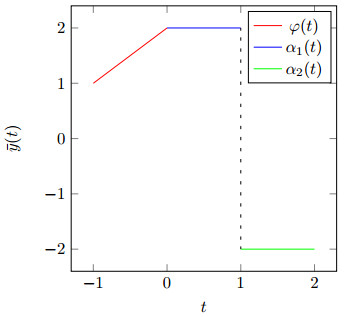
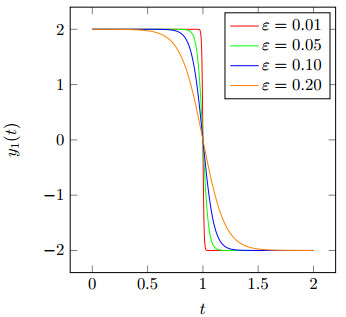
[1]	Z. Bartoszewski and A. Baranowska, Solving boundary value problems for second order singularly perturbed delay differential equations by 'ε'–approximate fixed–point method, Math. Model. Anal., 2015, 20(3), 369–381. Google Scholar
[2]	Y. Cao, Bifurcations in an internet congestion control system with distributed delay, Appl. Math. Comput., 2019, 347, 54–63. Google Scholar
[3]	È. Èl'sgol'c, Qualitative Methods in Mathematical Analysis, American Mathematical Society, Providence, 1964. Google Scholar
[4]	F. Erdogan and Z. Cen, A uniformly almost second order convergent numerical method for singularly perturbed delay differential equations, J. Comput. Appl. Math., 2018, 333, 282–394. Google Scholar
[5]	H. Gao, X. Meng and T. Chen, Stabilization of networked control systems with a new delay characterization, IEEE Trans. Automat. Control, 2008, 53(9), 2142–2148. doi: 10.1109/TAC.2008.930190 CrossRef Google Scholar
[6]	S. Hu and Q. Zhu, Stochastic optimal control and analysis of stability of networked control systems with long delay, Automatica, 2003, 39(11), 1877–1884. doi: 10.1016/S0005-1098(03)00196-1 CrossRef Google Scholar
[7]	H. Kargar, J. Zarei and R. Razavi–Far, Robust fault detection filter design for nonlinear networked control systems with time–varying delays and packet dropout, Circuits, Systems, Signal Process., 2019, 38(1), 63–84. Google Scholar
[8]	Y. Kuang, Delay Differential Equations with Applications in Population Dynamics, Academic Press, Boston, 1993. Google Scholar
[9]	C. Kuehn, Multiple Time Scale Dynamics, Applied Mathematical Sciences, Springer, 2015. Google Scholar
[10]	C. Lange and R. Miura, Singular perturbation analysis of boundary–value problems for differential–difference equations. VI. small shifts with rapid oscillations, SIAM J. Appl. Math., 1994, 54(1), 273–283. Google Scholar
[11]	M. Ni and W. Lin, The asymptotic solutions of delay singularly perturbed differential difference equations (in Chinese), Acta Math. Sci., 2010, 30A(6), 1413–1423. Available from: http://121.43.60.238/sxwlxbA/CN/Y2010/V30/I6/1413. Google Scholar
[12]	M. Ni, On the internal layer for a singularly perturbed system of second–order delay differential equations, Diff. Equ., 2013, 49(8), 941–954. doi: 10.1134/S001226611308003X CrossRef Google Scholar
[13]	N. Olgac, H. Elmali, M. Hosek and M. Renzulli, Active vibration control of distributed systems using delayed resonator with acceleration feedback, J. Dyn. Syst., Measure., Control, 1997, 119(3), 380–389. doi: 10.1115/1.2801269 CrossRef Google Scholar
[14]	V. Rožkov, On the asymptotic behaviour of solutions of equations of neutral type with small time lag (in Russian), Ž. Vyčisl. Mat. i Mat. Fiz., 1964, 4(4, suppl.), 161–175. Google Scholar
[15]	V. Rožkov, Asymptotic formulae in terms of a small time lag for the solution of an equation of neutral type with two retarded arguments (in Russian), Ž. Vyčisl. Mat. i Mat. Fiz., 1964, 4(4, suppl.), 176–182. Google Scholar
[16]	V. Rožkov, Equations of neutral types with variable small retardation (in Russian), Differencial'nye Uravnenija, 1966, 2, 407–416. Google Scholar
[17]	V. Rožkov, An equation of neutral type with a small retardation dependent on the unknown function (in Russian), Izv. Vysš. Učebn. Zaved. Mat., 1968, 1968(6), 90–97. Google Scholar
[18]	J. Ren and W. Ge, Singularly perturbed boundary value problems for semi–linear retarded differential equations with nonlinear boundary conditions, Appl. Math. Mech., 2003, 24(12), 1450–1455. doi: 10.1007/BF02435586 CrossRef Google Scholar
[19]	H. Tian, Asymptotic expansion for the solutions of singularly perturbed delay differential equations, J. Math. Anal. Appl., 2003, 281(2), 678–696. Google Scholar
[20]	A. Vasil'eva, An equation of neutral type with small lag (in Russian), Dokl. Akad. Nauk SSSR, 1962, 145, 495–497. Google Scholar
[21]	A. Vasil'eva and V. Butuzov, Asymptotic methods in the theory of singular perturbations (in Russian), Vyssh. Shkola, Moscow, 1990. Google Scholar
[22]	V. Volterra, Fluctuations in the abundance of a species considered mathematically, Nature, 1926, 118(2972), 558–560. doi: 10.1038/118558a0 CrossRef Google Scholar
[23]	A. Wang and M. Ni, The interior layer for a nonlinear singularly perturbed differential–difference equation, Acta Math. Sci., 2012, 32B(2), 695–709. Google Scholar
[24]	N. Wang and M. Ni, The interior layer phenomena for a class of singularly perturbed delay–differential equations, Acta Math. Sci., 2013, 33B(2), 532–542. Google Scholar