| Citation: |
Jiao-Jiao Dong, Biao Li, Manwai Yuen. GENERAL HIGH-ORDER BREATHER SOLUTIONS, LUMP SOLUTIONS AND MIXED SOLUTIONS IN THE (2+1)-DIMENSIONAL BIDIRECTIONAL SAWADA-KOTERA EQUATION[J]. Journal of Applied Analysis & Computation, 2021, 11(1): 271-286. doi: 10.11948/20190361
|
GENERAL HIGH-ORDER BREATHER SOLUTIONS, LUMP SOLUTIONS AND MIXED SOLUTIONS IN THE (2+1)-DIMENSIONAL BIDIRECTIONAL SAWADA-KOTERA EQUATION
-
Abstract
In this paper, we investigate some interesting solution structures of the (2+1)-dimensional bidirectional Sawada-Kotera (bSK) equation. We obtain general high-order breather solutions by utilizing the Hirota's bilinear method united with the perturbation expansion technique. Taking a long-wave limit of the obtained breather solutions and then making particular parameter constraints, smooth rational solutions are generated, which include high-order lumps and mixed solutions consisting of lumps and stripe. In order to easily explore the dynamical behaviors, some plots are presented to analyse these solutions. -
-
References
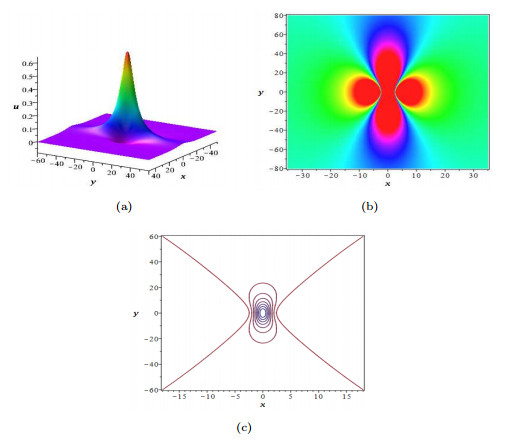
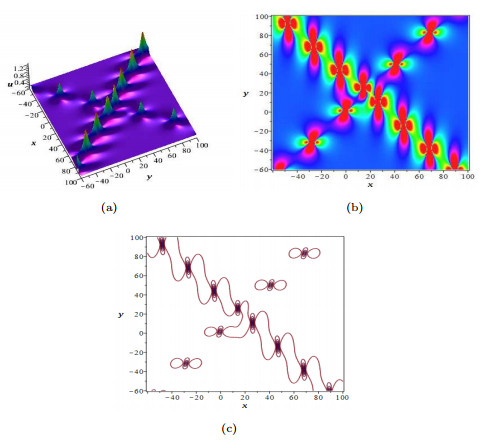
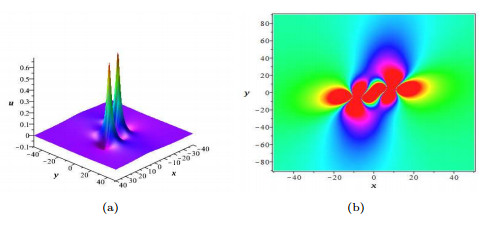
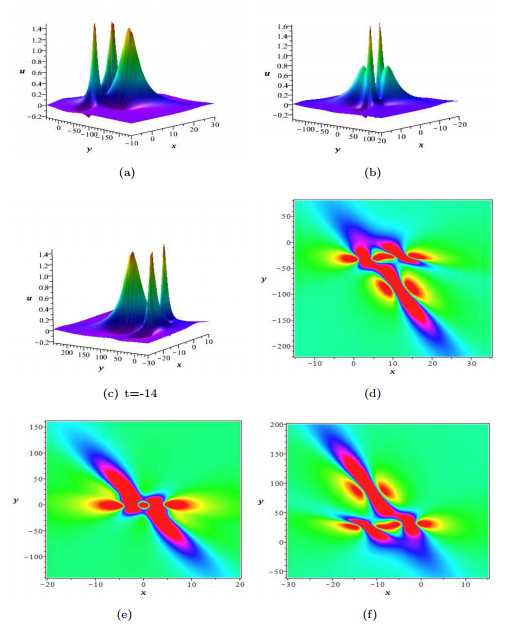
[1] M. J. Ablowitz, M. A. Ablowitz and P. A. Clarkson, Solitons, nonlinear evolution equations and inverse scattering, Cambridge university press, 1991. [2] M. Chen, X. Li, Y. Wang and B. Li, A Pair of Resonance Stripe Solitons and Lump Solutions to a Reduced ($3+1$)-dimensional Nonlinear Evolution Equation, Commun. Theor. Phys., 2017, 67, 595. doi: 10.1088/0253-6102/67/6/595 [3] J. M. Dye and A. Parker, A bidirectional Kaup-Kupershmidt equation and directionally dependent solitons, J. Math. Phys., 2002, 43(10), 4921-4949. doi: 10.1063/1.1503866 [4] E. Date, M. Jimbo, M. Kashiwara and T. Miwa, Transformation groups for soliton equations-Euclidean Lie algebras and reduction of the KP hierarchy, Publ. Res. Inst. Math. Sci., 1982, 18, 1077-1110. doi: 10.2977/prims/1195183297 [5] L. Feng and T. Zhang, Breather wave, rogue wave and solitary wave solutions of a coupled nonlinear Schrödinger equation, Appl. Math. Lett., 2018, 78, 133-140. doi: 10.1016/j.aml.2017.11.011 [6] C. Gu, H. Hu and Z. Zhou, Darboux transformation in soliton theory and its geometric applications, Shanghai. Sci. Tech. Publ. Shanghai, 1999, 1, 999. [7] R. Hirota, The Direct Method in Soliton Theory, Cambridge University Press, New York(NY), 2004. [8] M. Jimbo and T. Miwa, Solitons and infinite dimensional Lie algebras, Publ. Res. Inst. Math. Sci., 1983, 19, 943-1001. doi: 10.2977/prims/1195182017 [9] P. G. Kevrekidis and D. J. Frantzeskakis, Solitons in coupled nonlinear models: a survey of recent developments, Rev. Phys., 2016, 1, 140-153. doi: 10.1016/j.revip.2016.07.002 [10] Y. Liu, B. Li and H. An, General high-order breathers, lumps in the ($2+1$)-dimensional Boussinesq equation, Nonlinear Dyn., 2018, 92, 2061-2076. doi: 10.1007/s11071-018-4181-6 [11] J. Lv and S. D. Bilige, Lump solutions of a ($2+1$)-dimensional bSK equation, Nonlinear Dyn., 2017, 90(3), 2119-2124. doi: 10.1007/s11071-017-3788-3 [12] X. Lai and X. Cai, Adomian decomposition method for approximating the solutions of the bidirectional Sawada-Kotera Equation, Z. Naturf., 2010, 65a, 658-664. [13] V. B. Matveev and M. A. Salle, Darboux transformations and solitons, Springer, Berlin, 1991. [14] Y. Matsuno, Bilinear Transformation Method, Academic, New York(NY), 1984. [15] H. Ma and A. Deng, Lump solution of ($2+1$)-dimensional Boussinesq equation, Commun. Theor. Phys., 2016, 65, 546. doi: 10.1088/0253-6102/65/5/546 [16] W. Ma, Lump solutions to the Kadomtsev-Petviashvili equation, Phys. Lett. A, 2015, 379, 1975-1978. doi: 10.1016/j.physleta.2015.06.061 [17] Y. Ma and X. Geng, Darboux and Bäcklund transformations of the bidirectional Sawada-Kotera equation, Appl. Math. Comput., 2012, 218(12), 6963-6965. [18] W. Ma, X. Yong and H. Q, Diversity of interaction solutions to the ($2+1$)-dimensional Ito equation, Comput. Math. Appl., 2018, 75, 289-295. doi: 10.1016/j.camwa.2017.09.013 [19] W. Peng, S. Tian and T. Zhang, Breather waves, high-order rogue waves and their dynamics in the coupled nonlinear Schrödinger equations with alternate signs of nonlinearities, EPL, 2019. DOI: 10.1209/0295-5075/127/50005. [20] W. Peng and S. Tian, Dynamics of breather waves and higher-order rogue waves in a coupled nonlinear Schrödinger equation, EPL (Europhysics Letters), 2018. DOI: 10.1209/0295-5075/123/50005. [21] H. Sun and A. Chen, Lump and lump-kink solutions of the ($3+1$)-dimensional Jimbo-Miwa and two extended Jimbo-Miwa equations, Appl. Math. Lett., 2017, 68, 55-61. doi: 10.1016/j.aml.2016.12.008 [22] A. M. Wazwaz, The generalized Kaup-Boussinesq equation: multiple soliton solutions, Waves. Random. Complex. Media., 2015, 25, 473-481. doi: 10.1080/17455030.2015.1016474 [23] H. Wang, Lump and interaction solutions to the ($2+1$)-dimensional Burgers equation, Appl. Math. Lett., 2018, 85, 27-34. doi: 10.1016/j.aml.2018.05.010 [24] Y. Wang, M. Chen, X. Li and B. Li, Some interaction solutions of a reduced generalised ($3+1$)-dimensional shallow water wave equation for lump solutions and a pair of resonance solitons, Z. Naturf. A, 2017, 72, 419-424. doi: 10.1515/zna-2017-0057 [25] X. Yan, S. Tian, M. Dong, and T. Zhang, Rogue Waves and Their Dynamics on Bright-Dark Soliton Background of the Coupled Higher Order Nonlinear Schrödinger Equation, J. Phys. Soc. Japan, 2019. DOI: 10.7566/JPSJ.88.074004. [26] J. Yu and Y. Sun, Study of lump solutions to dimensionally reduced generalized KP equations, Nonlinear Dyn., 2017, 87, 2755-2763. doi: 10.1007/s11071-016-3225-z [27] J. Yang and W. Ma, Lump solutions to the BKP equation by symbolic computation, Int. J. Mod. Phys. B, 2016, 30, 1640028. doi: 10.1142/S0217979216400282 [28] J. Yang, W. Ma and Z. Qin, Lump and lump-soliton solutions to the ($2+1$)-dimensional Ito equation, Anal. Math. Phys., 2018, 8, 427-436. doi: 10.1007/s13324-017-0181-9 [29] H. Zhang and W. Ma, Lump solutions to the ($2+1$)-dimensional Sawada-Kotera equation, Nonlinear Dyn., 2017, 87, 2305-2310. doi: 10.1007/s11071-016-3190-6 [30] X. Zhang and Y. Chen, Rogue wave and a pair of resonance stripe solitons to a reduced ($3+1$)-dimensional Jimbo-Miwa equation, Commun. Nonlinear. Sci. Numer. Simul., 2017, 52, 24-31. doi: 10.1016/j.cnsns.2017.03.021 -
-
Figures(7)
Export File
Citation
Jiao-Jiao Dong, Biao Li, Manwai Yuen. GENERAL HIGH-ORDER BREATHER SOLUTIONS, LUMP SOLUTIONS AND MIXED SOLUTIONS IN THE (2+1)-DIMENSIONAL BIDIRECTIONAL SAWADA-KOTERA EQUATION[J]. Journal of Applied Analysis & Computation, 2021, 11(1): 271-286. doi: 10.11948/20190361
Format
Content
DownLoad:
-
Figure 1. (Color online) First-order breather solution
$ u $ of Eq. (1.1) with the parameters$ K_{1} = -i, K_{2} = i, W_{1} = 2-\frac{3}{2}i, W_{2} = 2+\frac{3}{2}i $ ; ($ a $ )3-D plot, ($ b $ )density plot, ($ c $ )contour plot -
Figure 2. (Color online) First-order lump solution
$ u $ of equation (1.1) with the parameters$ K_{1} = 1-i, K_{2} = 1+i, W_{1} = 1, W_{2} = 1 $ : ($ a $ ) 3-D plot; ($ b $ ) density plot; ($ c $ ) contour plot -
Figure 3. (Color online) Second-order breather solution
$ u $ of equation (1.1) with the parameters$ K_{1} = \frac{1}{7}+\frac{i}{9}, K_{2} = \frac{1}{7}-\frac{i}{9}, W_{1} = \frac{2}{9}, W_{2} = \frac{2}{9}, K_{3} = -\frac{1}{8}+\frac{i}{4}, K_{4} = -\frac{1}{8}-\frac{i}{9}, W_{3} = \frac{1}{10}-\frac{2}{9}i, W_{4} = \frac{1}{10}+\frac{2}{9}i, \phi_{1} = 0, \phi_{2} = 0, \phi_{3} = 4, \phi_{4} = 4 $ : ($ a $ ) the 3-D plot; ($ b $ ) density plot; ($ c $ ) contour plot -
Figure 4. (Color online) Second-order lump solution
$ u $ of equation (1.1) with the parameters$ K_{1} = 2-\frac{3}{2}i, K_{2} = 2+\frac{3}{2}i, K_{3} = 2-\frac{3}{2}i, K_{4} = 2+\frac{3}{2}i, W_{1} = 2, W_{2} = 2, W_{3} = 3, W_{4} = 3 $ : ($ a $ ) 3-D plot; ($ b $ ) density plot -
Figure 5. (Color online) Time evolution of three-order lump solution
$ u $ of equation (1.1): ($ a $ )($ b $ )($ c $ ): 3-D plot; ($ d $ ):density plot with$ t = -14 $ , ($ e $ ):density plot with$ t = 0 $ , ($ f $ ):density plot with$ t = 14 $ -
Figure 6. (Color online) Profiles of mixed solution between first-order lump and a stripe with the parameters
$ K_{1} = 3+3i, K_{1} = 3-3i, W_{1} = 5, W_{2} = 5 $ at times ($ a $ )$ t = -100 $ ; ($ b $ )$ t = 0 $ ; ($ c $ )$ t = 100 $ ; ($ d $ ) contour plot at$ t = -100 $ ; ($ e $ ) contour plot at$ t = 0 $ ; ($ f $ ) contour plot at$ t = 100 $ -
Figure 7. (Color online) Profiles of mixed solution between second-order lump and a stripe with the parameters
$ K_{1} = \frac{2}{3}, K_{2} = \frac{2}{3}, K_{3} = -\frac{3}{4}+\frac{i}{3}, K_{4} = -\frac{3}{4}-\frac{i}{3}, k_{5} = 1, W_{1} = \frac{4}{3}-i, W_{2} = \frac{4}{3}+i, W_{3} = -\frac{4}{3}-\frac{i}{4}, W_{4} = -\frac{4}{3}+\frac{i}{4}, w_{5} = \frac{1}{2} $ ; ($ d $ ) contour plot at$ t = -15 $ ; ($ e $ ) contour plot at$ t = 0 $ ; ($ f $ ) contour plot at$ t = 15 $