| Citation: | A. M. Elaiw, N. H. AlShamrani. STABILITY OF A DELAYED ADAPTIVE IMMUNITY HIV INFECTION MODEL WITH SILENT INFECTED CELLS AND CELLULAR INFECTION[J]. Journal of Applied Analysis & Computation, 2021, 11(2): 964-1005. doi: 10.11948/20200124 |
STABILITY OF A DELAYED ADAPTIVE IMMUNITY HIV INFECTION MODEL WITH SILENT INFECTED CELLS AND CELLULAR INFECTION
-
Abstract
In this paper we formulate a mathematical model to investigate the within-host HIV dynamics under the effect of both antibody and Cytotoxic T lymphocytes (CTL) immune responses. The model consists of five components: healthy CD$ 4^{+} $T cells, silent infected cells, active infected cells, free HIV particles, CTLs and antibodies. The healthy CD$ 4^{+} $T cells can be infected when they are contacted by (ⅰ) free HIV particles, (ⅱ) active infected cells, and (ⅲ) silent infected cells. The model is an improvement of some existing HIV infection models with both virus-to-cell (VTC) and cell-to-cell (CTC) transmissions by incorporating the incidence between the silent infected cells and healthy CD$ 4^{+} $T cells. The well-posedness of the model is established by showing that the solutions of the model are nonnegative and bounded. We have shown that the model has five equilibria and their existence is governed by five threshold parameters. We prove the global asymptotic stability of all equilibria by utilizing Lyapunov function and LaSalle's invariance principle. We have presented numerical simulations to illustrate the theoretical results. We have studied the effects of CTC transmission and time delays on the dynamical behavior of the system. We have shown that inclusion of time delay can significantly increase the concentration of the uninfected CD4$ ^{+} $ T cells and reduce the concentrations of the infected cells and free HIV particles. While the inclusion of CTC transmission decreases the concentration of the uninfected CD4$ ^{+} $ T cells and increases the concentrations of the infected cells and free HIV particles.
-

-
References
[1] L. Agosto, M. Herring, W. Mothes and A. Henderson, HIV-1-infected CD4+ T cells facilitate latent infection of resting CD4+ T cells through cell-cell contact, Cell, 2018, 24(8), 2088-2100. [2] K. Allali, J. Danane and Y. Kuang, Global analysis for an HIV infection model with CTL immune response and infected cells in eclipse phase, Applied Sciences, 2017, 7(8), 681. [3] N. Bellomo, K. J. Painter, Y. Tao and M. Winkler, Occurrence vs. Absence of taxis-driven instabilities in a May-Nowak model for virus infection, SIAM Journal of Applied Mathematics, 2019, 79(5), 1990-2010. doi: 10.1137/19M1250261 [4] N. Bellomo and Y. Tao, Stabilization in a chemotaxis model for virus infection, Discrete and Continuous Dynamical Systems-Series S, 2020, 13(2), 105-117. doi: 10.3934/dcdss.2020006 [5] B. Buonomo and C. Vargas-De-Leon, Global stability for an HIV-1 infection model including an eclipse stage of infected cells, Journal of Mathematical Analysis and Applications, 2012, 385(2), 709-720. [6] A. G. Cervantes-Perez and E. Avila-Vales, Dynamical analysis of multipathways and multidelays of general virus dynamics model, International Journal of Bifurcation and Chaos, 2019, 29(3), 1950031-30. [7] W. Chen, N. Tuerxun and Z. Teng, The global dynamics in a wild-type and drug-resistant HIV infection model with saturated incidence, Advances in Difference Equations, 2020, 2020(1), 25. doi: 10.1186/s13662-020-2497-2 [8] R. V. Culshaw and S. Ruan, A delay-differential equation model of HIV infection of CD4+ T-cells, Mathematical Biosciences, 2000, 165(1), 27-39. doi: 10.1016/S0025-5564(00)00006-7 [9] P. Dubey, U. S. Dubey and B. Dubey, Modeling the role of acquired immune response and antiretroviral therapy in the dynamics of HIV infection, Mathematics and Computers in Simulation, 2018, 144(C), 120-137. [10] A. M. Elaiw, Global properties of a class of HIV models, Nonlinear Analysis: Real World Applications, 2010, 11(4), 2253-2263. doi: 10.1016/j.nonrwa.2009.07.001 [11] A. M. Elaiw, R. M. Abukwaik and E. O. Alzahrani, Global properties of a cell mediated immunity in HIV infection model with two classes of target cells and distributed delays, International Journal of Biomathematics, 2014, 7(5), Article ID 1450055. [12] A. M. Elaiw and A. D. AlAgha, Analysis of a delayed and diffusive oncolytic M1 virotherapy model with immune response, Nonlinear Analysis: Real World Applications, 2020, 55, 103116. doi: 10.1016/j.nonrwa.2020.103116 [13] A. M. Elaiw and A. D. AlAgha, Global dynamics of reaction-diffusion oncolytic M1 virotherapy with immune response, Applied Mathematics and Computation, 2020, 367, 124758. doi: 10.1016/j.amc.2019.124758 [14] A. M. Elaiw, A. Almatrafi, A. D. Hobiny and K. Hattaf, Global properties of a general latent pathogen dynamics model with delayed pathogenic and cellular infections, Discrete Dynamics in Nature and Society, 2019, 2019, Article ID 9585497. [15] A. M. Elaiw and N. A. Almuallem, Global dynamics of delay-distributed HIV infection models with differential drug efficacy in cocirculating target cells , Mathematical Methods in the Applied Sciences, 2016, 39(1), 4-31. doi: 10.1002/mma.3453 [16] A. M. Elaiw and M. A. Alshaikh, Stability analysis of a general discrete-time pathogen infection model with humoral immunity, Journal of Difference Equations and Applications, 2019, 25(8), 1149-1172. doi: 10.1080/10236198.2019.1662411 [17] A. M. Elaiw and M. A. Alshaikh, Stability of discrete-time HIV dynamics models with three categories of infected CD4$^{+} $ T-cells, Advances in Difference Equations, 2019, 2019, 407. doi: 10.1186/s13662-019-2338-3 [18] A. M. Elaiw and N. H. AlShamrani, Global stability of a delayed adaptive immunity viral infection with two routes of infection and multi-stages of infected cells, Communications in Nonlinear Science and Numerical Simulation, 2020, 86, Article ID 105259. [19] A. M. Elaiw and N. H. AlShamrani, Global stability of humoral immunity virus dynamics models with nonlinear infection rate and removal, Nonlinear Analysis: Real World Applications, 2015, 26, 161-190. doi: 10.1016/j.nonrwa.2015.05.007 [20] A. M. Elaiw and N. H. AlShamrani, Stability of a general adaptive immunity virus dynamics model with multi-stages of infected cells and two routes of infection, Mathematical Methods in the Applied Sciences, 2020, 43(3), 1145-1175. doi: 10.1002/mma.5923 [21] A. M. Elaiw and N. H. AlShamrani, Stability of a general CTL-mediated immunity HIV infection model with silent infected cell-to-cell spread, Advances in Difference Equations, 2020, 2020, 355. doi: 10.1186/s13662-020-02818-3 [22] A. M. Elaiw and N. H. AlShamrani, Stability of a general delay-distributed virus dynamics model with multi-staged infected progression and immune response, Mathematical Methods in the Applied Sciences, 2017, 40(3), 699-719. doi: 10.1002/mma.4002 [23] A. M. Elaiw and N. H. AlShamrani, Stability of an adaptive immunity pathogen dynamics model with latency and multiple delays, Mathematical Methods in the Applied Sciences, 2018, 41(16), 6645-6672. doi: 10.1002/mma.5182 [24] A. M. Elaiw, S. F. Alshehaiween and A. D. Hobiny, Global properties of a delay-distributed HIV dynamics model including impairment of B-cell functions, Mathematics, 2019, 7(9), 837. doi: 10.3390/math7090837 [25] A. M. Elaiw and S. A. Azoz, Global properties of a class of HIV infection models with Beddington-DeAngelis functional response, Mathematical Methods in the Applied Sciences, 2013, 36(4), 383-394. doi: 10.1002/mma.2596 [26] A. M. Elaiw and E. K. Elnahary, Analysis of general humoral immunity HIV dynamics model with HAART and distributed delays, Mathematics, 2019, 7(2), 157. doi: 10.3390/math7020157 [27] A. M. Elaiw, E. K. Elnahary and A. A. Raezah, Effect of cellular reservoirs and delays on the global dynamics of HIV, Advances in Difference Equations, 2018, 2018(1), 85. doi: 10.1186/s13662-018-1523-0 [28] A. M. Elaiw, A. A. Raezah and S. A. Azoz, Stability of delayed HIV dynamics models with two latent reservoirs and immune impairment, Advances in Difference Equations, 2018, 2018(1), 414. doi: 10.1186/s13662-018-1869-3 [29] L. Gibelli, A. Elaiw, M. A. Alghamdi and A. M. Althiabi, Heterogeneous population dynamics of active particles: Progression, mutations, and selection dynamics, Mathematical Models and Methods in Applied Sciences, 2017, 27(4), 617-640. doi: 10.1142/S0218202517500117 [30] T. Guo, Z. Qiu and L. Rong, Analysis of an HIV model with immune responses and cell-to-cell transmission, Bulletin of the Malaysian Mathematical Sciences Society, 2020, 43, 581-607 doi: 10.1007/s40840-018-0699-5 [31] J. K. Hale and S. V. Lunel, Introduction to functional differential equations, Springer-Verlag, New York, 1993. [32] A. D. Hobiny, A. M. Elaiw and A. Almatrafi, Stability of delayed pathogen dynamics models with latency and two routes of infection, Advances in Difference Equations, 2018, 2018, Article Number: 276. [33] G. Huang, X. Liu and Y. Takeuchi, Lyapunov functions and global stability for age-structured HIV infection model, SIAM Journal on Applied Mathematics, 2012, 72(1), 25-38. doi: 10.1137/110826588 [34] G. Huang, Y. Takeuchi and W. Ma, Lyapunov functionals for delay differential equations model of viral infections, SIAM Journal of Applied Mathematics, 2010, 70(7), 2693-2708. doi: 10.1137/090780821 [35] D. Huang, X. Zhang, Y. Guo and H. Wang, Analysis of an HIV infection model with treatments and delayed immune response, Applied Mathematical Modelling, 2016, 40(4), 3081-3089. doi: 10.1016/j.apm.2015.10.003 [36] C. Jolly and Q. Sattentau, Retroviral spread by induction of virological synapses, Traffic, 2004, 5, 643-650. doi: 10.1111/j.1600-0854.2004.00209.x [37] T. Kajiwara and T. Sasaki, A note on the stability analysis of pathogen-immune interaction dynamics, Discrete and Continuous Dynamical Systems-Series B, 2004, 4(3), 615-622. doi: 10.3934/dcdsb.2004.4.615 [38] N. L. Komarova and D. Wodarz, Virus dynamics in the presence of synaptic transmission, Mathematical Biosciences, 2013, 242(2), 161-171. doi: 10.1016/j.mbs.2013.01.003 [39] A. Korobeinikov, Global properties of basic virus dynamics models, Bulletin of Mathematical Biology, 2004, 66(4), 879-883. doi: 10.1016/j.bulm.2004.02.001 [40] Y. Kuang, Delay Differential Equations with Applications in Population Dynamics, San Diego: Academic Press, 1993. [41] D. Li and W. Ma, Asymptotic properties of a HIV-1 infection model with time delay, Journal of Mathematical Analysis and Applications, 2007, 335(1), 683-691. doi: 10.1016/j.jmaa.2007.02.006 [42] J. Lin, R. Xu and X. Tian, Threshold dynamics of an HIV-1 model with both viral and cellular infections, cell-mediated and humoral immune responses, Mathematical Biosciences and Engineering, 2018, 16(1), 292-319. [43] J. Lin, R. Xu and X. Tian, Threshold dynamics of an HIV-1 virus model with both virus-to-cell and cell-to-cell transmissions, intracellular delay, and humoral immunity, Applied Mathematics and Computation, 2017, 315(C), 516-530. [44] A. Murase, T. Sasaki and T. Kajiwara, Stability analysis of pathogen-immune interaction dynamics, Journal of Mathematical Biology, 2005, 51(3), 247-267. doi: 10.1007/s00285-005-0321-y [45] M. A. Nowak and C. R. M. Bangham. Population dynamics of immune responses to persistent viruses, Science, 1996, 272(5258), 74-79. [46] M. A. Nowak and R. M. May, Virus dynamics: Mathematical Principles of Immunology and Virology, Oxford University Press, Oxford, 2000. [47] A. Perelson, A. Neumann, M. Markowitz, J. Leonard and D. Ho, HIV-1 dynamics in vivo: virion clearance rate, infected cell life-span, and viral generation time, Science, 1996, 271(5), 1582-1586. [48] H. Shu, L. Wang and J. Watmough, Global stability of a nonlinear viral infection model with infinitely distributed intracellular delays and CTL imune responses, SIAM Journal of Applied Mathematics, 2013, 73(3), 1280-1302. doi: 10.1137/120896463 [49] A. Sigal, J. T. Kim, A. B. Balazs, E. Dekel, A. Mayo, R. Milo and D. Baltimore, Cell-to-cell spread of HIV permits ongoing replication despite antiretroviral therapy, Nature, 2011, 477(7362), 95-98. doi: 10.1038/nature10347 [50] Y. Su, D. Sun and L. Zhao, Global analysis of a humoral and cellular immunity virus dynamics model with the Beddington-DeAngelis incidence rate, Mathematical Methods in the Applied Sciences, 2015, 38(14), 2984-2993. doi: 10.1002/mma.3274 [51] J. Wang, M. Guo, X. Liu and Z. Zhao, Threshold dynamics of HIV-1 virus model with cell-to-cell transmission, cell-mediated immune responses and distributed delay, Applied Mathematics and Computation, 2016, 291(C), 149-161. [52] J. Wang, J. Pang, T. Kuniya and Y. Enatsu, Global threshold dynamics in a five-dimensional virus model with cell-mediated, humoral immune responses and distributed delays, Applied Mathematics and Computation, 2014, 241(15), 298-316. [53] J. Wang, C. Qin, Y. Chen and X. Wang, Hopf bifurcation in a CTL-inclusive HIV-1 infection model with two time delays, Mathematical Biosciences and Engineering, 2019, 16(4), 2587-2612. doi: 10.3934/mbe.2019130 [54] X. Wang and S. Liu, A class of delayed viral models with saturation infection rate and immune response, Mathematical Methods in the Applied Sciences, 2013, 36(2), 125-142. doi: 10.1002/mma.2576 [55] WHO: Global Health Observatory (GHO) data. HIV/AIDS. http://www.who.int/gho/hiv/en/,2018. [56] D. Wodarz, Hepatitis C virus dynamics and pathology: The role of CTL and antibody responses, Journal of General Virology, 2003, 84(7), 1743-1750. doi: 10.1099/vir.0.19118-0 [57] J. K. Wong, M. Hezareh, H. F. Gunthard, D. V. Havlir, C. C. Ignacio, C. A. Spina and D. D. Richman, Recovery of replication-competent HIV despite prolonged suppression of plasma viremia, Science 1997, 278(5341), 1291-1295. [58] Y. Yan and W. Wang, Global stability of a five-dimensional model with immune responses and delay, Discrete and Continuous Dynamical Systems-Series B, 2012, 17(1), 401-416. doi: 10.3934/dcdsb.2012.17.401 [59] N. Yousfi, K. Hattaf and A. Tridane, Modeling the adaptive immune response in HBV infection, Journal of Mathematical Biology, 2011, 63(5), 933-957. doi: 10.1007/s00285-010-0397-x -
-
-
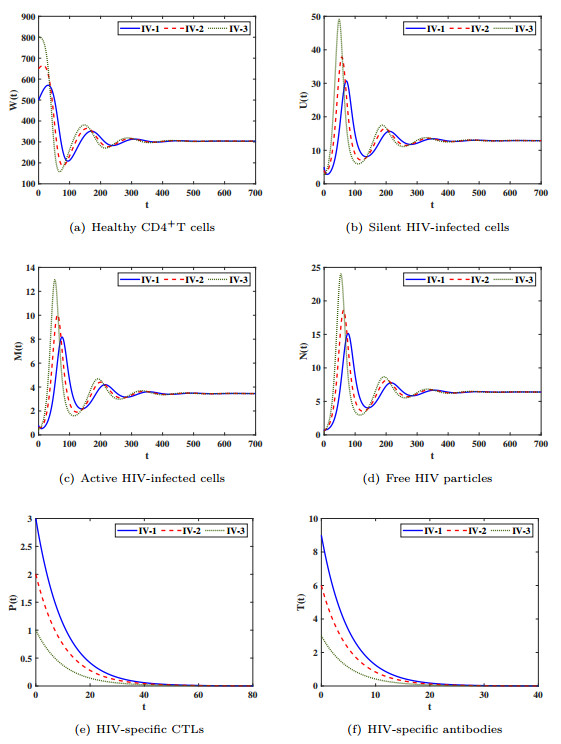
Figure 1. The behavior of solution trajectories of system (6.1) in case of
$ \Re_{0}\leq1 $ . -
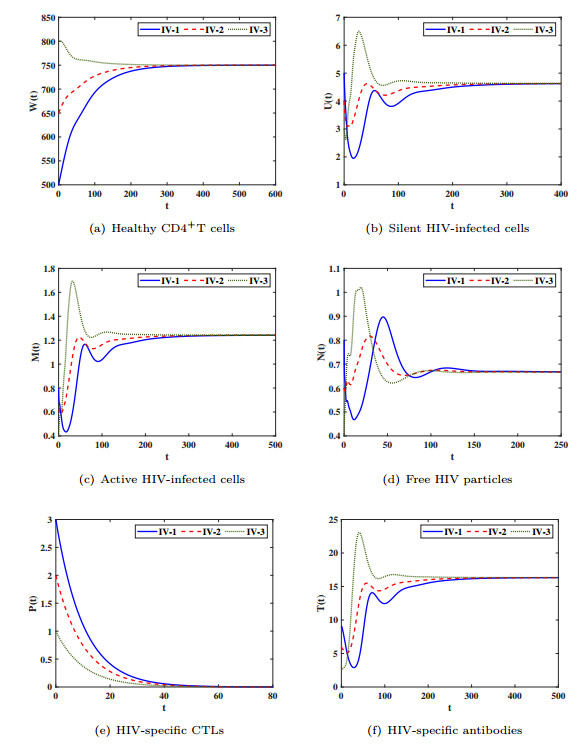
Figure 2. The behavior of solution trajectories of system (6.1) in case of
$ \Re_{0}>1 $ ,$ \Re_{1}\leq1 $ and$ \Re_{2}\leq1 $ . -
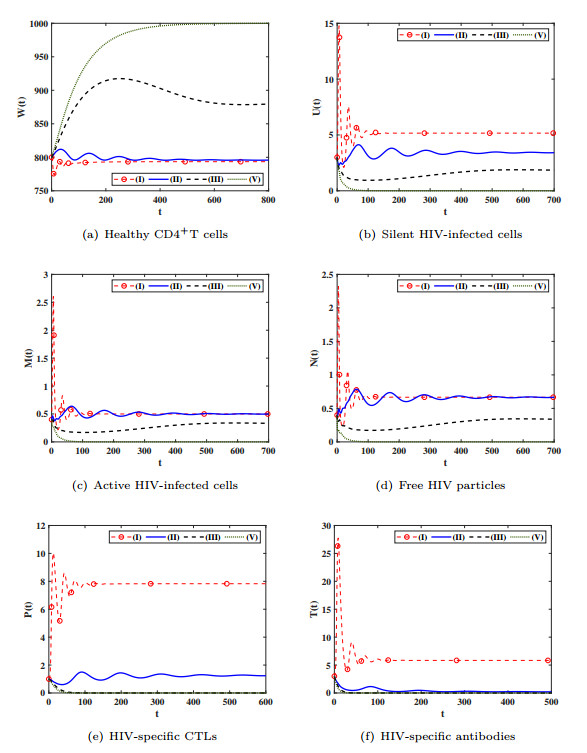
Figure 3. The behavior of solution trajectories of system (6.1) in case of
$ \Re_{1}>1 $ and$ \Re_{4}\leq1 $ . -
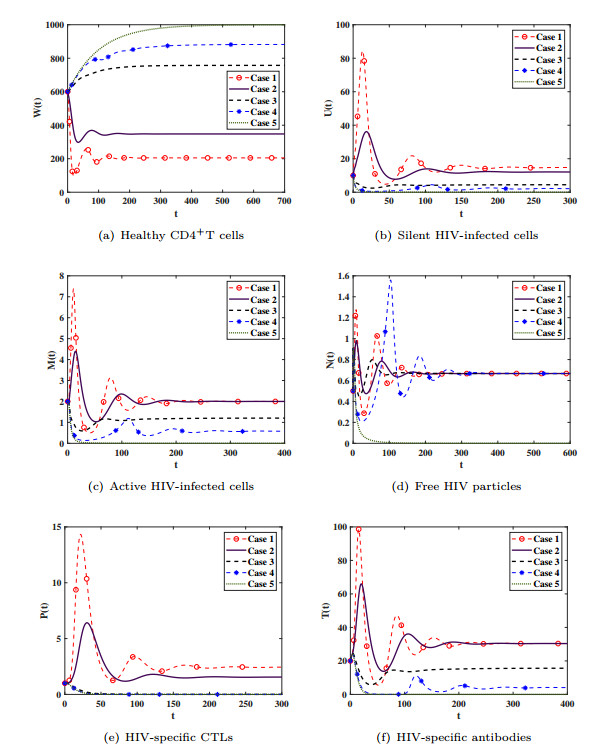
Figure 4. The behavior of solution trajectories of system (6.1) in case of
$ \Re_{2}>1 $ and$ \Re_{3}\leq1 $ . -
Figure 5. The behavior of solution trajectories of system (6.1) in case of
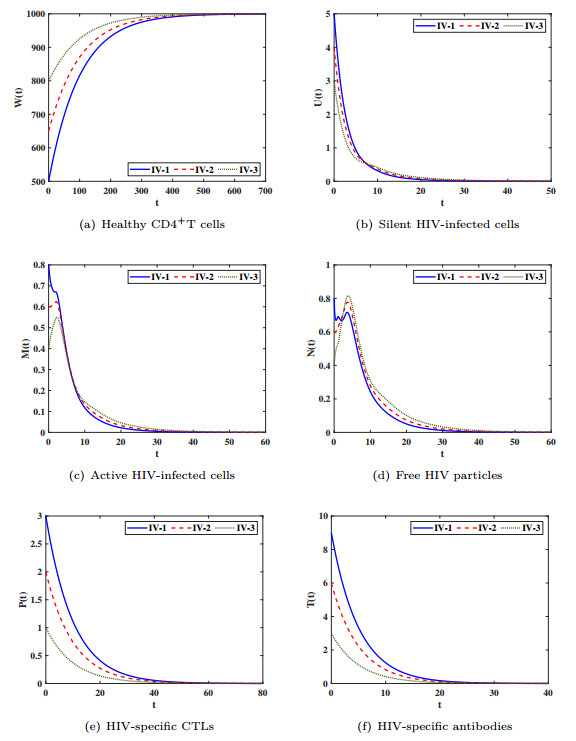
$ \Re_{3}>1 $ and$ \Re_{4}>1 $ . - Figure 6. The influence of time delay parameters on the behavior of solution trajectories of system (6.1).
- Figure 7. The evolution of HIV dynamics (6.1) under different modes of transmissions.




 DownLoad:
DownLoad: