FIXED POINT THEOREMS OVER $\mathfrak{B}$-METRIC-LIKE SPACES AND APPLICATIONS IN ELECTRIC CIRCUIT EQUATIONS

Mohammed M. A. Taleb; Saeed A. A. Al-Salehi; Shah Muhammad; V. C. Borkar

doi:10.11948/20240259

2025 Volume 15 Issue 3

Article Contents

Article navigation > Journal of Applied Analysis & Computation > 2025 > 15(3): 1430-1452

Next Article Previous Article

Mohammed M. A. Taleb, Saeed A. A. Al-Salehi, Shah Muhammad, V. C. Borkar. FIXED POINT THEOREMS OVER $\mathfrak{B}$-METRIC-LIKE SPACES AND APPLICATIONS IN ELECTRIC CIRCUIT EQUATIONS[J]. Journal of Applied Analysis & Computation, 2025, 15(3): 1430-1452. doi: 10.11948/20240259

Citation:

Mohammed M. A. Taleb, Saeed A. A. Al-Salehi, Shah Muhammad, V. C. Borkar. FIXED POINT THEOREMS OVER $\mathfrak{B}$-METRIC-LIKE SPACES AND APPLICATIONS IN ELECTRIC CIRCUIT EQUATIONS[J]. Journal of Applied Analysis & Computation, 2025, 15(3): 1430-1452. doi: 10.11948/20240259

FIXED POINT THEOREMS OVER $\mathfrak{B}$-METRIC-LIKE SPACES AND APPLICATIONS IN ELECTRIC CIRCUIT EQUATIONS

1.
Department of Mathematics, Science College, Swami Ramanand Teerth Marathwada University, Nanded-431606, India
2.
Department of Mathematics, College of Science, King Saud University, P.O. Box 2455, Riyadh 11451, Saudi Arabia
3.
Department of Mathematics, Yeshwant Mahavidyalaya, Swami Ramanand Teerth Marathwada University, Nanded-431606, India

Author Bio: Email: alsalehi.saeed72@gmail.com(S. A. A. Al-Salehi); Email: skabeer@ksu.edu.sa(S. Muhammad); Email: borkarvc@gmail.com(V. C. Borkar)
Corresponding author: Email: mohaayedtaleb@gmail.com(M. M. A. Taleb)
Fund Project: The authors were supported by King Saud University, Riyadh, Saudi Arabia (RSPD2025R733)

Abstract

Abstract

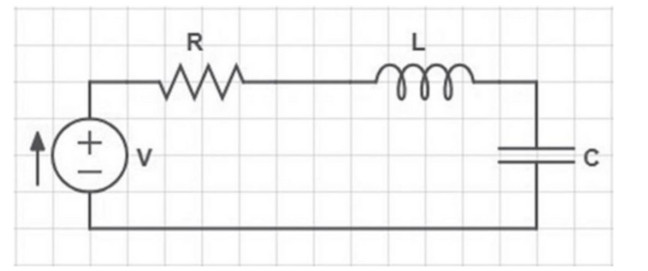
In this article, we introduce the notion of $\alpha$-admissible crooked mapping with respect to $\theta$ with its special cases, which are $\alpha$-admissible crooked mapping with respect to $\theta^*$ and $\alpha^*$-admissible crooked mapping with respect to $\theta$. We present the notion of $(\beta\gamma, \alpha\theta, \psi F)$-rational contraction and establish new fixed point results over $\mathfrak{b}$-metric-like space. The study includes illustrative examples to support our results. Furthermore, we apply our results to prove the existence and uniqueness solution of the electric circuit equation, which is in second-order differential equation form.
MSC: 47H10, 54H25

FullText(HTML)

References

[1]	M. A. Alghamdi, N. Hussain and P. Salimi, Fixed point and coupled fixed point theorems on $b$-metric-like spaces, J. Inequal. Appl., 2013, 2013, 1–25. doi: 10.1186/1029-242X-2013-1 CrossRef $b$-metric-like spaces" target="_blank">Google Scholar
[2]	M. Berzig, S. Chandok and M. S. Khan, Generalized Krasnoselskii fixed point theorem involving auxiliary functions in bimetric spaces and application to two-point boundary value problem, Appl. Math. Comput., 2014, 248, 323–327. Google Scholar
[3]	H. Bouhadjera, H. Z. Alzumi, W. Shammakh, S. M. Ali and M. S. Abdo, Unique common fixed points for occasionally weakly biased maps of type in-metric-like spaces, J. Funct. Spaces, 2023, 2023, 3937224. Google Scholar
[4]	S. S. Chauhan, K. Rana, M. Asim and M. Imdad, Geraghty type contractions in $b$-metric-like spaces, Korean J. Math., 2022, 30(4), 603–614. $b$-metric-like spaces" target="_blank">Google Scholar
[5]	C. Chen, L. Wen, J. Dong and Y. Gu, Fixed point theorems for generalized F-contractions in $b$-metric-like spaces, J. Nonlinear Sci. Appl., 2016, 9, 2161–2174. doi: 10.22436/jnsa.009.05.21 CrossRef $b$-metric-like spaces" target="_blank">Google Scholar
[6]	S. Czerwik, Contraction mappings in $b$-metric spaces, Acta Math. Inform. Univ. Ostrav., 1993, 1(1), 5–11. $b$-metric spaces" target="_blank">Google Scholar
[7]	D. Gopal, M. Abbas and C. Vetro, Some new fixed point theorems in Menger PM-spaces with application to Volterra type integral equation, Appl. Math. Comput., 2014, 232, 955–967. Google Scholar
[8]	T. Hamaizia, Fixed point theorems for generalized $(\psi, \phi, F)$-contraction type mappings in $b$-metric spaces with applications, Open J. Math. Anal., 2021, 5, 35–41. doi: 10.30538/psrp-oma2021.0080 CrossRef $(\psi, \phi, F)$-contraction type mappings in $b$-metric spaces with applications" target="_blank">Google Scholar
[9]	H. A. Hammad and M. De la Sen, Fixed-point results for a generalized almost $(s, q)$-jaggi F-Contraction-Type on $b$-metric-like spaces, Mathematics, 2020, 8(1), 63. doi: 10.3390/math8010063 CrossRef $(s, q)$-jaggi F-Contraction-Type on $b$-metric-like spaces" target="_blank">Google Scholar
[10]	H. Huang, G. Deng and S. Radenovic, Fixed point theorems for C-class functions in $b$-metric spaces and applications, Nonlinear Sci. Appl., 2017, 10, 5853–5868. doi: 10.22436/jnsa.010.11.23 CrossRef $b$-metric spaces and applications" target="_blank">Google Scholar
[11]	H. Huang, K. Zoto, Z. D. Mitrovic and S. Radenovic, Fixed point results for generalized F-contractions in $b$-metric-like spaces, Fractal. Fract., 2022, 6(5), 272. doi: 10.3390/fractalfract6050272 CrossRef $b$-metric-like spaces" target="_blank">Google Scholar
[12]	Z. Liu, X. Li, S. M. Kang and S. Y. Cho, Fixed point theorems for mappings satisfying contractive conditions of integral type and applications, Fixed Point Theory Appl., 2011, 2011, 1–18. doi: 10.1186/1687-1812-2011-1 CrossRef Google Scholar
[13]	P. Saipara, K. Khammahawong and P. Kumam, Fixed‐point theorem for a generalized almost Hardy‐Rogers‐type F contraction on metric‐like spaces, Math. Meth. Appl. Sci., 2019, 42(17), 5898–5919. doi: 10.1002/mma.5793 CrossRef Google Scholar
[14]	P. Salimi, A. Latif and N. Hussain, Modified $\alpha$-$\psi$-contractive mappings with applications, Fixed Point Theory Appl., 2013, 2013(1), 1–19. doi: 10.1186/1687-1812-2013-1 CrossRef $\alpha$-$\psi$-contractive mappings with applications" target="_blank">Google Scholar
[15]	B. Samet, C. Vetro and P. Vetro, Fixed point theorems for $\alpha–\psi$-contractive type mappings, Nonlinear Anal., 2012, 75(4), 2154–2165. doi: 10.1016/j.na.2011.10.014 CrossRef $\alpha-\psi$-contractive type mappings" target="_blank">Google Scholar
[16]	M. Taleb and V. C. Borkar, Some rational contraction and applications of fixed point theorems to F-metric space in differential equations, J. Math. Comput. Sci., 2022, 12, 133. Google Scholar
[17]	M. Taleb and V. C. Borkar, New fixed point results for some rational contraction on $(\phi, \psi)$-metric spaces, Sahand Commun. Math. Anal., 2024, 21(1), 47–66. $(\phi, \psi)$-metric spaces" target="_blank">Google Scholar
[18]	M. Taleb, S. Al-Salehi and V. C. Borkar, New fixed point results over orthogonal F-metric spaces and application in second-order differential equations, J. Nonlinear Model. Anal., 2024, 6(3), 825–840. Google Scholar
[19]	A. Tomar and R. Sharma, Some coincidence and common fixed point theorems concerning F-contraction and applications, J. Int. Math. Virtual Inst., 2018, 8, 181–198. Google Scholar
[20]	K. Zoto, H. Aydi and H. Alsamir, Generalizations of some contractions in $b$-metric-like spaces and applications to boundary value problems, Adv. Differ. Equ., 2021, 2021(1), 1–18. $b$-metric-like spaces and applications to boundary value problems" target="_blank">Google Scholar
[21]	K. Zoto, M. Gardasevic-Filipovic, I. Vardhami, Z. Mitrović and S. Radenovic, General new results on $(\phi, \mathcal{F})$-contractions in $b$-metric-like-spaces, Axioms, 2023, 12(7), 672. $(\phi, \mathcal{F})$-contractions in $b$-metric-like-spaces" target="_blank">Google Scholar
[22]	K. Zoto, S. Radenovic and A. H. Ansari, On some fixed point results for $(s, p, \alpha)$-contractive mappings in $b$-metric-like spaces and applications to integral equations, Open Math., 2018, 16(1), 235–249. $(s, p, \alpha)$-contractive mappings in $b$-metric-like spaces and applications to integral equations" target="_blank">Google Scholar
[23]	K. Zoto, B. E. Rhoades and S. Radenovic, Some generalizations for $(\alpha-\psi, \phi)$-contractions in $b$-metric-like spaces and an application, Fixed Point Theory Appl., 2017, 2017, 1–20. $(\alpha-\psi, \phi)$-contractions in $b$-metric-like spaces and an application" target="_blank">Google Scholar
[24]	K. Zoto, I. Vardhami, D. Bajovic, Z. Mitrovic and S. Radenovic, On some novel fixed point results for generalized F-contractions in $b$-metric-like spaces with application, Comput. Model. Eng. Sci., 2023, 135(1), 673–686. $b$-metric-like spaces with application" target="_blank">Google Scholar