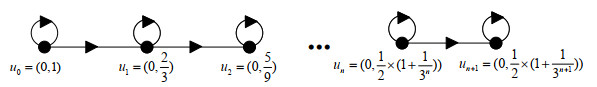| Citation: | Chaobo Li, Yunan Cui, Rizwan Anjum. BEST PROXIMITY POINTS FOR WEAK PROXIMAL ENRICHED G-CONTRACTIONS IN GRAPHICAL CONVEX METRIC SPACES[J]. Journal of Applied Analysis & Computation, 2025, 15(4): 2392-2407. doi: 10.11948/20240484 |
BEST PROXIMITY POINTS FOR WEAK PROXIMAL ENRICHED G-CONTRACTIONS IN GRAPHICAL CONVEX METRIC SPACES
-
Abstract
In this paper, we redefine the concept of a graphical convex metric space involving sets with a graphical structure, and a new class of non-self mappings, called weak proximal enriched $ G- $contractions, is introduced in the aforementioned space. Moreover, we demonstrate the existence of best approximation points for two types of weak proximal enriched $ G- $contractions in graphical convex metric spaces, under suitable control conditions. Additionally, we provide examples to confirm our main results.
-

-
References
[1] P. R. Agarwal, D. O'Regan and D. R. Sahu. Fixed Point Theory for Lipschitzian-Type Mappings with Applications, New York, Springer, 2009, 6. [2] Ya. I. Alber and S. Guerre-Delabriere, Principle of weakly contractive maps in Hilbert spaces, in: New Results in Operator Theory and its Applications, Birkhäuser, Basel, 1997, 98, 7-22. [3] R. Anjum, M. Abbas and H. Işık, Completeness problem via fixed point theory, Complex Anal. Oper. Th., 2023, 17(6), 85. doi: 10.1007/s11785-023-01385-1 [4] S. Banach, Sur les opérations dans les ensembles abstraits et leur application aux équations intégrales, Fund. Math., 1922, 3, 133-181. doi: 10.4064/fm-3-1-133-181 [5] S. S. Basha, Best proximity points: Global optimal approximate solutions, J. Global Optim., 2011, 49, 15-21. doi: 10.1007/s10898-009-9521-0 [6] S. S. Basha, Best proximity point theorems, J. Approx. Theory, 2011, 163, 1772-1781. doi: 10.1016/j.jat.2011.06.012 [7] V. Berinde and M. Pǎcurar, Approximating fixed points of enriched contractions in Banach spaces, J. Fixed Point Theory Appl., 2020, 22, 1-10. doi: 10.1007/s11784-019-0746-3 [8] V. Berinde and M. Pǎcurar, Existence and approximation of fixed points of enriched contractions and enriched φ-Contractions, Symmetry, 2021, 13(3), 498. doi: 10.3390/sym13030498 [9] L. L. Chen, L. Gao and D. Y. Chen, Fixed point theorems of mean nonexpansive set-valued mappings in Banach spaces, J. Fixed Point Theory Appl., 2017, 19, 2129-2143. doi: 10.1007/s11784-017-0401-9 [10] L. L. Chen, Y. Y. Jiang and Y. F. Zhao, Iterative algorithms and fixed point theorems for set-valued G-contractions in graphical convex metric spaces, J. Appl. Anal. Comput., 2024, 14(6), 3558-3580. [11] L. L. Chen, C. B. Li, R. Kaczmarek and Y. F. Zhao, Several fixed point theorems in convex $b-$metric spaces and applications, Mathematics, 2020, 8(2), 242. doi: 10.3390/math8020242 [12] L. L. Chen, N. Yang, Y. F. Zhao and Z. H. Ma, Fixed point theorems for set-valued G-contractions in a graphical convex metric space with applications, J. Fixed Point Theory Appl., 2020, 22, 1-23. doi: 10.1007/s11784-019-0746-3 [13] L. B. Ćirić, A generalization of Banach's contraction principle, Proc. Am. Math. Soc., 1974, 45, 267-273. [14] R. Espinola and W. A. Kirk, Fixed point theorems in R-trees with applications to graph theory, Topology Appl., 2006, 153, 1046-1055. doi: 10.1016/j.topol.2005.03.001 [15] J. Jachymski, The contraction principle for mappings on a metric space with a graph, Proc. Amer. Math. Soc., 2008, 1, 1359-1373. [16] S. H. Khan and M. Abbas, Common fixed point results with applications in convex metric spaces, J. Concr. Appl. Math., 2012, 10, 65-76. [17] P. D. Proinov, Fixed point theorems for generalized contractive mappings in metric spaces, J. Fixed Point Theory Appl., 2020, 22, 21. [18] B. E. Rhoades, Some theorems on weakly contractive maps, Nonlinear Anal., 2001, 47, 2683-2693. doi: 10.1016/S0362-546X(01)00388-1 [19] T. Suzuki, A new type of fixed point theorem in metric spaces, Nonlinear Anal. Theor., 2009, 71, 5313-5317. [20] W. Takahashi, A convexity in metric space and nonexpansive mappings, I. Kodai Math. J., 1970, 22, 142-149. [21] T. Thianwan and D. Yambangwai, Convergence analysis for a new two-step iteration process for G-nonexpansive mappings with directed graphs, J. Fixed Point Theory Appl., 2019, 21(44), 1-16. [22] D. Wardowski, Fixed points of a new type of contractive mappings in complete metric spaces, Fixed Point Theory A., 2012, 2012, 1-6. [23] D. Yambangwai, S. Aunruean and T. Thianwan, A new modified three-step iteration method for G-nonexpansive mappings in Banach spaces with a graph, Numer. Algor., 2019, 20, 1-29. [24] M. Younis, D. Singh and A. A. N. Abdou, A fixed point approach for tuning circuit problem in dislocated b-metric spaces, Math. Meth. Appl. Sci., 2022, 45(4), 2234-2253. [25] M. Younis, D. Singh, L. L. Chen and M. Metwali, A study on the solutions of notable engineering models, Math. Model. Anal., 2022, 27(3), 492-509. [26] M. Younis, D. Singh and A. Goyal, A novel approach of graphical rectangular b-metric spaces with an application to the vibrations of a vertical heavy hanging cable, J. Fixed Point Theory Appl., 2019, 21, 1-33. -
-
-
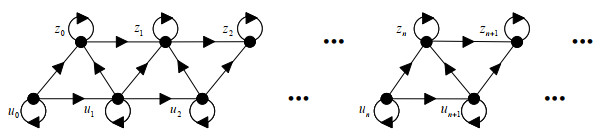
Figure 1.
The
$ T- $ -
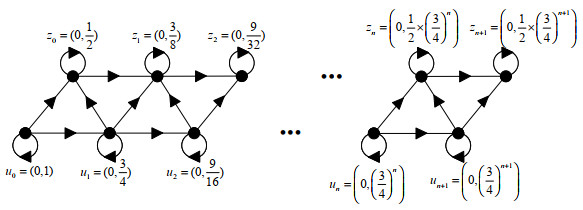
Figure 2.
The graph of
$ \{u_{n}\} $ $ \{z_{n}\} $ -
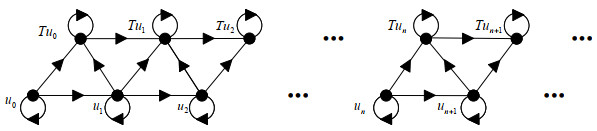
Figure 3.
Graph associated with Example 2.5.
-
Figure 4.
The graph of
$ \{u_{n}\} $ -
Figure 5.
Graph associated with Example 2.6.




 DownLoad:
DownLoad: