| Citation: |
Huanyan Jian, Tingzhu Huang, Xile Zhao, Yongliang Zhao. FAST SECOND-ORDER ACCURATE DIFFERENCE SCHEMES FOR TIME DISTRIBUTED-ORDER AND RIESZ SPACE FRACTIONAL DIFFUSION EQUATIONS[J]. Journal of Applied Analysis & Computation, 2019, 9(4): 1359-1392. doi: 10.11948/2156-907X.20180247
|
FAST SECOND-ORDER ACCURATE DIFFERENCE SCHEMES FOR TIME DISTRIBUTED-ORDER AND RIESZ SPACE FRACTIONAL DIFFUSION EQUATIONS
-
Abstract
The aim of this paper is to develop fast second-order accurate difference schemes for solving one- and two-dimensional time distributed-order and Riesz space fractional diffusion equations. We adopt the same measures for one- and two-dimensional problems as follows: we first transform the time distributed-order fractional diffusion problem into the multi-term time-space fractional diffusion problem with the composite trapezoid formula. Then, we propose a second-order accurate difference scheme based on the interpolation approximation on a special point to solve the resultant problem. Meanwhile, the unconditional stability and convergence of the new difference scheme in L2-norm are proved. Furthermore, we find that the discretizations lead to a series of Toeplitz systems which can be efficiently solved by Krylov subspace methods with suitable circulant preconditioners. Finally, numerical results are presented to show the effectiveness of the proposed difference methods and demonstrate the fast convergence of our preconditioned Krylov subspace methods. -
-
References
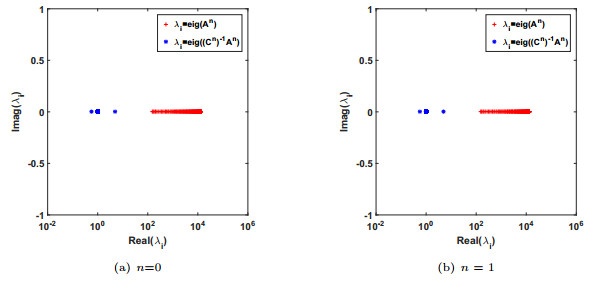
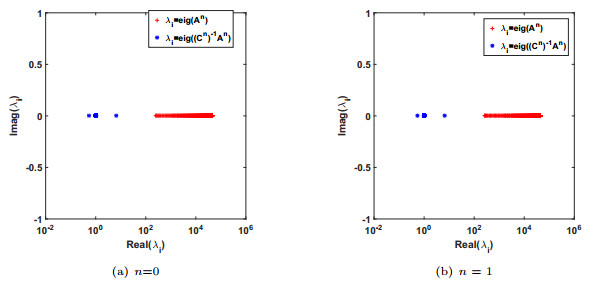
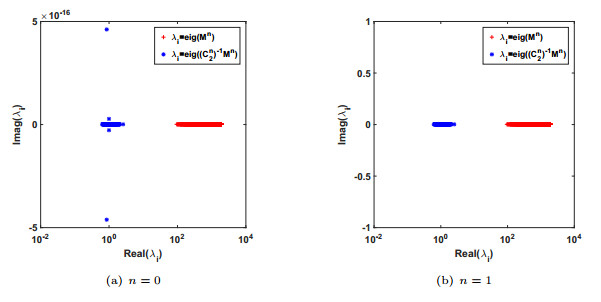
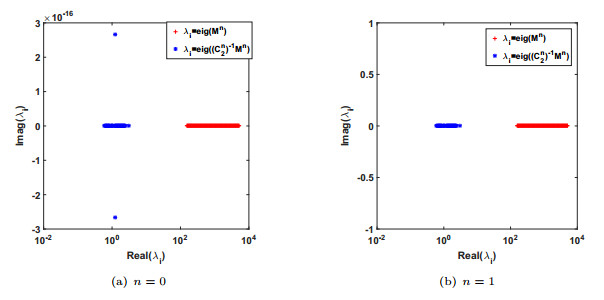
[1] A. A. Alikhanov, A new difference scheme for the time fractional diffusion equation, J. Comput. Phys., 2015, 280, 424-438. doi: 10.1016/j.jcp.2014.09.031 [2] A. H. Bhrawy and M. A. Zaky, A method based on the Jacobi tau approximation for solving multi-term time-space fractional partial differential equations, J. Comput. Phys., 2015, 281, 876-895. doi: 10.1016/j.jcp.2014.10.060 [3] A. H. Bhrawy and M. A. Zaky, Numerical simulation of multi-dimensional distributed-order generalized Schrödinger equations, Nonlinear Dyn., 2017, 89, 1415-1432. doi: 10.1007/s11071-017-3525-y [4] W. Bu, A. Xiao and W. Zeng, Finite difference/finite element methods for distributed-order time fractional diffusion equations, J. Sci. Comput., 2017, 72(3), 422-441. [5] R. Chan and X. Q. Jin, An Introduction to Iterative Toeplitz Solvers, SIAM, PA, 2007. [6] R. Chan and G. Strang, Toeplitz equations by conjugate gradients with circulant preconditioner, SIAM J. Sci. Stat. Comput., 1989, 10(1), 104-119. doi: 10.1137/0910009 [7] A. Chechkin, R. Gorenflo and I. Sokolov, Retarding subdiffusion and accelerating superdiffusion governed by distributed-order fractional diffusion equations, Phys. Rev. E, 2002, 66(4), 046129. doi: 10.1103/PhysRevE.66.046129 [8] L. K. Chou and S. L. Lei, Fast ADI method for high dimensional fractional diffusion equations in conservative form with preconditioned strategy, Comput. Math. Appl., 2017, 73(3), 385-403. doi: 10.1016/j.camwa.2016.11.034 [9] G. H. Gao, A. A. Alikhanov and Z. Z. Sun, The temporal second order difference schemes based on the interpolation approximation for solving the time multiterm and distributed-order fractional sub-diffusion equations, J. Sci. Comput., 2017, 73, 93-121. doi: 10.1007/s10915-017-0407-x [10] G. H. Gao, H. W. Sun and Z. Z. Sun, Some high-order difference schemes for the distributed-order differential equations, J. Comput. Phys., 2015, 298, 337-359. doi: 10.1016/j.jcp.2015.05.047 [11] G. H. Gao and Z. Z. Sun, Two alternating direction implicit difference schemes with the extrapolation method for the two-dimensional distributed-order differential equations, Comput. Math. Appl., 2015, 69(9), 926-948. doi: 10.1016/j.camwa.2015.02.023 [12] X. M. Gu, T. Z. Huang, B. Carpentieri et al., A hybridized iterative algorithm of the BiCORSTAB and GPBiCOR methods for solving non-Hermitian linear systems, Comput. Math. Appl., 2015, 70(12), 3019-3031. doi: 10.1016/j.camwa.2015.10.012 [13] X. M. Gu, T. Z. Huang, C. C. Ji et al., Fast iterative method with a second order implicit difference scheme for time-space fractional convection-diffusion equations, J. Sci. Comput., 2017, 72, 957-985. doi: 10.1007/s10915-017-0388-9 [14] X. M. Gu, T. Z. Huang, H. B. Li et al., On k-step CSCS-based polynomial preconditioners for Toeplitz linear systems with application to fractional diffusion equations, Appl. Math. Lett., 2015, 42, 53-58. doi: 10.1016/j.aml.2014.11.005 [15] X. M. Gu, T. Z. Huang, X. L. Zhao et al., Strang-type preconditioners for solving fractional diffusion equations by boundary value methods, J. Comput. Appl. Math., 2015, 277, 73-86. doi: 10.1016/j.cam.2014.08.011 [16] B. Henry, T. Langlands and S. Wearne, Anomalous diffusion with linear reaction dynamics: from continuous time random walks to fractional reactiondiffusion equations, Phys. Rev. E, 2006, 74(3), 031116. doi: 10.1103/PhysRevE.74.031116 [17] X. Hu, F. Liu, I. Turner and V. Anh, An implicit numerical method of a new time distributed-order and two-sided space-fractional advection-dispersion equation, Numer. Algor., 2016, 72(2), 393-407. doi: 10.1007/s11075-015-0051-1 [18] H. Jiang, F. Liu, I. Turner and K. Burrage, Analytical solutions for the multiterm time-space Caputo-Riesz fractional advection-diffusion equations on a finite domain, J. Math. Anal. Appl., 2012, 389(2), 1117-1127. doi: 10.1016/j.jmaa.2011.12.055 [19] J. T. Katsikadelis, Numerical solution of distributed order fractional differential equations, J. Comput. Phys., 2014, 259, 11-22. doi: 10.1016/j.jcp.2013.11.013 [20] A. N. Kochubei, Distributed order calculus and equations of ultraslow diffusion, J. Math. Anal. Appl., 2008, 340(1), 252-281. doi: 10.1016/j.jmaa.2007.08.024 [21] S. L. Lei, X. Chen and X. Zhang, Multilevel circulant preconditioner for highdimensional fractional diffusion equations, East Asian J. Appl. Math., 2016, 6(2), 109-130. doi: 10.4208/eajam.060815.180116a [22] S. L. Lei and H. W. Sun, A circulant preconditioner for fractional diffusion equations, J. Comput. Phys., 2013, 242, 715-725. doi: 10.1016/j.jcp.2013.02.025 [23] C. Li and H. Ding, Higher order finite difference method for the reaction and anomalous-diffusion equation, Appl. Math. Model., 2014, 38(15), 3802-3821. [24] H. Li, X. Wu, J. Zhang et al., Numerical solution of the time-fractional subdiffusion equation on an unbounded domain in two-dimensional space, East. Asia. J. Appl. Math., 2017, 7(3), 439-454. doi: 10.4208/eajam.031116.080317a [25] Z. Li, Y. Luchko and M. Yamamoto, Analyticity of solutions to a distributed order time-fractional diffusion equation and its application to an inverse problem, Comput. Math. Appl., 2017, 73(6), 1041-1052. doi: 10.1016/j.camwa.2016.06.030 [26] F. Liu, M. M. Meerschaert, R. J. McGough et al., Numerical methods for solving the multi-term time-fractional wave-diffusion equation, Fract. Calc. Appl. Anal., 2013, 16(1), 9-25. [27] F. Liu, C. Yang and K. Burrage, Numerical method and analytical technique of the modified anomalous subdiffusion equation with a nonlinear source term, J. Comput. Appl. Math., 2009, 231(1), 160-176. doi: 10.1016/j.cam.2009.02.013 [28] Q. Liu, F. Liu, I. Turner and V. Anh, Finite element approximation for a modified anomalous subdiffusion equation, Appl. Math. Model., 2011, 35(8), 4103-4116. doi: 10.1016/j.apm.2011.02.036 [29] R. L. Magin, O. Abdullah, D. Baleanu and X. J. Zhou, Anomalous diffusion expressed through fractional order differential operators in the Bloch-Torrey equation, J. Magn. Reson., 2008, 190(2), 255-270. doi: 10.1016/j.jmr.2007.11.007 [30] S. Mashayekhi and M. Razzaghi, Numerical solution of distributed order fractional differential equations by hybrid functions, J. Comput. Phys., 2016, 315, 169-181. doi: 10.1016/j.jcp.2016.01.041 [31] M. M. Meerschaert, E. Nane and P. Vellaisamy, Distributed-order fractional diffusions on bounded domains, J. Math. Anal. Appl., 2011, 379(1), 216-228. doi: 10.1016/j.jmaa.2010.12.056 [32] D. Rui, Z. P. Hao and Z. Z. Sun, Lubich second-order methods for distributedorder time-fractional differential equations with smooth solutions, East. Asia. J. Appl. Math., 2016, 6(2), 131-151. doi: 10.4208/eajam.020615.030216a [33] H. Scher and E. W. Montroll, Anomalous transit-time dispersion in amorphous solids, Phys. Rev. B, 1975, 12(6), 2455. [34] W. Schneider and W. Wyss, Fractional diffusion and wave equations, J. Math. Phys., 1989, 30(1), 134-144. doi: 10.1063/1.528578 [35] Z.-Z. Sun and G.-H. Gao, Finite Difference Methods for the Fractional Differential Equations, Science Press, Beijing, 2015. (in Chinese). [36] K. Wang and H. Wang, A fast characteristic finite difference method for fractional advection-diffusion equations, Adv. Water Resour., 2011, 34(7), 810-816. doi: 10.1016/j.advwatres.2010.11.003 [37] H. Ye, F. Liu, V. Anh and I. Turner, Numerical analysis for the time distributed-order and Riesz space fractional diffusions on bounded domains, IMA J. Appl. Math., 2015, 80(3), 825-838. doi: 10.1093/imamat/hxu015 [38] M. A. Zaky, A Legendre collocation method for distributed-order fractional optimal control problems, Nonlinear Dyn., 2018, 91, 2667-2681. doi: 10.1007/s11071-017-4038-4 [39] M. A. Zaky, A Legendre spectral quadrature tau method for the multi-term time-fractional diffusion equations, Comp. Appl. Math., 2018, 37, 3525-3538. doi: 10.1007/s40314-017-0530-1 [40] M. A. Zaky, E. H. Doha and J. A. T. Machado, A spectral numerical method for solving distributed-order fractional initial value problems, J. Comput. Nonlinear Dynam., 2018, 13(10), 101007. doi: 10.1115/1.4041030 [41] M. A. Zaky and J. A. T. Machado, On the formulation and numerical simulation of distributed-order fractional optimal control problems, Commun. Nonlinear Sci. Numer. Simulat., 2017, 52, 177-189. doi: 10.1016/j.cnsns.2017.04.026 [42] X. L. Zhao, T. Z. Huang, S. L. Wu and Y. F. Jing, DCT- and DST-based splitting methods for Toeplitz systems, Int. J. Comput. Math., 2012, 89(5), 691-700. doi: 10.1080/00207160.2011.649264 -
-
Figures(6) / Tables(13)
Export File
Citation
Huanyan Jian, Tingzhu Huang, Xile Zhao, Yongliang Zhao. FAST SECOND-ORDER ACCURATE DIFFERENCE SCHEMES FOR TIME DISTRIBUTED-ORDER AND RIESZ SPACE FRACTIONAL DIFFUSION EQUATIONS[J]. Journal of Applied Analysis & Computation, 2019, 9(4): 1359-1392. doi: 10.11948/2156-907X.20180247
Format
Content
DownLoad:
-
Figure 1. Exact solutions (lines) and numerical solutions (symbols) of Example 4.1: (a)
$ \beta $ = 1.3 at$ T $ = 1.5 (stars), 1.2 (rhombus), 0.8 (triangles); (b)$ \beta $ = 1.8 at$ T $ = 1.5 (stars), 1.2 (rhombus), 0.8 (triangles) -
Figure 2. Spectrum of original matrice (red) and R. Chan's-based preconditioned matrice (blue) for Example 4.1 at time level (a)
$ n = 0 $ and (b)$ n = 1 $ , respectively, when$ M $ =$ N $ = 128,$ J $ = 50,$ \beta $ = 1.8, and$ T $ = 1.5. -
Figure 3. Spectrum of original matrice (red) and R. Chan's-based preconditioned matrice (blue) for Example 4.1 at time level (a)
$ n = 0 $ and (b)$ n = 1 $ , respectively, when$ M $ =$ N $ = 256,$ J $ = 50,$ \beta $ = 1.8, and$ T $ = 1.5 -
Figure 4. The solution surfaces obtained from Example 4.3 at
$ J $ = 50,$ \widetilde{M} $ = 40 and$ N $ = 10: (a) the exact solution with$ T = 1 $ ,$ \beta $ =$ \gamma $ = 1.8; (b) the numerical solution with$ T = 1 $ ,$ \beta $ =$ \gamma $ = 1.8 by the scheme (3.12)-(3.14); (c) the exact solution with$ T = 0.5 $ ,$ \beta $ =$ \gamma $ = 1.3; (d) the numerical solution with$ T = 0.5 $ ,$ \beta $ =$ \gamma $ = 1.3 by the scheme (3.12)-(3.14); -
Figure 5. Spectrum of original matrice (red) and R. Chan's-based preconditioned matrice (blue) for Example 4.3 at time level (a)
$ n = 0 $ and (b)$ n = 1 $ , respectively, when$ \widetilde{M} $ =$ N $ = 64,$ J $ = 50,$ \beta $ =$ \gamma $ = 1.5, and$ T $ = 1.5 -
Figure 6. Spectrum of original matrice (red) and R. Chan's-based preconditioned matrice (blue) for Example 4.3 at time level (a)
$ n = 0 $ and (b)$ n = 1 $ , respectively, when$ \widetilde{M} $ =$ N $ = 128,$ J $ = 50,$ \beta $ =$ \gamma $ = 1.5, and$ T $ = 1.5