GLOBAL ANALYSIS OF AN AGE-STRUCTURED SEIR MODEL WITH IMMIGRATION OF POPULATION AND NONLINEAR INCIDENCE RATE

Ran Zhang; Dan Li; Shengqiang Liu

doi:10.11948/2156-907X.20180281

2019 Volume 9 Issue 4

Article Contents

Article navigation > Journal of Applied Analysis & Computation > 2019 > 9(4): 1470-1492

Next Article Previous Article

Ran Zhang, Dan Li, Shengqiang Liu. GLOBAL ANALYSIS OF AN AGE-STRUCTURED SEIR MODEL WITH IMMIGRATION OF POPULATION AND NONLINEAR INCIDENCE RATE[J]. Journal of Applied Analysis & Computation, 2019, 9(4): 1470-1492. doi: 10.11948/2156-907X.20180281

Citation:

Ran Zhang, Dan Li, Shengqiang Liu. GLOBAL ANALYSIS OF AN AGE-STRUCTURED SEIR MODEL WITH IMMIGRATION OF POPULATION AND NONLINEAR INCIDENCE RATE[J]. Journal of Applied Analysis & Computation, 2019, 9(4): 1470-1492. doi: 10.11948/2156-907X.20180281

GLOBAL ANALYSIS OF AN AGE-STRUCTURED SEIR MODEL WITH IMMIGRATION OF POPULATION AND NONLINEAR INCIDENCE RATE

1.
School of Mathematics, Harbin Institute of Technology, Harbin 150001, China
2.
School of Mathematical Science, Huaiyin Normal University, Huaian 223300, China

Corresponding author: Email address: sqliu@hit.edu.cn(S. Liu)
Fund Project: The authors were supported by National Natural Science Foundation of China(Nos.11801209, 11871179 and 11771374), and Natural Science Foundation of the Jiangsu Higher Education Institutions of China(No. 18KJB110004)

Abstract

Abstract

Epidemic models with infection age of infectious individuals have been extensively studied, however, most of the existing works ignore the combined effects of immigration and nonlinear incidence. In this paper, we incorporate both the effects of immigration and nonlinear incidence, based on which we formulate an SEIR epidemic model. We give a rigorous mathematical analysis on some necessary technical materials. Then, by constructing a Lyapunov functional, we show that the endemic equilibrium is globally asymptotically stable. Numerical simulations of an application are given to support our theoretical results.
- Lyapunov functional /
- global stability /
- infection age /
- immigration /
- nonlinear incidence
MSC: 35Q92, 37N25, 92D30

FullText(HTML)

References

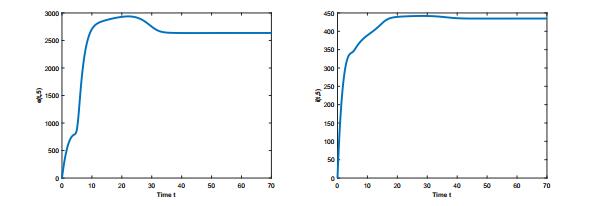
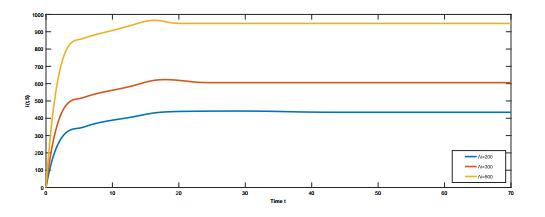
[1]	V. Akimenko, An age-structured SIR epidemic model with fixed incubation period of infection, Comput. Math. Appl., 2017, 73, 1485-1504. doi: 10.1016/j.camwa.2017.01.022 CrossRef Google Scholar
[2]	R. M. Anderson and R. M. May, Infectious Diseases in Humans: Dynamics and Control, Oxford University Press, Oxford, 1991. Google Scholar
[3]	S. M. Blower, A. R. Mclean, T. C. Porco et al., The intrinsic transmission dynamics of tuberculosis epidemics, Nat. Med., 1995, 1, 815-821. doi: 10.1038/nm0895-815 CrossRef Google Scholar
[4]	F. Brauer and P. van den Driessche, Models for transmission of disease with immigration of infectives, Math. Biosci., 2001, 171, 143-154. doi: 10.1016/S0025-5564(01)00057-8 CrossRef Google Scholar
[5]	V. Capasso and G. Serio, A generalization of the Kermack-Mackendric deterministic model, Math. Biosci., 1978, 42, 43-61. doi: 10.1016/0025-5564(78)90006-8 CrossRef Google Scholar
[6]	C. Castillo Chavez and B. Song, Dynamical models of tuberculosis and their applications, Math. Biosci. Eng., 2004, 1, 361-404. doi: 10.3934/mbe CrossRef Google Scholar
[7]	Y. Chen, J. Yang and F. Zhang, The global stability of an SIRS model with infection age, Math. Biosci. Eng., 2014, 11, 449-469. doi: 10.3934/mbe CrossRef Google Scholar
[8]	Y. Chen, S. Zou and J. Yang, Global analysis of an SIR epidemic model with infection age and saturated incidence, Nonlinear Anal.: Real World Appl., 2016, 30, 16-31. doi: 10.1016/j.nonrwa.2015.11.001 CrossRef Google Scholar
[9]	W. R. Derrick and P. van den Driessche, Homoclinic orbits in a disease transmission model with nonlinear incidence and nonconstant population, Discret. Contin. Dyn. Syst. Ser. B, 2003, 3, 299-309. doi: 10.3934/dcdsb CrossRef Google Scholar
[10]	X. Duan, S. Yuan, Z. Qiu and J. Ma, Global stability of an SVEIR epidemic model with ages of vaccination and latency, Comput. Math. Appl., 2016, 68, 288-308. Google Scholar
[11]	A. Ducrot, P. Magal and O. Seydi, Singular perturbation for an abstract non-densely defined cauchy problem, J. Evol. Equ., 2017, 17, 1089-1128. doi: 10.1007/s00028-016-0374-y CrossRef Google Scholar
[12]	W. E. Fitzgibbon, J. J. Morgan, G. F. Webb and Y. Wu, A vector-host epidemic model with spatial structure and age of infection, Nonlinear Anal.: Real World Appl., 2018, 41, 692-705. doi: 10.1016/j.nonrwa.2017.11.005 CrossRef Google Scholar
[13]	R. Gao, B. Cao, Y. Hu et al., Human infection with a novel avian-origin influenza A(H7N9) virus, N. Engl. J. Med., 2013, 368, 1888-1897. Google Scholar
[14]	J. K. Hale, Asymptotic Behavior of Dissipative Systems, Mathematical Surveys and Monographs Vol. 25, American Mathematical Society, Providence, RI, 1988. Google Scholar
[15]	H. W. Hethcote and P. van den Driessche, Some epidemiological models with nonlinear incidence, J. Math. Biol., 1991, 29, 271-287. doi: 10.1007/BF00160539 CrossRef Google Scholar
[16]	M. Iannelli, Mathematical Theory of Age-Structured Population Dynamics, Giardini Editori e Stampatori in Pisa, 1995. Google Scholar
[17]	H. Inaba, R. Saito and N. Baca$\ddot{\textrm{e}}$r, An age-structured epidemic model for the demographic transition, Comput. Math. Appl., 2017, 73, 1485-1504. doi: 10.1016/j.camwa.2017.01.022 CrossRef Google Scholar
[18]	W. Kermack and A. McKendrick, A contribution to mathematical theory of epidemics, Proc Roy Soc Lond A, 1927, 115, 700-721. doi: 10.1098/rspa.1927.0118 CrossRef Google Scholar
[19]	A. Khan and G. Zaman, Global analysis of an age-structured SEIR endemic model, Chaos Soliton. Fract., 2018, 108, 154-165. doi: 10.1016/j.chaos.2018.01.037 CrossRef Google Scholar
[20]	A. Korobeinikov, Lyapunov functions and global properties for SEIR and SEIS epidemic models, Math. Med. Biol., 2004, 21, 75-83. doi: 10.1093/imammb/21.2.75 CrossRef Google Scholar
[21]	A. Korobeinikov and P. K. Maini, Nonlinear incidence and stability of infectious disease models, Math. Med. Biol., 2005, 22, 113-128. doi: 10.1093/imammb/dqi001 CrossRef Google Scholar
[22]	J. Li and F. Brauer, Continuous-time age-structured models in population dynamics and epidemiology, in: F. Brauer, P. van den Driessche and J. Wu (Eds), Mathematical Epidemiology, Lecture Notes in Mathematics Vol. 1945, Springer-Verlag, Berlin, 2008. Google Scholar
[23]	J. Li, Y. Yang, Y. Xiao and S. Liu, A class of Lyapunov functions and the global stability of some epidemic models with nonlinear incidence, J. Appl. Anal. Comput., 2016, 6(1), 38-46. Google Scholar
[24]	M. Y. Li and J. Muldowney, Global stability for the SEIR model in epidemiology, Math. Biosci., 1995, 12, 155-164. Google Scholar
[25]	L. Liu, J. Wang and X. Liu, Global stability of an SEIR epidemic model with age-dependent latency and relapse, Nonlinear Anal.: Real World Appl., 2015, 24, 18-35. doi: 10.1016/j.nonrwa.2015.01.001 CrossRef Google Scholar
[26]	S. Liu, X. Xie and J. Tang, Competing population model with nonlinear intraspecific regulation and maturation delays, Int. J. Biomath., 2012, 5, 1260007:1-22. doi: 10.1142/S1793524512600078 CrossRef Google Scholar
[27]	W. M. Liu, S. A. Levin and X. Iwasa, Influence of nonlinear incidence rates upon the behaviour of SIRS epidemiological models, J. Math. Biol., 1986, 23, 187-204. doi: 10.1007/BF00276956 CrossRef Google Scholar
[28]	P. Magal, C. C. McCluskey and G. F. Webb, Lyapunov functional and global asymptotic stability for an infection-age model, Appl. Anal., 2010, 89, 1109-1140. doi: 10.1080/00036810903208122 CrossRef Google Scholar
[29]	R. M. May and R. M. Anderson, Regulation and stability of host-parasite population interactions: Ⅱ. destabilizing processes, J. Anim. Ecol., 1978, 249-267. Google Scholar
[30]	C. C. McCluskey, Global stability for an SEI model of infectious disease with age structure and immigration of infecteds, Math. Biosci. Eng., 2016, 13, 381-400. Google Scholar
[31]	S. J. Olsen, H. L. Chang, T. Y. Cheung et al, Transmission of the severe acute respiratory syndrome on aircraft, N. Engl. J. Med., 2003, 349, 2416-2422. Google Scholar
[32]	R. Ross, The Prevention of Malaria, John Murray, London, 1911. Google Scholar
[33]	G. Rost and J. Wu, SEIR epidemiological model with varying infectivity and infinite delay, Math. Biosci. Eng., 2008, 5, 389-402. doi: 10.3934/mbe CrossRef Google Scholar
[34]	R. P. Sigdel and C. C. McCluskey, Global stability for an SEI model of infectious disease with immigration, Appl. Math. Comput., 2014, 243, 684-689. Google Scholar
[35]	H. L. Smith, Subharmonic bifurcation in an SIR epidemic model, J. Math. Biol., 1983, 17, 163-177. doi: 10.1007/BF00305757 CrossRef Google Scholar
[36]	H. L. Smith and H. R. Thieme, Dynamical Systems and Population Persistence, Graduate Studies in Mathematics Vol. 118, American Mathematical Society, Providence, RI, 2011. Google Scholar
[37]	B. Soufiane and T. M. Touaoula, Global analysis of an infection age model with a class of nonlinear incidence rates, J. Math. Anal. Appl., 2016, 434, 1211-1239. doi: 10.1016/j.jmaa.2015.09.066 CrossRef Google Scholar
[38]	K. Styblo, D. Frencly and T. Petty, Tuberculosis control and surveillance, Recent Adv. Respir. Med., 1986, 4, 77-108. Google Scholar
[39]	J. Wang, M. Guo and S. Liu, SVIR epidemic model with age structure in susceptibility, vaccination effects and relapse, IMA J. Appl. Math., 2018, 82, 945-970. Google Scholar
[40]	J. Wang, R. Zhang and T. Kuniya, The dynamics of an SVIR epidemiological model with infection age, IMA J. Appl. Math., 2016, 81, 321-343. doi: 10.1093/imamat/hxv039 CrossRef Google Scholar
[41]	L. Wang and X. Wang, Influence of temporary migration on the transmission of infectious diseases in a migrants' home village, J. Theoret. Biol., 2012, 300, 100-109. doi: 10.1016/j.jtbi.2012.01.004 CrossRef Google Scholar
[42]	X. Wang, S. Liu, L. Wang and W. Zhang, An epidemic patchy model with entry-exit screening, Bull. Math. Biol., 2015, 77, 1237-1255. doi: 10.1007/s11538-015-0084-6 CrossRef Google Scholar
[43]	G. F. Webb, An age-dependent epidemic model with spatial diffusion, Arch. Ration. Mech. An., 1980, 75, 91-102. doi: 10.1007/BF00284623 CrossRef Google Scholar
[44]	G. F. Webb, Theory of Nonlinear Age-Dependent Population Dynamics, Marcel Dekker, New York, 1985. Google Scholar
[45]	G. F. Webb and C. J. Browne, A model of the Ebola epidemics in West Africa incorporating age of infection, J. Biol. Dyna., 2016, 10, 18-30. doi: 10.1080/17513758.2015.1090632 CrossRef Google Scholar
[46]	World Health Organization, Fact sheets on Tuberculosis, www.who.int/tb. Accessed March 2019. Google Scholar
[47]	Y. Yang, S. Tang, X. Ren et al., Global stability and optimal control for a tuberculosis model with vaccination and treatment, Discret. Contin. Dyn. Syst. Ser. B, 2016, 21, 1009-1022. doi: 10.3934/dcdsb CrossRef Google Scholar
[48]	H. Yu, S. Cauchemez et al., Transmission dynamics, border entry screening, and school holidays during the 2009 influenza A (H1N1) pandemic, Emerg. Infect. Dis., 2012, 18, 758-766. doi: 10.3201/eid1805.110356 CrossRef Google Scholar
[49]	T. Zhang and Z. Teng, Pulse vaccination delayed SEIRS epidemic model with saturation incidence, Appl. Math. Model, 2008, 32, 1403-1416. doi: 10.1016/j.apm.2007.06.005 CrossRef Google Scholar