| Citation: |
Zhenzhen Li, Binxiang Dai. ANALYSIS OF DYNAMICS IN A GENERAL INTRAGUILD PREDATION MODEL WITH INTRASPECIFIC COMPETITION[J]. Journal of Applied Analysis & Computation, 2019, 9(4): 1493-1526. doi: 10.11948/2156-907X.20180296
|
ANALYSIS OF DYNAMICS IN A GENERAL INTRAGUILD PREDATION MODEL WITH INTRASPECIFIC COMPETITION
-
Abstract
This paper is devoted to studying the dynamical properties of a general intraguild predation model with intraspecific competition. We first investigate the stability of all possible equilibria in relation to the ecological parameters, and then study the long time behavior of the solution. Moreover, we provide a detailed analysis of dynamics of a IGP model with linear functional response and intraspecific competition. Our results show that the impact of the intraspecific competition essentially increases the dynamical complexity of the system. -
-
References
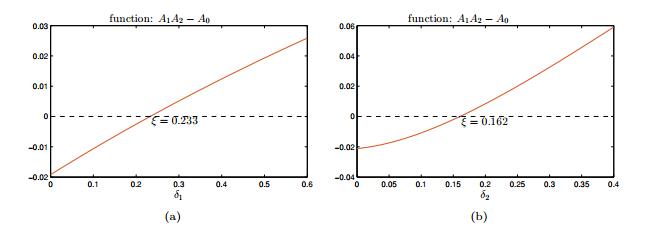
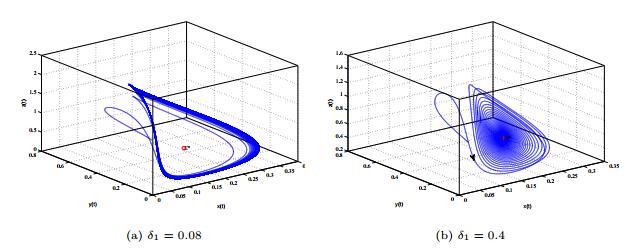
[1] A. Atabaigi, Bifurcation and chaos in a discrete time predator-prey system of Leslie type with generalized Holling type Ⅲ functional response, J. Appl. Anal. Comput., 2017, 7(2), 411-426. [2] P. Amarasekare, Coexistence of intraguild predators and prey in resource-rich environments, Ecol., 2008, 89(10), 2786-2797. doi: 10.1890/07-1508.1 [3] P. Abrams, S. Fung, Prey persistence and abundance in systems with intraguild predation and type-2 functional response, J. Theor. Biol., 2010, 264, 1033-1042. doi: 10.1016/j.jtbi.2010.02.045 [4] D. Bolnick, Can intraspecific competition drive disruptive selection? an experimental test in natural populations of sticklebacks, Evol., 2004, 58(3), 608-618. [5] F. Brauer, C. Castillo Chavez, Mathematical Models in Population Biology and Epidemiology (Second Edition), Springer, New York, 2012. [6] G. Butler, H. Freedman, P. Waltman, Uniformly persistent systems, Proc. Amer. Math. Soc., 1986, 96(3), 425-430. doi: 10.1090/S0002-9939-1986-0822433-4 [7] S. Chow, C. Li, D. Wang, Normal Forms and Bifurcation of Planar Vector Fields, Cambridge University Press, Cambridge, 1994. [8] C. Dye, Intraspecific competition amongst larval Aedes aegypti food exploitation or chemical interference, Ecol. Entomol., 1982, 7, 39-46. doi: 10.1111/j.1365-2311.1982.tb00642.x [9] H. Freedman, P. Waltman, Persistence in models of three interacting predator-prey populations, Math. Biosci., 1984, 68(2), 213-231. doi: 10.1016/0025-5564(84)90032-4 [10] H. Freedman, S. Ruan, M. Tang, Uniform persistence and flows near a closed positively invariant set, J. Dynam. Diff. Eqs., 1994, 6(4), 583-600. doi: 10.1007/BF02218848 [11] M. Freeze, Y. Chang, W. Feng, Analysis of dynamics in a complex food chain with ratio-dependent functional response, JAAC, 2014, 4(1), 69-87. [12] C. Holling, The components of predation as revealed by a study of small mammal predation of the European Pine Sawfly, Can. Entomol., 1959, 91, 293-320. doi: 10.4039/Ent91293-5 [13] R. Holt, G. Polis, A theoretical framework for intraguild predation, Am. Nat., 1997, 149, 745-764. doi: 10.1086/286018 [14] R. Han, B. Dai, Spatiotemporal dynamics and Hopf bifurcation in a delayed diffusive intraguild predation model with Holling Ⅱ functional response, Int. J. Bifurcat.Chaos, 2016, 26(12), 1-31. [15] R. Han, B. Dai, Spatiotemporal dynamics and spatial pattern in a diffusive intraguild predation model with delay effect, Appl. Math. Comput., 2017, 312, 177-201. [16] R. Han, B. Dai, L. Wang, Delay induced spatiotemporal patterns in a diffusive intraguild predation model with Beddington-DeAngelis functional response, Math. Biosci. Eng., 2018, 15(3), 595-627. [17] S. Hsu, S. Ruan, T. Yang, Analysis of three species Lotka-Volterra food web models with omnivory, J. Math. Anal. Appl., 2015, 426(2), 659-687. [18] T. Hanse, N. Stenseth, H. Henttonen, J. Tast, Interspecific and intraspecific competition as causes of direct and delayed density dependence in a fluctuating vole population, Proc. Natl. Acad. Sci., 1999, 96, 986-991. doi: 10.1073/pnas.96.3.986 [19] A. Korobeinikov, Stability of ecosystem: global properties of a general predator-prey model, Math. Medic. Biol., 2009, 26, 309-321. doi: 10.1093/imammb/dqp009 [20] Y. Kuznetsov, Elements of Applied Bifurcation Theory (Third Edition), Springer, New York, 2004. [21] Y. Kang, L. Wedekin, Dynamics of a intraguild predation model with generalist or specialist predator, J. Math. Biol., 2013, 67(5), 1227-1259. doi: 10.1007/s00285-012-0584-z [22] M. Van Kleunen, M. Fischer, B. Schmid, Effects of intraspecific competition on size variation and reproductive allocation in a clonal plant, OIKOS, 2001, 94, 515-524. doi: 10.1034/j.1600-0706.2001.940313.x [23] Z. Li, B. Dai, Global dynamics of delayed intraguild predation model with intraspecific competition, Int. J. Biomath., 2018, 11(8), 1-39. [24] K. Mischaikow, H. Smith, H. Thieme, Asymptotically autonomous semiflows: chain recurrence and Lyapunov functions, Trans. Amer. Math. Soc., 1995, 347(5), 1669-1685. doi: 10.1090/tran/1995-347-05 [25] C. Pao, Nonlinear parabolic and elliptic equations, Plenum Press, New York and London, 1992. [26] G. Polis, R. Holt, Intraguild predation: the dynamics of complex trophic interactions, Trends Ecol. Evol., 1992, 7, 151-154. doi: 10.1016/0169-5347(92)90208-S [27] M. Peng, Z. Zhang, X. Wang, X. Liu, Hopf bifurcation analysis for a delayed predator-prey system with a prey refuge and selective harvesting, J. Appl. Anal. Comput., 2018, 8(3), 982-997. [28] S. Ruan, Oscillations in plankton models with nutrient recycling, J. Theor. Biol., 2001, 208(1), 15-26. [29] H. Shu, X. Hu, L. Wang, J. Watmough, Delay induced stability switch, multitype bistability and chaos in an intraguild predation model, J. Math. Biol., 2015, 71, 1269-1298. doi: 10.1007/s00285-015-0857-4 [30] A. Verdy, P. Amarasekare, Alternative stable states in communities with intraguild predation, J. Theor. Biol., 2010, 262(1), 116-128. doi: 10.1016/j.jtbi.2009.09.011 [31] Y. Wang, D. DeAngelis, Stability of an intraguild predation system with mutual predation, Commun. Nonl. Sci. Numer. Simul., 2016, 33, 141-159. doi: 10.1016/j.cnsns.2015.09.004 [32] Y. Wang, W. Jiang, Bifurcations in a delayed differential-algebraic plankton economic system, J. Appl. Anal. Comput., 2017, 7(4), 1431-1447. -
-
Figures(10) / Tables(1)
Export File
Citation
Zhenzhen Li, Binxiang Dai. ANALYSIS OF DYNAMICS IN A GENERAL INTRAGUILD PREDATION MODEL WITH INTRASPECIFIC COMPETITION[J]. Journal of Applied Analysis & Computation, 2019, 9(4): 1493-1526. doi: 10.11948/2156-907X.20180296
Format
Content
DownLoad:
-
Figure 1. The two possible generic cases for the intersection of the two curves
$ C_1 $ and$ C_2 $ . -
Figure 2. The two possible generic cases for the intersection of the two curves
$ C_3 $ and$ C_4 $ . -
Figure 3. All generic possibilities of classification of parameters with varied
$ e_2 $ in regions (1)–(6) with$ \gamma_1 > e_1 $ . -
Figure 4. The four possible generic cases for the intersection of the two straight lines
$ L_1 $ and$ L_2 $ for category (Ⅱ). -
Figure 5. The two possible generic cases for the intersection of the two straight lines
$ L_1 $ and$ L_2 $ for category (Ⅲ). -
Figure 6. The two possible generic cases for the intersection of the two straight lines
$ L_1 $ and$ L_2 $ for category (Ⅳ). -
Figure 7. A typical picture of the parameter space with varied
$ \gamma_2, \gamma_3 $ and fixed$ e_1, e_2, \delta_1, \delta_2, \gamma_1, c $ with$ \gamma_1 > e_1 $ . The dynamics in each region of the parameter space are indicated with different color. First, in the yellow regions species$ z $ dies out eventually because of results in Propositions 5.5, 5.6(ⅰ) and 5.8. In the orange region, species y dies out eventually (Propositions 5.6(ⅱ) and 5.7). Moreover, in the green region, the bistability phenomenon occurs (Proposition 5.6(ⅲ)). Finally, the positive equilibrium appears in the cyan region and the permanence effect of the populations of the model (5.2) follows (Proposition 5.11). -
Figure 8. Hopf bifurcation curve of system (5.2) with parameter condition
$ C_1 $ . When$ (\delta_1, \delta_2)\in $ (Ⅰ),$ F(\delta_1, \delta_2) = A_2A_1-A_0 < 0 $ . When$ (\delta_1, \delta_2)\in $ (Ⅱ),$ F(\delta_1, \delta_2) = A_2A_1-A_0 > 0 $ . -
Figure 9. (a) The graph of
$ F $ in terms of$ \delta_1 $ . (b) The graph of$ F $ in terms of$ \delta_2 $ . -
Figure 10. Two numerical solutions of system (5.2) with parameter condition
$ (C_1) $ . (a) A periodic solution bifurcates from the positive equilibrium via Hopf bifurcation when$ \delta_1 = 0.08 $ . (b) The positive equilibrium$ E^* $ is locally asymptotically stable when$ \delta_1 = 0.4 $ . The initial condition is:$ (x_0, y_0, z_0) = (0.19, 0.5, 0.3) $ .