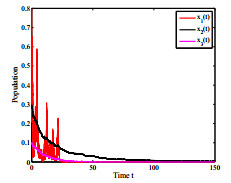
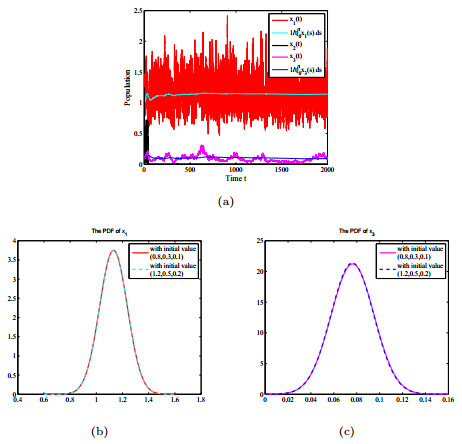
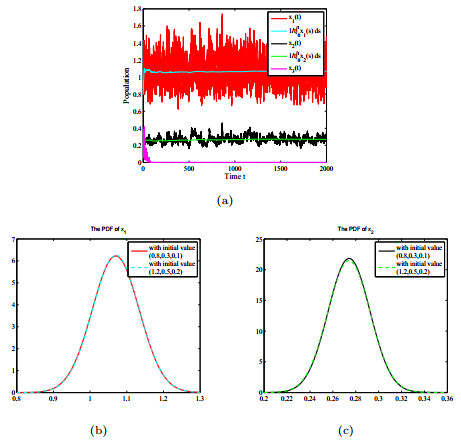
|
[1]
|
A. Al-Khedhairi, A. A. Elsadany, A. Elsonbaty and A. G. Abdelwahab, Dynamical study of a chaotic predator-prey model with an omnivore, Eur. Phys. J. Plus, 2018, 133(1), 29. doi: 10.1140/epjp/i2018-11864-8
CrossRef Google Scholar
|
|
[2]
|
J. Bao, X. Mao, G. Yin and C. Yuan, Competitive Lotka-Volterra population dynamics with jumps, Nonlinear Anal., 2011, 74, 6601–6616. doi: 10.1016/j.na.2011.06.043
CrossRef Google Scholar
|
|
[3]
|
E. Bonyah, A. Atangana and A. A. Elsadany, A fractional model for predator-prey with omnivore, Chaos, 2019, 29(1), 013136. doi: 10.1063/1.5079512
CrossRef Google Scholar
|
|
[4]
|
R. Hall, Intraguild predation in the presence of a shared natural enemy, Ecology, 2011, 92, 352–361. doi: 10.1890/09-2314.1
CrossRef Google Scholar
|
|
[5]
|
S. Hsu, S. Ruan and T. Yang, Analysis of three species Lotka-Volterra food web models with omnivory, J. Math. Anal. Appl., 2015, 426, 659–687. doi: 10.1016/j.jmaa.2015.01.035
CrossRef Google Scholar
|
|
[6]
|
C. Ji, D. Jiang and X. Li, Qualitative analysis of a stochastic ratio-dependent predator-prey system, J. Comput. Appl. Math., 2011, 235, 1326–1341. doi: 10.1016/j.cam.2010.08.021
CrossRef Google Scholar
|
|
[7]
|
C. Ji, D. Jiang and N. Shi, Analysis of a predator-prey model with modified Leslie-Gowerand Holling-type Ⅱ schemes with stochastic perturbation, J. Math. Anal. Appl., 2009, 359, 482–498. doi: 10.1016/j.jmaa.2009.05.039
CrossRef Google Scholar
|
|
[8]
|
G. Jing, M. Li and Y. Zhang, Stability of a stochastic one-predator-two-prey population model with time delays, Commun. Nonlinear Sci. Numer. Simulat., 2017, 53, 65–82. doi: 10.1016/j.cnsns.2017.04.022
CrossRef Google Scholar
|
|
[9]
|
M. Jovanovi$\acute{c}$ and M. Krsti$\acute{c}$, Extinction in stochastic predator-prey population model with Allee effect on prey, Discret. Contin. Dyn. Syst. Ser. B, 2017, 22, 2651–2667.
Google Scholar
|
|
[10]
|
H. Liu, T. Li and F. Zhang, A prey-predator model with Holling Ⅱ functional response and the carrying capacity of predator depending on its prey, J. Appl. Anal. Comput., 2018, 8, 1464–1474.
Google Scholar
|
|
[11]
|
M. Liu and C. Z. Bai, Analysis of a stochastic tri-trophic food-chain model with harvesting, J. Math. Biol., 2016, 73, 597–625. doi: 10.1007/s00285-016-0970-z
CrossRef Google Scholar
|
|
[12]
|
M. Liu and M. Fan, Stability in distribution of a three-species stochastic cascade predator-prey system with time delays, IMA J. Appl. Math., 2017, 82, 396–423.
Google Scholar
|
|
[13]
|
M. Liu, H. Qiu and W. K, A remark on a stochastic predator-prey system with time delays, Appl. Math. Lett., 2013, 26, 318–323. doi: 10.1016/j.aml.2012.08.015
CrossRef Google Scholar
|
|
[14]
|
A. Maiti, M. M. Jana and G. P. Samanta, Deterministic and stochastic analysis of a ratio-dependent predator-prey system with delay, Nonlinear Anal. Model. Control, 2007, 12, 383–398. doi: 10.15388/NA.2007.12.3.14700
CrossRef Google Scholar
|
|
[15]
|
X. Mao, Stochsatic Differential Equations and Applications, Horwood Publishing Limited, Chichester, 2007.
Google Scholar
|
|
[16]
|
D. Mukherjee, Stability analysis of a stochastic model for prey-predator system with disease in the prey, Nonlinear Anal. Model. Control, 2003, 8, 83–92.
Google Scholar
|
|
[17]
|
M. Ouyang and X. Li, Permanence and asymptotical behavior of stochastic prey-predator system with Markovian switching, Appl. Math. Comput., 2015, 266, 539–559.
Google Scholar
|
|
[18]
|
S. Peng and X. Zhu, Necessary and sufficient condition for comparison theorem of 1-dimensional stochastic differential equations, Stochastic Process. Appl., 2006, 116, 370–380. doi: 10.1016/j.spa.2005.08.004
CrossRef Google Scholar
|
|
[19]
|
D. Prato and J. Zabczyk, Ergodicity for Infinite Dimensional Systems, Cambridge University Press, Cambridge, 1996.
Google Scholar
|
|
[20]
|
H. Qiu and W. Deng, Stationary distribution and global asymptotic stability of a three-species stochastic food-chain system, Turk. J. Math., 2017, 41, 1292–1307. doi: 10.3906/mat-1510-52
CrossRef Google Scholar
|
|
[21]
|
S. Sadhu and C. Kuehn, Stochastic mixed-mode oscillations in a three-species predator-prey model, Chaos, 2018, 28(3), 033606. doi: 10.1063/1.4994830
CrossRef Google Scholar
|
|
[22]
|
D. Sen, S. Ghorai and M. Banerjee, Complex dynamics of a three species prey-predator model with intraguild predation, Ecol. Complex., 2018, 34, 9–22. doi: 10.1016/j.ecocom.2018.02.002
CrossRef Google Scholar
|
|
[23]
|
J. Yang and S. Tang, Holling type Ⅱ predator-prey model with nonlinear pulse as state-dependent feedback control, J. Comput. Appl. Math., 2016, 291, 225–241. doi: 10.1016/j.cam.2015.01.017
CrossRef Google Scholar
|
|
[24]
|
X. Yu, S. Yuan and T. Zhang, Persistence and ergodicity of a stochastic single species model with Allee effect under regime switching, Commun. Nonlinear Sci. Numer. Simulat., 2018, 59, 359–374. doi: 10.1016/j.cnsns.2017.11.028
CrossRef Google Scholar
|
|
[25]
|
T. Zeng, Z. D. Teng, Z. M. Li and J. N. Hu, Stability in the mean of a stochastic three species food chain model with general L$\acute{e}$vy jumps, Chaos Soliton. Fract., 2018, 106, 258–265. doi: 10.1016/j.chaos.2017.10.025
CrossRef Google Scholar
|




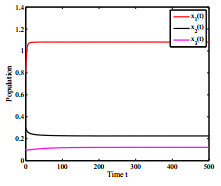

 DownLoad:
DownLoad: