| Citation: | Rong Wu, Yan Zhou. PEAKON AND PSEUDO-PEAKON IN A GENERALIZED CAMASSA-HOLM TYPE EQUATION[J]. Journal of Applied Analysis & Computation, 2021, 11(5): 2553-2560. doi: 10.11948/20210012 |
PEAKON AND PSEUDO-PEAKON IN A GENERALIZED CAMASSA-HOLM TYPE EQUATION
-
Abstract
This paper studies traveling wave solutions of a nonlinear generalization of the Camassa-Holm equation introduced by Anco et al. in 2015 and 2019. Under given parameter conditions, the corresponding traveling system is a singular system of the first class defined in [
8 ]. The bifurcations of traveling wave solutions in the parameter space are investigated from the perspective of dynamical systems. The existence of solitary wave solution, periodic peakon solutions and peakon, pseudo-peakon are proved. Possible exact explicit parametric representations of various solutions are given.-
Keywords:
- Solitary wave /
- peakon /
- pseudo-peakon /
- periodic peakon /
- bifurcation /
- Camassa-Holm type equation
-

-
References
[1] S. C. Anco, P. L. da Silva and I. L. Freire, A family of wave-breaking equations generalizing the Camassa-Holm and Novikov equations, J. Math. Phys., 2015, 56, 091506. doi: 10.1063/1.4929661 [2] S. C. Anco, E. Recio, M. L. Gandarias and M. S. Bruzon, A nonlinear generalization of the Camassa-Holm equation with peakon solutions Dynamical Systems, Diff. Equs. Appl., Proc. 10th AIMS Int. Conf. (Spain), 2015, 29-37. [3] S. C. Anco and E. Recio, A general family of multi-peakon equations and their properties, J. Phys. A: Math. Theor., 2019, 52, 125203 (37pp). doi: 10.1088/1751-8121/ab03dd [4] P. F. Byrd and M. D. Fridman, Handbook of Elliptic Integrals for Engineers and Sciensists, Springer, Berlin, 1971. [5] R. Cammasa and D. D. Holm, An integrable shallow water equation with peaked solution, Phys. Rev. Lett., 1993, 71, 1161-1164. [6] R. Cammasa, D. D. Holm and J. M. Hyman, A new integrable shallow water equation, Adv. Appl. Mech., 1994, 31, 1-33. [7] J. Li, Singular Nonlinear Traveling Wave Equations: Bifurcations and Exact Solutions, Science Press, Beijing, 2013. [8] J. Li and G. Chen, On a class of singular nonlinear traveling wave equations, Int. J. Bifur. Chaos, 2007, 17, 4049-4065. doi: 10.1142/S0218127407019858 [9] J. Li and Z. Qiao, Bifurcations and exact travelling wave solutions of the generalized two-component Camassa-Holm equation, Int. J. Bifur. Chaos, 2012, 22, 1250305. doi: 10.1142/S0218127412503051 [10] J. Li, Variform exact one-peakon solutions for some singular nonlinear traveling wave equations of the first kind, Int. J. Bifur. Chaos, 2014, 24, 1450160. doi: 10.1142/S0218127414501600 [11] J. Li, W. Zhu and G. Chen, Understanding peakons, periodic peakons and compactons via a shallow water wave equation, Int. J. Bifur. Chaos, 2016, 26, 1650207. doi: 10.1142/S0218127416502072 [12] T. Zhang, T. Xu, J. Wang and Z. Jiang, Homoclinic cycle and homoclinic bifurcations of a predator-prey model with impulsive state Feedback control, J. Nonlinear Mod. Anal., 2020, 2, 227-240. -
-
-
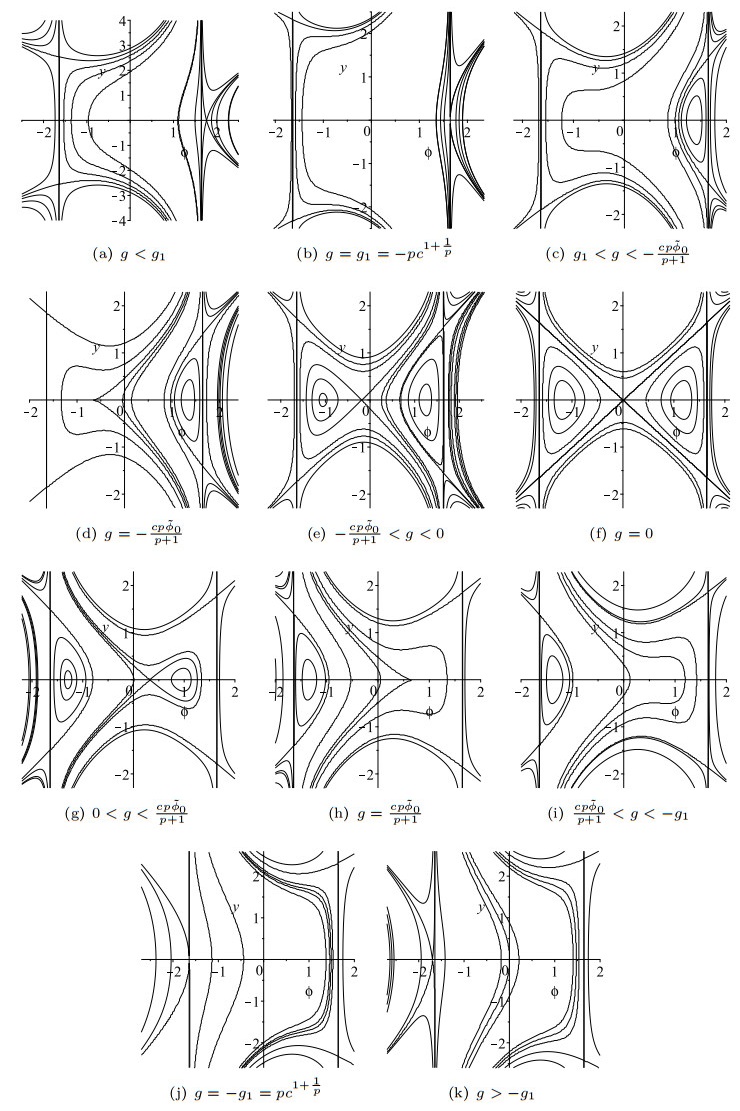
Figure 1. The bifurcations of phase portraits of system (1.3) when
$ p $ is not an even number -
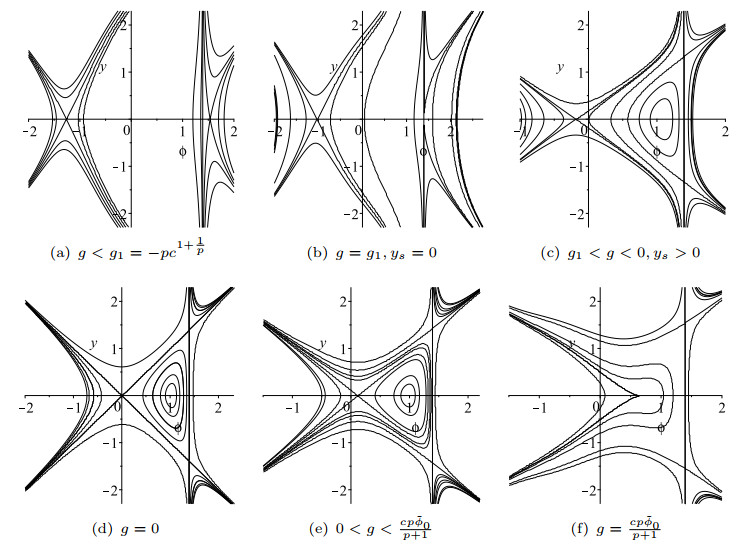
Figure 2. The bifurcations of phase portraits of system (1.3) when
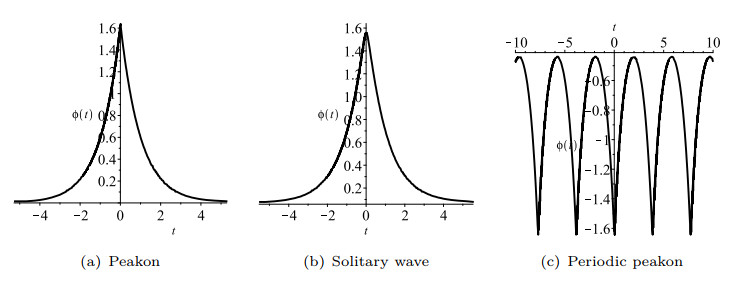
$ p $ is an even number - Figure 3. Profiles of traveling waves of equation (1.1)




 DownLoad:
DownLoad: