| Citation: |
Huiling Wu, Hongyu Wu, Quanyong Zhu, Jinxi Fei, Zhengyi Ma. SOLITON, BREATHER AND LUMP MOLECULES IN THE (2+1)-DIMENSIONAL B-TYPE KADOMTSEV-PETVIASHVILI-KORTEWEG DE-VRIES EQUATION[J]. Journal of Applied Analysis & Computation, 2022, 12(1): 230-244. doi: 10.11948/20210132
|
SOLITON, BREATHER AND LUMP MOLECULES IN THE (2+1)-DIMENSIONAL B-TYPE KADOMTSEV-PETVIASHVILI-KORTEWEG DE-VRIES EQUATION
-
Abstract
The $ N $-soliton solutions of the (2+1)-dimensional B-type Kadomtsev-Petviashvili-Korteweg de-Vries (BKP-KdV) equation are constructed through the Hirota bilinear method. By inserting the velocity resonance conditions into the soliton solutions, soliton molecule, breather molecule, breather-soliton molecule, lump-soliton molecule and lump-breather molecule are presented. The interaction of two soliton molecules, and the interaction between one soliton molecule and breather are discussed and shown to be the elastic collisions.
-
-
References
[1] Y. Cao, J. He and D. Mihalache, Families of exact solutions of a new extended (2+1)-dimensional Boussinesq equation, Nonlinear Dyn., 2018, 91(4), 2593-2605. doi: 10.1007/s11071-017-4033-9 [2] J. Dong, B. Li and M. Yuen, Soliton molecules and mixed solutions of the (2+1)-dimensional bidirectional Sawada-Kotera equation, Commun. Theor. Phys., 2020, 72(2), 025002. doi: 10.1088/1572-9494/ab6184 [3] Z. Dai, Z. Liu and D. Li, Exact periodic solitary-wave solution for KdV equation, Chin. Phys. Lett., 2008, 25(5), 1531-1533. doi: 10.1088/0256-307X/25/5/003 [4] L. Feng, S. Tian, X. Wang and T. Zhang, Rogue waves, homoclinic breather waves and soliton waves for the (2+1)-dimensional B-type Kadomtsev-Petviashvili equation, Appl. Math. Lett., 2017, 65, 90-97. doi: 10.1016/j.aml.2016.10.009 [5] W. Hu, Y. Gao, S. Jia, Q. Huang and Z. Lan, Periodic wave, breather wave and travelling wave solutions of a (2+1)-dimensional B-type Kadomtsev-Petviashvili equation in fluids or plasmas, Eur. Phys. J. Plus, 2016, 131(11), 390. doi: 10.1140/epjp/i2016-16390-1 [6] G. Herink, F. Kurtz, B. Jalali, D. R. Solli and C. Ropers, Real-time spectral interferometry probes the internal dynamics of femtosecond soliton molecules, Science, 2017, 356(6333), 50-54. doi: 10.1126/science.aal5326 [7] R. Hirota and M. Ito, Resonance of solitons in one dimension, J. Phys. Soc. Jpn., 1983, 52(3), 744-748. doi: 10.1143/JPSJ.52.744 [8] R. Hirota, The direct method in soliton theory, Cambridge university press, Cambridge, 2004. [9] M. Jia, J. Lin and S. Lou, Soliton and breather molecules in few-cycle-pulse optical model, Nonlinear Dyn., 2020, 100(4), 3745-3757. doi: 10.1007/s11071-020-05695-3 [10] Z. Lan, Y. Gao, J. Yang, C. Su and Q. Wang, Solitons, Bäcklund transformation and Lax pair for a (2+1)-dimensional B-type Kadomtsev-Petviashvili equation in the fluid/plasma mechanics, Mod. Phys. Lett. B, 2016, 30(25), 1650265. doi: 10.1142/S0217984916502651 [11] X. L$\ddot{u}$, S. Chen and W. Ma, Constructing lump solutions to a generalized Kadomtsev-Petviashvili-Boussinesq equation, Nonlinear Dyn., 2016, 86(1), 523-534. [12] X. Liu, X. Yao and Y. Cui, Real-time observation of the buildup of soliton molecules, Phys. Rev. Lett., 2018, 121(2), 023905. doi: 10.1103/PhysRevLett.121.023905 [13] K. Lakomy, R. Nath and L. Santos, Spontaneous crystallization and filamentation of solitons in dipolar condensates, Phys. Rev. A, 2012, 85(3), 033618. doi: 10.1103/PhysRevA.85.033618 [14] S. Lou, Soliton molecules and asymmetric solitons in three fifth order systems via velocity resonance, J. Phys. Commun., 2020, 4(4), 041002. doi: 10.1088/2399-6528/ab833e [15] S. Lou, A novel (2+1)-dimensional integrable KdV equation with peculiar solution structures, Chin. Phys. B, 2020, 29(8), 080502. doi: 10.1088/1674-1056/ab9699 [16] Y. Liu, B. Li and H. An, General high-order breathers, lumps in the (2+1)-dimensional Boussinesq equation, Nonlinear Dyn., 2018, 92(4), 2061-2076. doi: 10.1007/s11071-018-4181-6 [17] W. Ma, Interaction solutions to Hirota-Satsuma-Ito equation in (2+1)-dimensions, Front. Math. China, 2019, 14(3), 619-629. doi: 10.1007/s11464-019-0771-y [18] W. Ma, Lump and interaction solutions of linear PDEs in (3+1)-dimensions, E. Asian J. Appl. Math., 2019, 9(1), 185-194. doi: 10.4208/eajam.100218.300318 [19] W. Ma, Lump and interaction solutions to linear (4+1)-dimensional PDEs, Acta Math. Sci., 2019, 39B(2), 498-508. [20] W. Peng, S. Tian and T. Zhang, Analysis on lump, lumpoff and rogue waves with predictability to the (2+1)-dimensional B-type Kadomtsev-Petviashvili equation, Phys. Lett. A, 2018, 382, 2701-2708. doi: 10.1016/j.physleta.2018.08.002 [21] B. Ren, J. Lin and P. Liu, Soliton molecules and the CRE method in the extended mKdV equation, Commun. Theor. Phys., 2020, 72(5), 055005. doi: 10.1088/1572-9494/ab7ed6 [22] H. Shen and M. Tu, On the constrained B-type Kadomtsev-Petviashvili equation: Hirota bilinear equations and Virasoro symmetry, J. Math. Phys., 2011, 52(3), 032704. doi: 10.1063/1.3559081 [23] M. Stratmann, T. Pagel and F. Mitschke, Experimental observation of temporal soliton molecules, Phys. Rev. Lett., 2005, 95(14), 143902. doi: 10.1103/PhysRevLett.95.143902 [24] A. M. Wazwaz, Two B-type Kadomtsev-Petviashvili equations of (2+1) and (3+1) dimensions: Multiple soliton solutions, rational solutions and periodic solutions, Comput. Fluids, 2013, 86, 357-362. doi: 10.1016/j.compfluid.2013.07.028 [25] A. M. Wazwaz, N-soliton solutions for the combined KdV-CDG equation and the KdV-Lax equation, Appl. Math. Comput., 2008, 203, 402-407. [26] B. Wang, Z. Zhang and B. Li, Soliton Molecules and Some Hybrid Solutions for the Nonlinear Schrödinger Equation, Chin. Phys. Lett., 2020, 37(3), 030501. doi: 10.1088/0256-307X/37/3/030501 [27] C. Wang, H. Fang and X. Tang, State transition of lump-type waves for the (2+1)-dimensional generalized KdV equation, Nonlinear Dyn., 2019, 95(4), 2943-2961. doi: 10.1007/s11071-018-04733-5 [28] D. Xu and S. Lou, Dark soliton molecules in nonlinear optics, Acta Phys. Sin., 2020, 69(1), 014208. doi: 10.7498/aps.69.20191347 [29] X. Yang, R. Fan and B. Li, Soliton molecules and some novel interaction solutions to the (2+1)-dimensional B-type Kadomtsev-Petviashvili equation, Phys. Scr., 2020, 95(4), 045213. doi: 10.1088/1402-4896/ab6483 [30] Z. Yan and S. Lou, Soliton molecules in Sharma-Tasso-Olver-Burgers equation, Appl. Math. Lett., 2020, 104, 106271. doi: 10.1016/j.aml.2020.106271 [31] Z. Yan and S. Lou, Special types of solitons and breather molecules for a (2+1)-dimensional fifth-order KdV equation, Commun. Nonlinear Sci. Numer. Simulat., 2020, 91, 105425. doi: 10.1016/j.cnsns.2020.105425 [32] S. Yang, Z. Zhang and B. Li, Soliton molecules and some novel types of hybrid solutions to (2+1)-dimensional variable-coefficient Caudrey-Dodd-Gibbon-Kotera-Sawada equation, Adv. Math. Phys., 2020, 2020, 2670710. [33] Z. Zhang, X. Yang and B. Li, Soliton molecules and novel smooth positons for the complex modified KdV equation, App. Math. Lett., 2020, 103, 106168. doi: 10.1016/j.aml.2019.106168 [34] Q. Zhao, S. Lou and M. Jia, Solitons and soliton molecules in two nonlocal Alice-Bob Sawada-Kotera systems, Commun. Theor. Phys., 2020, 72(8), 085005. doi: 10.1088/1572-9494/ab8a0e [35] Z. Zhang, S. Yang and B. Li, Soliton molecules, asymmetric solitons and hybrid solutions for (2+1)-dimensional fifth-order KdV equation, Chin. Phys. Lett., 2019, 36(12), 120501. doi: 10.1088/0256-307X/36/12/120501 [36] J. Zhu, B. Wang, Z. Ma and J. Fei, Soliton molecules and some related interaction solutions of the (2+1)-dimensional Kadomtsev-Petviashvili hierarchy, Mod. Phys. Lett. B, 2021, 35(6), 2150106. -
-
Figures(9)
Export File
Citation
Huiling Wu, Hongyu Wu, Quanyong Zhu, Jinxi Fei, Zhengyi Ma. SOLITON, BREATHER AND LUMP MOLECULES IN THE (2+1)-DIMENSIONAL B-TYPE KADOMTSEV-PETVIASHVILI-KORTEWEG DE-VRIES EQUATION[J]. Journal of Applied Analysis & Computation, 2022, 12(1): 230-244. doi: 10.11948/20210132
Format
Content
DownLoad:
-
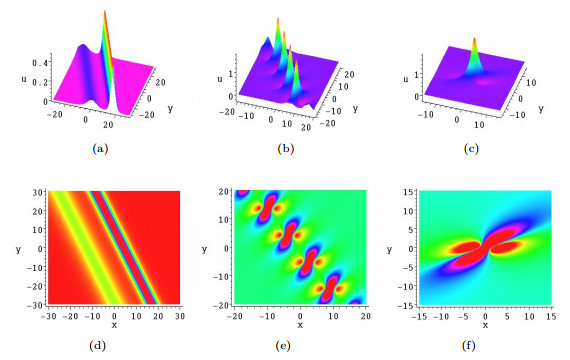
Figure 1. (a) The soliton molecule with the parameters (2.12), (2.13) and (2.14) at
$ t = 0 $ . (b) The breather with the parameters (2.16), (2.9) and (2.20) at$ t = 0 $ . (c) The lump structure given by (2.29) at$ t = 0 $ . (d)-(f) are the corresponding density figures (a)-(c), respectively. -
Figure 2. Interaction of one soliton molecule and a line soliton for the (2+1)-dimensional BKP-KdV equation (1.3) with the parameters (2.33) and (2.34) at (a)
$ t = -5 $ , (b)$ t = 0 $ and (c)$ t = 5 $ , respectively. -
Figure 3. The soliton-breather molecule for the (2+1)-dimensional BKP-KdV equation (1.3) with the parameters (2.36) and (2.37) at (a)
$ t = -2 $ , (b)$ t = 0 $ and (c)$ t = 2 $ , respectively. -
Figure 4. The soliton-lump molecule in the (2+1)-dimensional BKP-KdV equation (1.3) with the parameters (2.28) and (2.41) at (a)
$ t = -1.2 $ , (b)$ t = 0 $ and (c)$ t = 1.2 $ , respectively. -
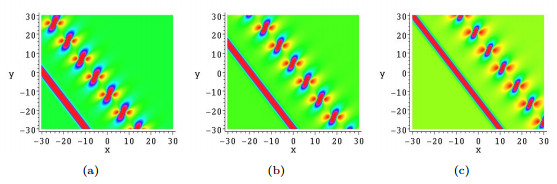
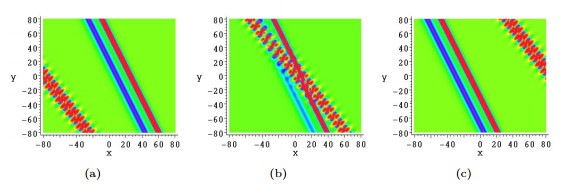
Figure 5. The elastic interaction between two soliton molecules in the (2+1)-dimensional BKP-KdV equation (1.3) with the parameters (2.43) at (a)
$ t = -2 $ , (b)$ t = 0 $ and (c)$ t = 2 $ , respectively. -
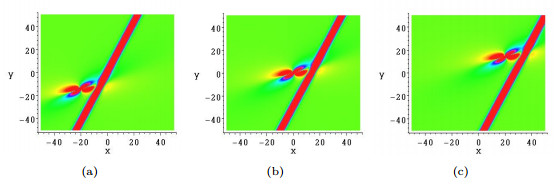
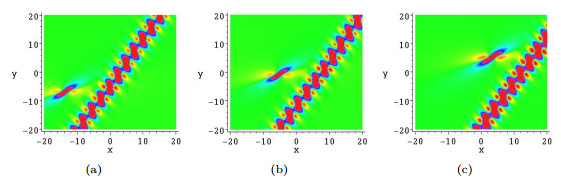
Figure 6. The molecule consisting of one soliton molecule and one breather in the (2+1)-dimensional BKP-KdV equation (1.3) with the parameter (2.44) and (2.45) at (a)
$ t = -16 $ , (b)$ t = 0 $ and (c)$ t = 16 $ , respectively. -
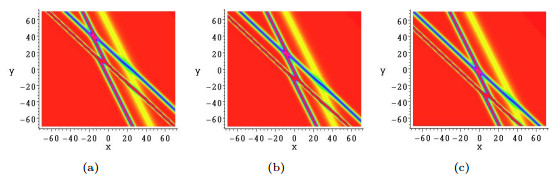
Figure 7. The elastic interaction between one soliton molecule and one breather in the (2+1)-dimensional BKP-KdV equation (1.3) with the parameter (2.44) and (2.45), but
$ \kappa = -\frac{2}{5},\;{\eta _2} = 5,\ \ \rho = \frac{{1}}{{5}},\;\varrho = \frac{{2}}{{5}} $ at (a)$ t = -16 $ , (b)$ t = 0 $ and (c)$ t = 16 $ , respectively. -
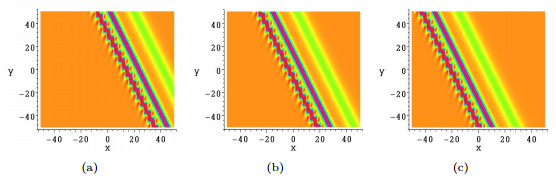
Figure 8. The molecule consisting of one breather and one lump in the (2+1)-dimensional BKP-KdV equation (1.3) with the parameter (2.46), (2.47) and (2.48) at (a)
$ t = -\frac{1}{2} $ , (b)$ t = 0 $ and (c)$ t = \frac{1}{2} $ , respectively. -
Figure 9. The elastic interaction between one breather and one lump in the (2+1)-dimensional BKP-KdV equation (1.3) with the parameters (2.20) and (2.28) at (a)
$ t = -2 $ , (b)$ t = 0 $ and (c)$ t = 2 $ , respectively.